In a diatiomic molecule, the rotational energy at a given temperature
obeys Maxwell's distribution
have the same value for all molecules
equals the translational kinetic energy for each molecule
is (2/3) rd the translational kinetic energy for each molecule
Consider a diatomic molecule as shown in the diagram. ltbr> The total energy associated with the molecule is
E=12mv2x+12mv2y+12mv2z+12Ixω2x+12Iyω2y
The above expression contains translational kinetic energy (12mv2) corresponding to velocity in each x,y and z- directions as well as rotational KE(12Iω2) associated with axis of rotations x and y.
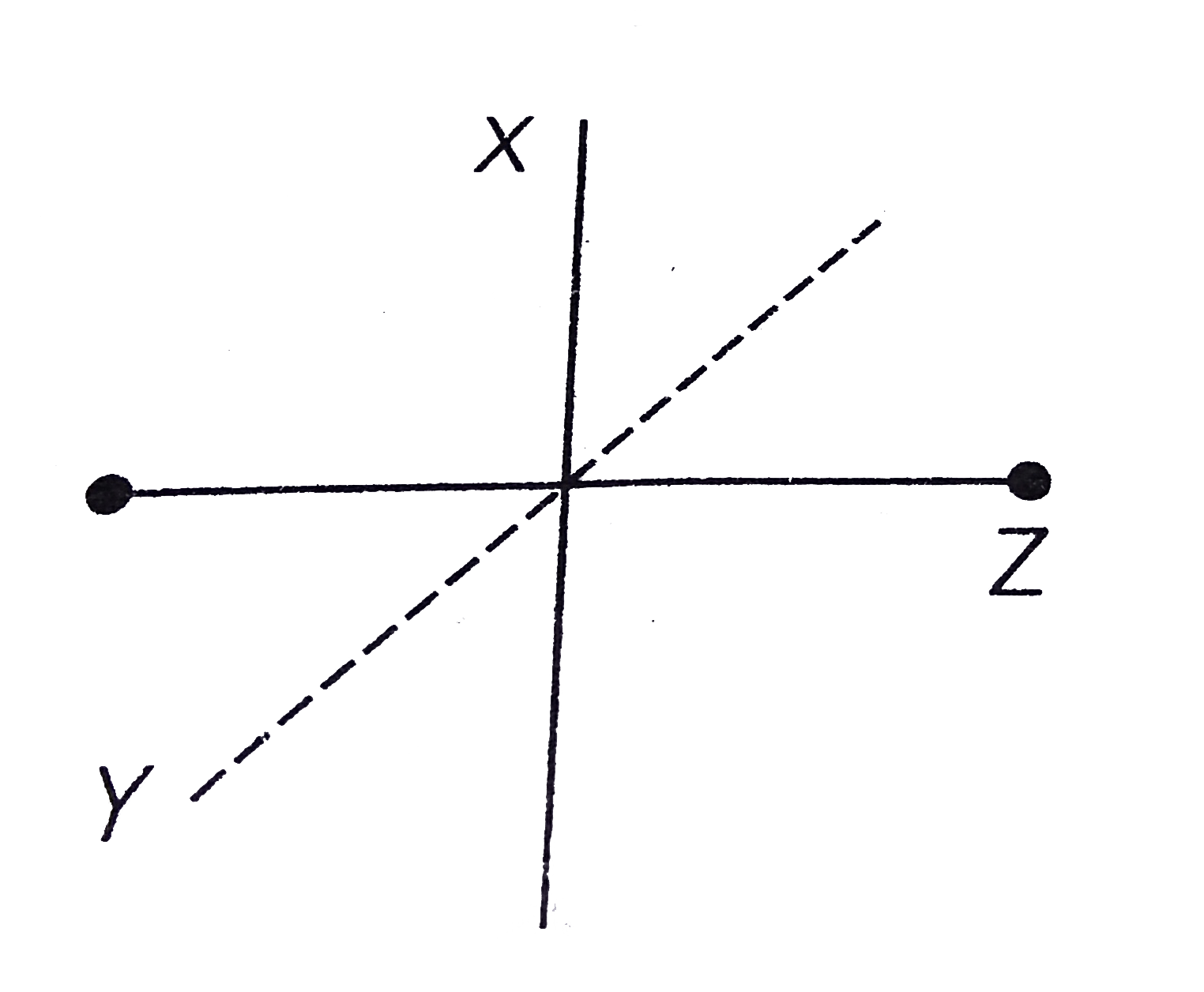
The number of independent terms in the above expression is 5.As we can predict velocities of molecules by Maxwell's distribution ,hence the above expression also obeys Maxwell's distribution. ∴2 rotational and 3 translational energies are associated with each molecule. ∴ Rotational energy at a given temperature is (23) rd of translational KE of each molecule.
Topper's Solved these Questions
Similar Questions
The heat capacities of the diatomic molecules attain a limiting value at higher temperature
At room temperature the translational and rotational degrees of freedom contribute to the heat capacities of gases while the vibrational degree of freedom becomes active only at higher temperature .
View SolutionFind the number of ratational levels per unit energy interval, dN/dE, for a diatomic molecule as a function of rotational energy E. Calculate of an magnitude for an iodine molecule in the state with rotational quantum number J=10. The distance between the nuclei of that molecule is equal to 267pm.
View Solution
Knowledge Check
Given below are two statements :
Statement I : In a diatomic molecule, the rotational energy at a given temperature obeys Maxwell's distribution.
Statement II : In a diatomic molecule, the rotational energy at a given temperature equals the translational kinetic energy for each molecule.
In the light of the above statements, choose the correct answer from the options given below :AStatement I is false but Statement II is true.BBoth Statement I and Statement II are false.CBoth Statement I and Statement II are true.DStatement I is true but Statement II is false.In a diatomic molecule, the rotational energy at given temperature
AObeys Maxwell's distributionBHave the same value for all moleculesCEquals the translational kinetic energy force moleculeDIs (2/3) rd the translational kinetic enery each moleculeAccording to the law of equipartition of energy, internal energy of an ideal gas at a given temperature, is equally distributed in translational and rotational kinetic energies.
Rotational kinetic energy of a monoatomic gas is zero.AIf both Assertion and Reason are true and the reason is correct explanation of the Assertion.BIf both Assertion and Reason are true but Reason is not the correct explanation of Assertion.CIf Assertion is true, but the Reason is false.DIf Assertion is false but the Reason is true.
The ratio of translational to rotational kinetic energies at 100K temperature is 3:2. The internal energy (in J ) of one mole gas at that temperature is (R=8.3Jmol−1K−1)
View SolutionThe average kinetic energy of a gas molecule is _______ proportional to the absolute temperature of the gas.
View SolutionWhich of the following parameters is the same for molecules of all gases at a given temperature?
View SolutionAssertion Total internal energy of oxygen gas at a given temperature is E of this energy 35 E is rotational kinetic energy.
Reason Potantial energy of an ideal gas is zero.
View SolutionSTATEMENT-1 : Fraction of total molecules having energy equal to or greater than activation energy =K/A=e−Ea/RT
and
STATEMENT-2 : Activation energy of a chemical reaction is always constant at a given temperature.
View Solution
