Three rods of equal lengths are joined to form an equilateral triangle ABC. D is the mid-point of AB. The coefficient of linear expansion is α1, for material of rod AB and α2, for material of rods of rods AC and BC. If the distance DC remains constant for small changes in temperature, then Solution in Telugu
α1=2α2
α1=4α2
α1=8α2
α1=α2
The correct Answer is:B
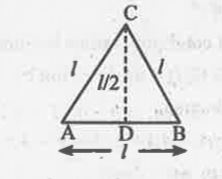
(CD)2=(AC)2−(AD)2
=l2−[12]2
After temperature variation,
(CD)2=(AC)2−(AD)2
⇒l2−(l2)2=[l(1+α2t)]2−[12l(1+α2t)]2
∴l2−l24=l2[1+α22t2+2α2t]
=l24[1+α21l2+2α1t]
α22t2 and α21t2 are neglected since they are very small
∴l2(2α2t)=l24(2α1t)
∴α1=4α2
Topper's Solved these Questions
Similar Questions
A circular loop and a square loop are formed from two wires of same length and cross section. Same current is passed through them. Then the ratio of their dipole moments is
View SolutionThe dimensions of a/b in the equation p=a−t2bx where P is pressure, x is distance ant 't' is time are,
View SolutionTwo rods having length l_1 and l_2 , made of materials with the linear expansion coefficient alpha_1 and alpha_2 , were soldered together. The equivalent coefficient of linear expansion for the obtained rod is
View SolutionAn equilateral triangle ABC if formed by two Cu rods AB and BC and one Al rod

it is heated in such a way that temperature of each rod increases by ΔT. Find change in the angle ABC. [Coeff. Of linear expansion for Cu. is α1, Coeff. of linear expansion for Al is α2]
View SolutionThree rods of equal of length are joined to from an equilateral triangle ABC. D is the midpoint of AB. The coefficient of linear expansion is α1 for AB and α2 for AC and BC . If the distance DC remains constant for small changes in temperature,

View SolutionAn equilateral triangle ABC is formed by joining three rods of equal length and D is the mid-point of AB . The coefficient of linear expansion for AB is alpha_(1) and for AC and BC is alpha_(2) . The relation between alpha_(1) and alpha_(2) , if distance DC remains constant for small changes in temperture is
View SolutionThree rods of equal length of same material are joined to form an equilateral triangle ABC as shown in figure. Area of cross-section of rod AB is S, of rod BC is 2S and that of AC is S. Then match the following.
View SolutionAs shown in the figure , an equilateral triangle ABC is formed by joining three rods of equal lengths and D is the midpoint of AB. Coefficient of linear expansion of the material of AB is alpha_1 and that of AC and BC is alpha_2 . If the length DC remains constant for small changes in temperature , then
View SolutionTwo copper rods and one aluminium rod of the same length are joined together to form an equilateral triangle ABC. A rod AD of another material connects the vertex A to the mid-point D of the base (aluminium) rod of the triangle. For small rise in temperature of the system there will be no tendency for the sides of the triangle to buckle. Determine the coefficient of linear expansion of the material of the rod AD. Given, α for copper =16×10−6∘C−1 and α for aluminium =26×10−6∘C−1.
View SolutionAmong three rods, the first two are made of same material having coefficient of linear expansion α1 and the third rod is made of material of coefficient of linear expansion α2 . The third rod forms the base of the equilateral triangle when the three rods are arranged to form an equilateral triangle. If the altitude of triangle remains constant at all temperatures, what is the ratio of α1/α2 ?
View Solutionएक समबाहु त्रिभुज ABC दो Cu की छड़ों AB व BC एवं एक Al की छड़ द्वारा बना है। उसे इस प्रकार से गर्म किया जाता है कि प्रत्येक छड़ का तापमान ΔT बढ़ जाए। कोण ABC में क्या परिवर्तन होगा?
(यहाँ, Cu का रेखीय प्रसार गुणांक α1 है, Al का रेखीय प्रसार गुणांक α2 है)
View SolutionAn equilateral triangle ABC if formed by two Cu rods AB and BC and one Al rod

it is heated in such a way that temperature of each rod increases by ΔT. Find change in the angle ABC. [Coeff. Of linear expansion for Cu. is α1, Coeff. of linear expansion for Al is α2]
View SolutionAn equilateral triangle ABC is formed by joining three rods of equal length and D is the mid point of AB. The coefficient of linear expansion for AB is α1 and for AC and BC is α2, if distance DC remains constant for small changes in temperature. Find the relation between α1andα2.
View SolutionTwo uniform metal rods of length L1andL2 and their linear coefficients of expansion α1andα2 respectively, are connected to form a single rod of length (L1+L2). When the temperature of the combined rod is raised by ''t∘C'', the length of each rod increased by the same amount then [α2α1+α2] is
View SolutionThree rods A, B and C form an equilateral triangle at 0°C. Rods AB and BC have same coefficient of expansion alpha_(1) and rod AC has alpha_2 . If change in angle between AB and BC is d theta then temperature through which system is heated.
View Solution
