A box of 1 L capacity is divided into two equal compartments by a thin partition which are filled with 2g H2 and 16 gCH4 respectively. The pressure in each compartment is reorded as P atm. The total pressure when partition is removed will be:
P
2P
P/2
P/4
The correct Answer is:a
To solve the problem step by step, we will use the ideal gas law and the concept of partial pressures.
Step 1: Determine the moles of each gas in the compartments.
1. For Hydrogen (H₂):
- Given mass = 2 g
- Molar mass of H₂ = 2 g/mol
- Moles of H₂ (n₁) = mass / molar mass = 2 g / 2 g/mol = 1 mol
2. For Methane (CH₄):
- Given mass = 16 g
- Molar mass of CH₄ = 16 g/mol
- Moles of CH₄ (n₂) = mass / molar mass = 16 g / 16 g/mol = 1 mol
Step 2: Calculate the pressure in each compartment before the partition is removed.
- The volume of each compartment = 1 L / 2 = 0.5 L
Using the ideal gas law, PV=nRT:
- For H₂:
P1⋅0.5=n1⋅R⋅T⟹P1=n1⋅R⋅T0.5=1⋅R⋅T0.5=2⋅R⋅T1
Thus, P1=2P (where P is a constant derived from R⋅T).
- For CH₄:
P2⋅0.5=n2⋅R⋅T⟹P2=n2⋅R⋅T0.5=1⋅R⋅T0.5=2⋅R⋅T1
Thus, P2=2P.
Step 3: Calculate the total pressure after the partition is removed.
- When the partition is removed, the total volume becomes 1 L, and the total number of moles of gas is:
ntotal=n1+n2=1+1=2 moles
Using the ideal gas law again for the total pressure Ptotal:
Ptotal⋅1=ntotal⋅R⋅T⟹Ptotal=ntotal⋅R⋅T=2⋅R⋅T
Step 4: Relate the total pressure back to the individual pressures.
Since we previously established that P1=2P and P2=2P, the total pressure can also be expressed as:
Ptotal=P1+P2=2P+2P=4P
Conclusion:
Thus, the total pressure when the partition is removed is:
Ptotal=4P
Final Answer:
The total pressure when the partition is removed will be 4P atm.
---
1. For Hydrogen (H₂):
- Given mass = 2 g
- Molar mass of H₂ = 2 g/mol
- Moles of H₂ (n₁) = mass / molar mass = 2 g / 2 g/mol = 1 mol
- Given mass = 16 g
- Molar mass of CH₄ = 16 g/mol
- Moles of CH₄ (n₂) = mass / molar mass = 16 g / 16 g/mol = 1 mol
- The volume of each compartment = 1 L / 2 = 0.5 L
- For H₂:
P1⋅0.5=n1⋅R⋅T⟹P1=n1⋅R⋅T0.5=1⋅R⋅T0.5=2⋅R⋅T1
Thus, P1=2P (where P is a constant derived from R⋅T).
P2⋅0.5=n2⋅R⋅T⟹P2=n2⋅R⋅T0.5=1⋅R⋅T0.5=2⋅R⋅T1
Thus, P2=2P.
- When the partition is removed, the total volume becomes 1 L, and the total number of moles of gas is:
ntotal=n1+n2=1+1=2 moles
Ptotal⋅1=ntotal⋅R⋅T⟹Ptotal=ntotal⋅R⋅T=2⋅R⋅T
Since we previously established that P1=2P and P2=2P, the total pressure can also be expressed as:
Ptotal=P1+P2=2P+2P=4P
Thus, the total pressure when the partition is removed is:
Ptotal=4P
The total pressure when the partition is removed will be 4P atm.
Topper's Solved these Questions
- GASEOUS STATEBOOK - NARENDRA AWASTHICHAPTER - GASEOUS STATEEXERCISE - Level 1 (Q.31 To Q.60)
1 Videos
- GASEOUS STATEBOOK - NARENDRA AWASTHICHAPTER - GASEOUS STATEEXERCISE - Level 1 (Q.121 To Q.150)
1 Videos
- ELECTROCHEMISTRYBOOK - NARENDRA AWASTHICHAPTER - ELECTROCHEMISTRYEXERCISE - Level 3 - Subjective Problems
1 Videos
- IONIC EEQUILIBRIUMBOOK - NARENDRA AWASTHICHAPTER - IONIC EEQUILIBRIUMEXERCISE - Exercise
196 Videos
Similar Questions
A box is divided by a thin partition into equal compartments and they are filled with an equal number of hydrogen and heavy hydrogen molecules respectively. If the pressure in the hydrogen compartment is 1 cm Hg, what is the pressure in the other compartment ? What will be the pressure if the partition is removed ?
View SolutionA container is divided into two compartments by a removable partition as shown below :
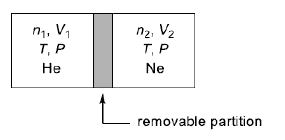
In the first compartment n1 moles of ideal gas He is present in a volume V1 . In the second compartment, n2 moles of ideal gas Ne is present in a volume V2. The temperature and pressure in both the compartments are T and P repectively. Assuming R is the gas constant. the total change is entropy upon removing the partition when the gases mix irreversibly is :
View Solution
Knowledge Check
A box is divided into two equal compartments by a thin partition and they are filled with gases X and Y respectively. The two compartments have a pressure of 250 torr each. The pressure after removing the partition will be equal to:
A125 torrB500 torrC250 torrD750 torrA vessel has two equal compartments A and B containing H2 and O2 respectively, each at 1 atm pressure. If the wall separating the compartment is removed, the pressure
Awill remain unchanged in A and BBwill increase in A and decrease in BCwill decrease in A and increase in BDwill increase in both A and B.A container of volume 1m3 is divided into two equal compartments by a partition. One of these compartments contains an ideal gas at 300 K . The other compartment is vacuum. The whole system is thermally isolated from its surroundings. The partition is removed and the gas expands to occupy the whole volume of the container. Its temperature now would be
A300 kB239 kC200 kD100 k
A sound wave passing volume 1m3 is divided into two equal compartments by a partition. One of these compartments contains an ideal gas at 300K. The other compartment is vacuum. The whole system is thermally isolated from its surroundings. The partition is removed and the gas expands to occupy the whole volume of the container. Its temperature now would be
View SolutionA container of volume 1m3 is divided into two equal compartments, one of which contains an ideal gas at 300K. The oterh compartment is vaccum. The whole system is thermally isolated from its surroundings. The partition is removed and the gas expands to occupy the whole volume of the container. Its temperature now would be
View SolutionA container of volume 2m3 is divided into two equal compartments, one of which contains an ideal gas at 400 K. The other compartment is vacuum. The whole system is thermally isolated from its surroundings. The partition is removed and the surrounding. The partition is removed and the gas expands to occupy the whole volume of the container.Its temperature now would be
View SolutionA tank with a square base of area 2m2 is divided into two compartments by a vertical partition in the middle. There is a small hinged door of face area 20cm2 at the bottom of the partition. Water is filled in one compartment and an acid of relative density 1.5 in the other, both to a height of 4m. The force necessary to keep the door closed is (Takeg=10ms−2)
View SolutionGaseous mixture of contains 56 g of N2 , 44g of CO2 and 16 g of CH4 . The total pressure of mixture is 720 mm of Hg . The partial pressure of CH4 is :-
View Solution
