A container of volume 1m3 is divided into two equal compartments, one of which contains an ideal gas at 300K. The oterh compartment is vaccum. The whole system is thermally isolated from its surroundings. The partition is removed and the gas expands to occupy the whole volume of the container. Its temperature now would be
300K
250K
200K
100K
The correct Answer is:A
To solve the problem step by step, let's analyze the situation:
Step 1: Understand the System
We have a container of volume 1m3 divided into two equal compartments. One compartment contains an ideal gas at a temperature of 300K and the other compartment is a vacuum.
Step 2: Remove the Partition
When the partition is removed, the gas expands to fill the entire volume of the container. Since one side is a vacuum, the gas will undergo free expansion.
Step 3: Analyze the Thermodynamic Process
The system is thermally isolated, which means there is no heat exchange with the surroundings. According to the first law of thermodynamics:
ΔU=Q−W
Where:
- ΔU is the change in internal energy,
- Q is the heat added to the system,
- W is the work done by the system.
Step 4: Determine Heat Transfer and Work Done
Since the system is thermally isolated:
Q=0
Also, during free expansion into a vacuum, the work done W is zero:
W=0
Step 5: Calculate Change in Internal Energy
Substituting these values into the first law:
ΔU=0−0=0
This means the change in internal energy is zero.
Step 6: Relate Change in Internal Energy to Temperature
For an ideal gas, the change in internal energy is given by:
ΔU=nCvΔT
Where:
- n is the number of moles,
- Cv is the specific heat at constant volume,
- ΔT is the change in temperature.
Since ΔU=0, we have:
nCvΔT=0
This implies that either n=0, Cv=0, or ΔT=0. Since the number of moles and specific heat cannot be zero for an ideal gas, we conclude:
ΔT=0
Step 7: Final Temperature Calculation
Since ΔT=Tfinal−Tinitial=0, we have:
Tfinal=Tinitial
Given that the initial temperature Tinitial=300K, we find:
Tfinal=300K
Conclusion
The final temperature of the gas after it expands into the vacuum is 300K.
---
We have a container of volume 1m3 divided into two equal compartments. One compartment contains an ideal gas at a temperature of 300K and the other compartment is a vacuum.
When the partition is removed, the gas expands to fill the entire volume of the container. Since one side is a vacuum, the gas will undergo free expansion.
The system is thermally isolated, which means there is no heat exchange with the surroundings. According to the first law of thermodynamics:
ΔU=Q−W
Where:
- ΔU is the change in internal energy,
- Q is the heat added to the system,
- W is the work done by the system.
Since the system is thermally isolated:
Q=0
Also, during free expansion into a vacuum, the work done W is zero:
W=0
Substituting these values into the first law:
ΔU=0−0=0
This means the change in internal energy is zero.
For an ideal gas, the change in internal energy is given by:
ΔU=nCvΔT
Where:
- n is the number of moles,
- Cv is the specific heat at constant volume,
- ΔT is the change in temperature.
nCvΔT=0
This implies that either n=0, Cv=0, or ΔT=0. Since the number of moles and specific heat cannot be zero for an ideal gas, we conclude:
ΔT=0
Since ΔT=Tfinal−Tinitial=0, we have:
Tfinal=Tinitial
Given that the initial temperature Tinitial=300K, we find:
Tfinal=300K
The final temperature of the gas after it expands into the vacuum is 300K.
Topper's Solved these Questions
- KINETIC THEORY OF GASESBOOK - NARAYNACHAPTER - KINETIC THEORY OF GASESEXERCISE - LEVEL-II(C.W)
25 Videos
- KINETIC THEORY OF GASESBOOK - NARAYNACHAPTER - KINETIC THEORY OF GASESEXERCISE - LEVEL-III(C.W)
52 Videos
- KINETIC THEORY OF GASESBOOK - NARAYNACHAPTER - KINETIC THEORY OF GASESEXERCISE - C.U.Q
153 Videos
- GRAVITATIONBOOK - NARAYNACHAPTER - GRAVITATIONEXERCISE - EXERCISE -IV
40 Videos
- LAW OF MOTIONBOOK - NARAYNACHAPTER - LAW OF MOTIONEXERCISE - EXERCISE - IV
35 Videos
Similar Questions
A container of volume 1m3 is divided into two equal parts by a partition. One part has an ideal gas at 300K and the other part is vacuum. The whole system is thermally isolated from the surroundings. When the partition is removed, the gas expands to occupy the whole volume. Its temperature will now be .......
View SolutionA weightless piston divides a thermally insulated cylinder into two equal parts. One part contains one mole of an ideal gas with adiabatic exponent γ, the other is evacuated. The initial gas temperature is T0. The piston is released and the gas fills the whole volume of the cylinder. Then the piston is slowly displaced back to the initial position. Find the increment of the internal energy and entropy of the gas was resulting from these two processes.
View Solution
Knowledge Check
A container of volume 1m3 is divided into two equal compartments by a partition. One of these compartments contains an ideal gas at 300 K . The other compartment is vacuum. The whole system is thermally isolated from its surroundings. The partition is removed and the gas expands to occupy the whole volume of the container. Its temperature now would be
A300 kB239 kC200 kD100 kA sound wave passing volume 1m3 is divided into two equal compartments by a partition. One of these compartments contains an ideal gas at 300K. The other compartment is vacuum. The whole system is thermally isolated from its surroundings. The partition is removed and the gas expands to occupy the whole volume of the container. Its temperature now would be
A8.97×10−4KB8.97×10−6KC8.97×10−8KDnone of theseA container of volume 2m3 is divided into two equal compartments, one of which contains an ideal gas at 400 K. The other compartment is vacuum. The whole system is thermally isolated from its surroundings. The partition is removed and the surrounding. The partition is removed and the gas expands to occupy the whole volume of the container.Its temperature now would be
A400KB250KC200KD100K
A container of volume 1m3 is divided into two equal parts by a partition. One part has an ideal gas at 300K and the other part is vacuum. The whole system is thermally isolated from the surroundings. When the partition is removed, the gas expands to occupy the whole volume. Its temperature will now be .......
View SolutionA rigid and insulated tank of 3m3 volume is divided into two compartments. One compartment of volume of 2m3 contains an ideal gas at 0.8314 Mpa and 400 K while the second compartment of volume of 1m3 contains the same gas at 8.314 Mpa and 500 K. If the partition between the two compartments is rptured, the final temperature of the gas is :
View SolutionA rigid and insulated tank of 3m3 volume is divided into two compartments. One compartment of volume of 2m3 contains an ideal gas at 0.8314 Mpa and 400 K while the second compartment of volume of 1m3 contains the same gas at 8.314 Mpa and 500 K. If the partition between the two compartments is rptured, the final temperature of the gas is :
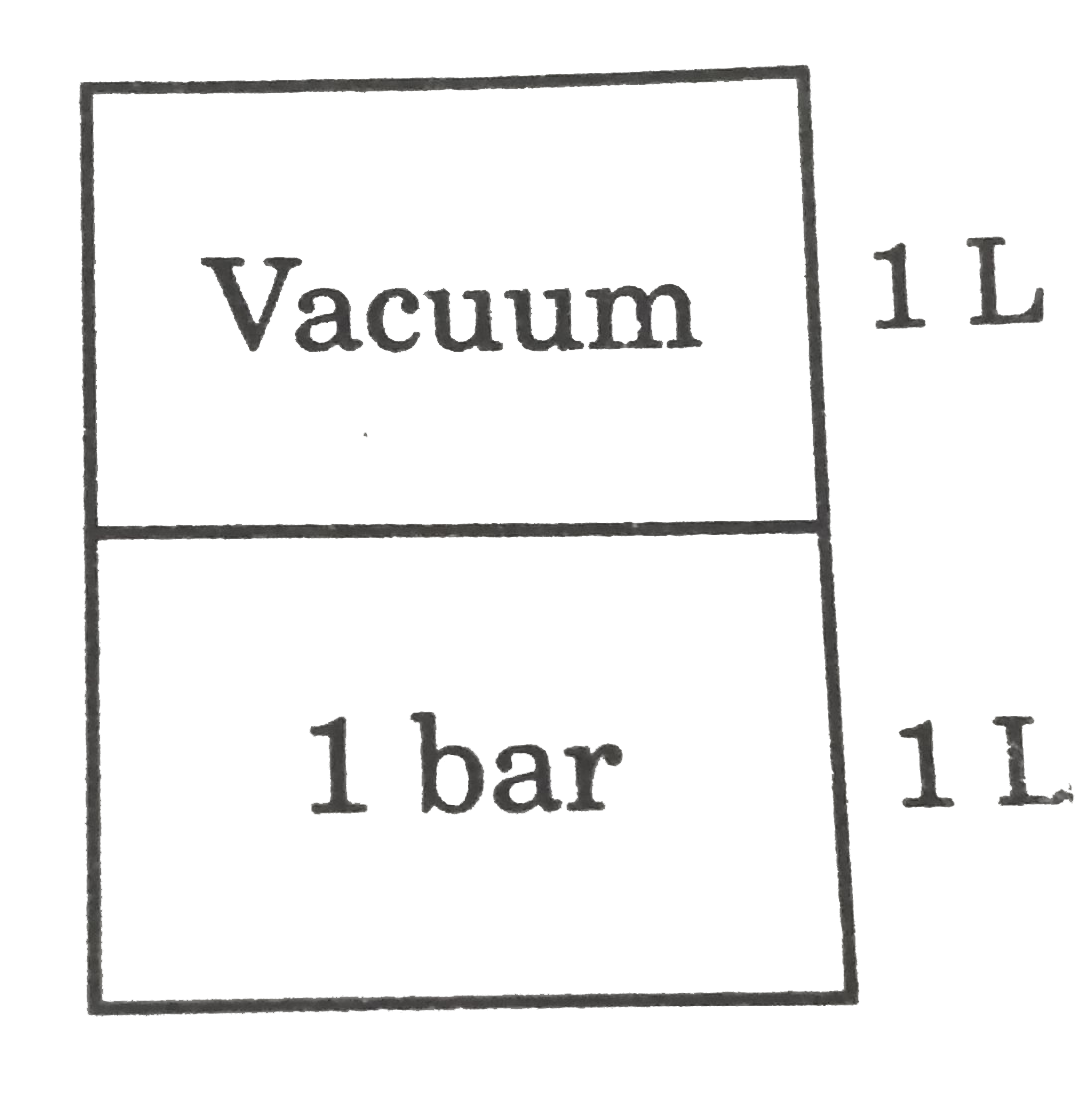
View SolutionA container of volume 2L is seperated into equal compartments. In one compartment, one mole of an ideal monoatomic gas is filled at 1 bar pressure and the other compartment is completely evacuted. A pihole is made in the seperator so gas expands to occupy full 2 L and heat is supplied to gas so that finally pressure of gas equals 1 bar. Then :
View SolutionAn ideal monoatomic gas at 300K expands adiabatically to twice its volume. What is the final temperature?
View Solution
