A dancer is standing on a rotating platform taking two sphere on her hands. If she drops down the sphere on ground, then dancer's:
angular velocity will increase
angular momentum & angular velocity both will unchange
angular momentum unchanged & angular velocity will increase
both will decrease
The correct Answer is:3
To solve the problem step-by-step, we will analyze the situation of the dancer on the rotating platform holding two spheres and what happens when she drops them.
1. Understand the System:
- The dancer is standing on a rotating platform and is holding two spheres.
- The initial angular velocity of the system (dancer + spheres) is denoted as ωi.
2. Identify the Moment of Inertia:
- The moment of inertia of the dancer alone is I0.
- The moment of inertia contributed by the two spheres can be expressed as mr21+mr22, where m is the mass of each sphere and r1 and r2 are their respective distances from the axis of rotation.
- Therefore, the total initial moment of inertia Ii is:
Ii=I0+mr21+mr22
3. Conservation of Angular Momentum:
- Since there are no external torques acting on the system, angular momentum is conserved.
- The initial angular momentum Li can be expressed as:
Li=Ii⋅ωi
- After the dancer drops the spheres, the final moment of inertia If will be just the moment of inertia of the dancer:
If=I0
- The final angular momentum Lf is:
Lf=If⋅ωf
4. Setting Up the Equation:
- By conservation of angular momentum, we have:
Li=Lf
- Substituting the expressions for angular momentum gives:
Ii⋅ωi=I0⋅ωf
5. Solving for Final Angular Velocity:
- Rearranging the equation to solve for ωf:
ωf=IiI0⋅ωi
- Substituting Ii:
ωf=I0+mr21+mr22I0⋅ωi
- This can be simplified to:
ωf=(1+mr21+mr22I0)⋅ωi
6. Analyzing the Result:
- Since mr21+mr22 is positive, the term mr21+mr22I0 is also positive.
- Therefore, ωf>ωi, indicating that the angular velocity of the dancer increases after dropping the spheres.
7. Conclusion:
- The angular momentum of the dancer remains unchanged (conserved), while the angular velocity increases.
Final Answer:
- The dancer's angular velocity increases, while her angular momentum remains unchanged.
- The dancer is standing on a rotating platform and is holding two spheres.
- The initial angular velocity of the system (dancer + spheres) is denoted as ωi.
- The moment of inertia of the dancer alone is I0.
- The moment of inertia contributed by the two spheres can be expressed as mr21+mr22, where m is the mass of each sphere and r1 and r2 are their respective distances from the axis of rotation.
- Therefore, the total initial moment of inertia Ii is:
Ii=I0+mr21+mr22
- Since there are no external torques acting on the system, angular momentum is conserved.
- The initial angular momentum Li can be expressed as:
Li=Ii⋅ωi
- After the dancer drops the spheres, the final moment of inertia If will be just the moment of inertia of the dancer:
If=I0
- The final angular momentum Lf is:
Lf=If⋅ωf
- By conservation of angular momentum, we have:
Li=Lf
- Substituting the expressions for angular momentum gives:
Ii⋅ωi=I0⋅ωf
- Rearranging the equation to solve for ωf:
ωf=IiI0⋅ωi
- Substituting Ii:
ωf=I0+mr21+mr22I0⋅ωi
- This can be simplified to:
ωf=(1+mr21+mr22I0)⋅ωi
- Since mr21+mr22 is positive, the term mr21+mr22I0 is also positive.
- Therefore, ωf>ωi, indicating that the angular velocity of the dancer increases after dropping the spheres.
- The angular momentum of the dancer remains unchanged (conserved), while the angular velocity increases.
- The dancer's angular velocity increases, while her angular momentum remains unchanged.
Similar Questions
A dancer is standing on a stool rotating about the vertical axis passing through its centre. She pulls her arms towards the body reducing her moment of inertia by a factor of n. The new angular speed of turn table is proportional to
View SolutionA dancer is rotating on smooth horizontal floor with an angular momentum L The dancer folds her hands so that her moment of inertia decreases by 25%. The new angular momentum is
View Solution
Knowledge Check
A person is standing on a rotating table with metal spheres in his hands. If he withdraws his hands to his chest, then the effect on his angular velocity will be.
AincreaseBdecreaseCremain sameDcan't sayA dancer is standing on a stool rotating about the vertical axis passing through its centre. She pulls her arms towards the body reducing her moment of inertia by factor of n. The new angular speed of turn table is proportional to :
AnBn−1Cn∘Dn2A dancer is standing on a stool rotating about the vertical axis passing through its centre. She pulls her arms towards the body reducing her moment of inertia by factor of n. The new angular speed of turn table is proportional to :
AnBn−1Cn∘Dn2
A man stands holding a weight in each hand and with his arms outstretched on a frictionless platform which is rotating at a speed of 1 revolution per sec. In ths position the total rotational intertia of the man, the weights and the platform is 6kgm2. If by drawing in his arms, the man decreases the rotational inertia of the system by 2kgm2, calcualte the resulting speed of the platform and the increase in kinetic energy. How do you account for the increase of kinetic energy?
View SolutionA hollow sphere of radius r is rotating about a horizontal axis at some angular speed ω0. It is gently lowered to ground and the coefficient of friction between sphere and the ground is μ.How far does the sphere move before it starts pure rolling?
View SolutionWater from a tap, maintained at a costant potential V, is allolwed to fall by drops of radius r through a small hole into a hollow conducting sphere of radius R standing on an insulating stand until it fills the entire sphere. Find the potential of the hollow conductor after it is completely filled with water.
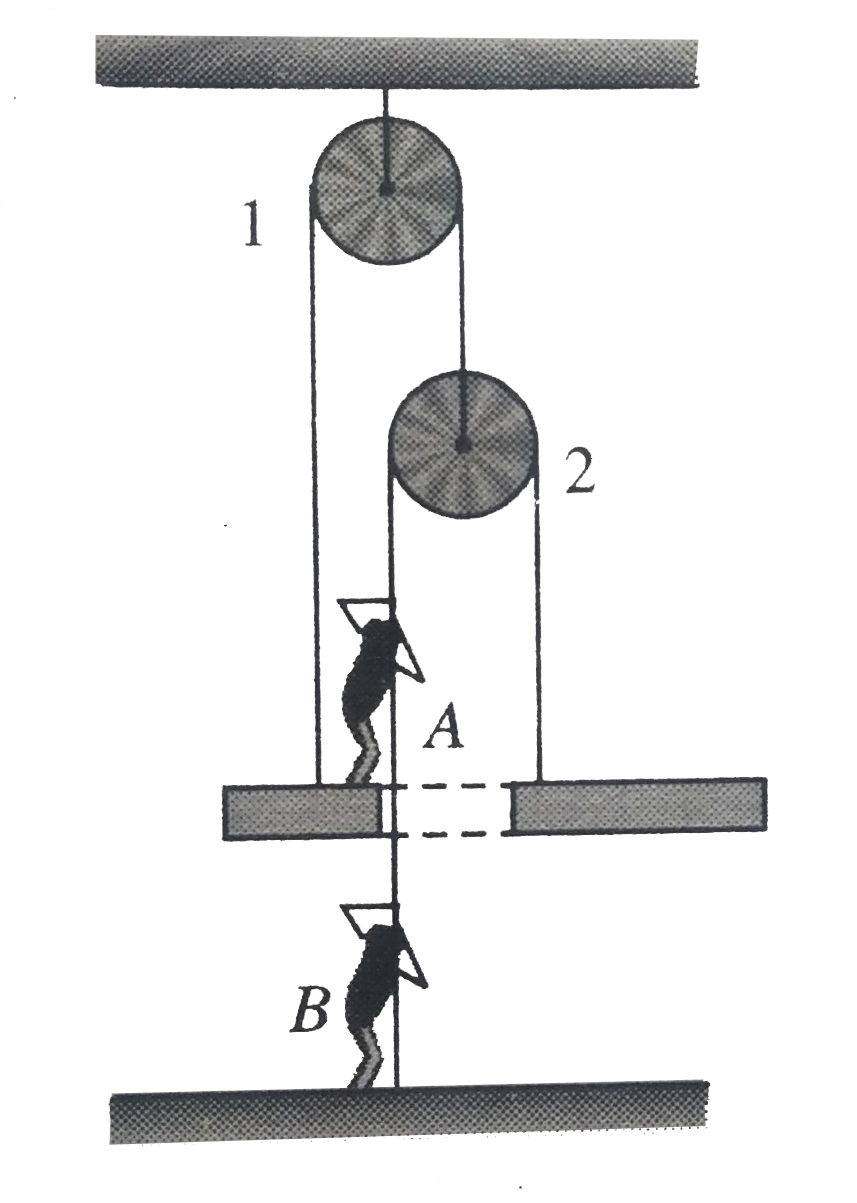
View SolutionIn fig. man A is standing on a movable plank while man B is standing on a stationary platform. Both are pulling the string down such that the plank moves slowly up. As a result of this the string slops through the hands of the men, find the ratio of length of the string that slips through the hands of A and B.
View SolutionA child is standing wiith hands at the centre of a platform rotating about its central axis. The kinetie energy of the system K. The chilid now seretches his arms so that of the kinetic energy of the system is now.
View Solution
