A swimmer wishes to reach directly opposite bank of a river, flowing with velocity 8 m/s. The swimmer can swim 10 m/s still water. The width of the river is 480 m. Time taken by him to do so:
60 sec
48 sec
80 sec
None of these.
The correct Answer is:C
To solve the problem of the swimmer trying to reach the opposite bank of a river flowing with a velocity of 8 m/s, while he can swim at a speed of 10 m/s in still water, we can follow these steps:
1. Identify the Given Data:
- Velocity of the river, Vr=8m/s
- Velocity of the swimmer in still water, Vs=10m/s
- Width of the river, d=480m
2. Determine the Angle of Swimming:
- To reach directly opposite the bank, the swimmer must swim at an angle θ such that the resultant velocity has no x-component (i.e., it should be directed straight across the river).
- The x-component of the swimmer's velocity must balance the river's velocity:
Vr=Vscosθ
- This gives us:
8=10cosθ
- Rearranging gives:
cosθ=810=0.8
- Therefore, θ=cos−1(0.8).
3. Calculate the y-component of the Swimmer's Velocity:
- The y-component of the swimmer's velocity is given by:
Vsy=Vssinθ
- Using the identity sin2θ+cos2θ=1:
sinθ=√1−cos2θ=√1−0.82=√1−0.64=√0.36=0.6
- Thus:
Vsy=10sinθ=10×0.6=6m/s
4. Calculate the Time Taken to Cross the River:
- The time taken to cross the river can be calculated using the formula:
Time=DistanceVelocity=dVsy=480m6m/s=80s
Final Answer:
The time taken by the swimmer to reach the opposite bank is 80 seconds.
---
- Velocity of the river, Vr=8m/s
- Velocity of the swimmer in still water, Vs=10m/s
- Width of the river, d=480m
- To reach directly opposite the bank, the swimmer must swim at an angle θ such that the resultant velocity has no x-component (i.e., it should be directed straight across the river).
- The x-component of the swimmer's velocity must balance the river's velocity:
Vr=Vscosθ
- This gives us:
8=10cosθ
- Rearranging gives:
cosθ=810=0.8
- Therefore, θ=cos−1(0.8).
- The y-component of the swimmer's velocity is given by:
Vsy=Vssinθ
- Using the identity sin2θ+cos2θ=1:
sinθ=√1−cos2θ=√1−0.82=√1−0.64=√0.36=0.6
- Thus:
Vsy=10sinθ=10×0.6=6m/s
- The time taken to cross the river can be calculated using the formula:
Time=DistanceVelocity=dVsy=480m6m/s=80s
The time taken by the swimmer to reach the opposite bank is 80 seconds.
Similar Questions
A swimmer wishes to reach directly opposite point on the other bank of a river,flowing with velocity 4√2ms.The swimmer can swim with 8ms in still water.The angle with the flow direction he should swim to reach the directly opposite point is (A)450 (B)900 (C)1350 (D)1500
View SolutionA swimmer can swim in still water with a speed of 2.5 m/s. What is the shortest time taken by him to swim across the river?

View Solution
Knowledge Check
A person reaches on a point directly opposite on the other bank of a river.The velocity of the water in the river is 4m/sand the velocity of the person in still water is 5m/s.If the width of the river is 84.6m, time taken to cross the river in seconds is
A9.4B2C84.6D28.2A man wishes to cross a river flowing with velocity u swims at an angle θ with the river flow.If the man swims with speed vand if the width of the river is d, then the drift travelled by him is
A(u+vcosθ)dvsinθB(u−vcosθ)dvsinθC(u−vcosθ)dvcosθD(u+vcosθ)dvcosθA swimmer wishes to cross a 500−m river flowing at 5kmh−1. His speed with respect to water is 3kmh−1. The shortest possible time to cross the river is.
A10minB20minC6minD7.5min
A swimmer starts to swim from point a to cross a river. He wants to reach point B on the opposite side of the river. The line AB makes an angle 60∘ with the river flow as hown. The velocity of the swimmer in still water is same as that of the water

(i) In what direction should he try to direct his velocity ? Calculate angle between his velocity anf river velocity.
(ii) Find the ratio of the time taken to cross the river in this situation to the minimum time in which he can cross this river.
View SolutionA man can swim in still water at a speed of 6kmph and he has to cross the river and reach just opposite point on the other bank.If the river is flowing at a speed of 3kmph,and the width of the river is 2km, the time taken to cross the river is (in hours)
View SolutionA man wants to reach point B on the opposite bank of a river flowing at a speed 4m/s as shown in the figure. What minimum speed relative to water should the man have so that he can reach point B directly by swimming?
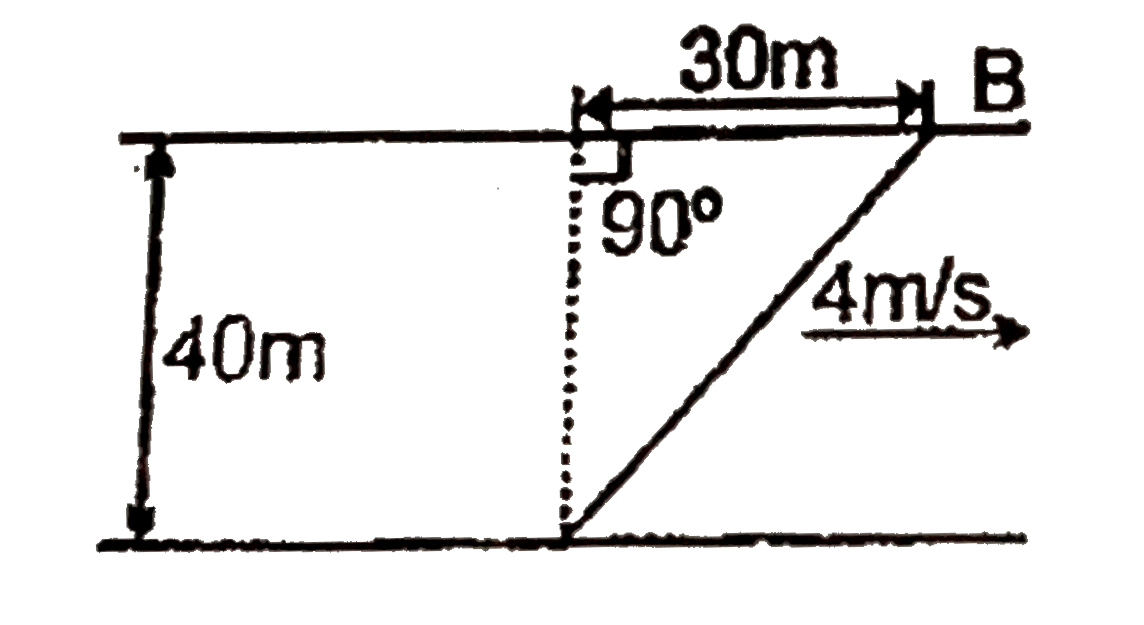
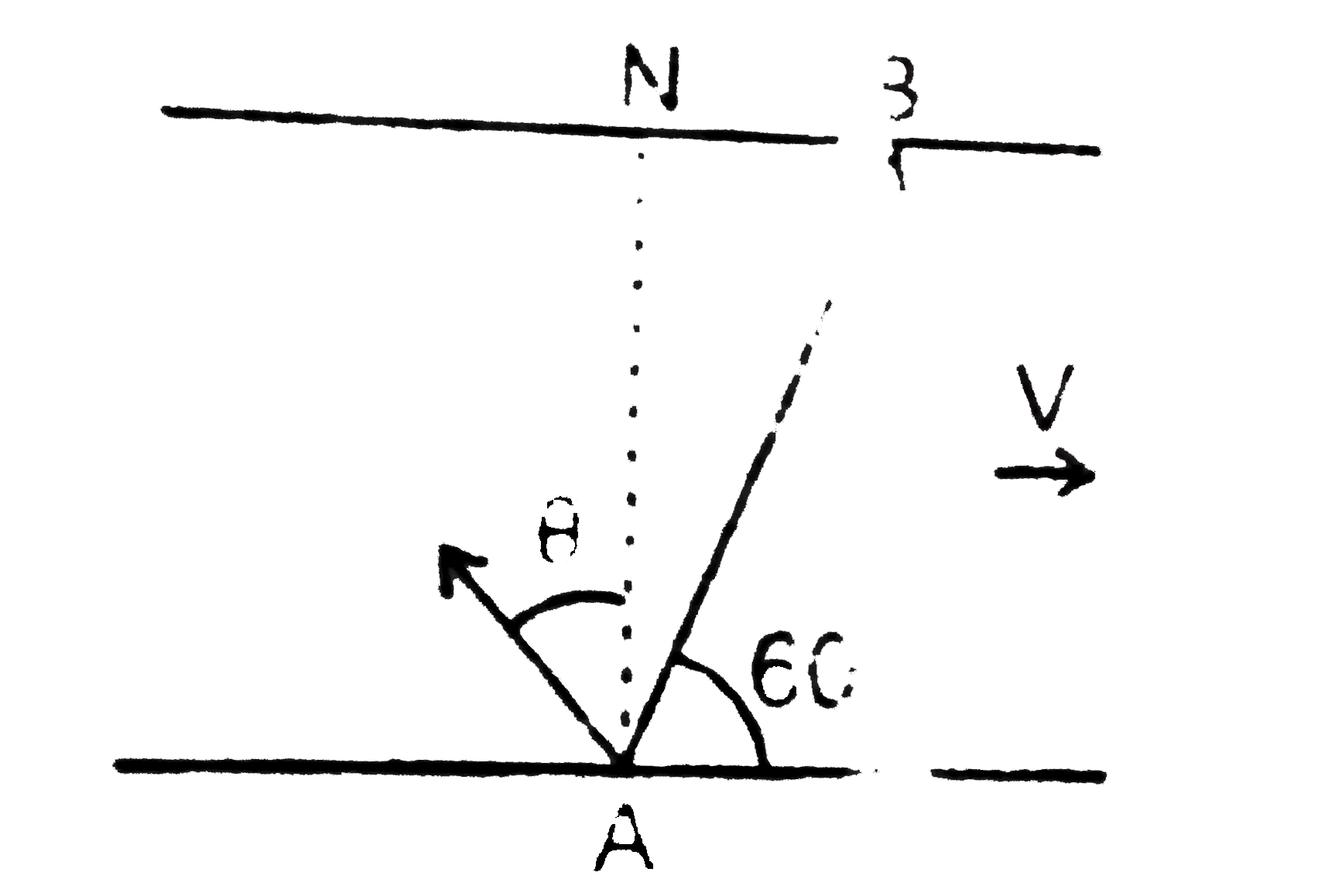
View SolutionA swimmer wands to cross a river and reach point B directly from A. The speed of the wimmer in still river and that of river flow are same. The dotted line AN is normal to flow direction of river. For swimmer to reach point B, the angle his velocity relative to river will make with line AN is given by θ. then the value of θ is :
View SolutionA person, reaches a point directly opposite on the other bank of a flowing river, while swimming at a speed of 5 m/s at an angle of 120∘ with the flow. The speed of the flow must be
View Solution
