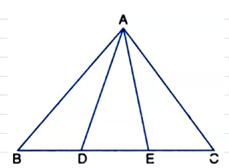
In Figure, D,E are points on sides AB and AC respectively of ABC, such that ar(BCE)=ar(BCD). Show that DEBC.
Given D and E are point on sides AB and AC respectively of triangle ABC such that Area(ΔDBC) = Area(ΔEBC).
Given that Area(ΔDBC)=Area(ΔEBC).
Then the height of triangle DBC and Triangle EBC are the same because both triangles are the same
Then it possible that both triangle on the same base and between parallel line BC and DE
Therefore, they will lie between the same parallel lines.
Then, BC∥DE [henceproved]
Topper's Solved these Questions
Similar Questions
D and E are points on sides AB and AC respectively of ΔABCsuch thatar (DBC) = ar (EBC). Prove that DE ∣∣ BC.
View SolutionIn a parallelogram ABCD,E,F are any two points on the sides AB and BC respectively. Show that ar(ADF)=ar(DCE).
View Solutionp AND q are any two points lying on the sides DC and AD respectively of a parallelogram ABCD. Show that ar(APB)=ar (BQC).
View SolutionA point D is taken on the side BC of a ABC such that BD=2dC. Prove that ar(ABD)=2ar(ADC).
View SolutionTriangles ABC and +DBC are on the same base BC with A, D on opposite side of line BC, such that ar(△ABC)=ar(△DBC). Show that BC bisects AD.
View SolutionIn a ABC , D and E are points on the sides AB and AC respectively such that DEBC If AD=6 cm , DB=9 cm and AE=8 cm , find AC . (ii) If ADDB=34 and AC=15 cm , find AE . (iii) If ADDB=23 and AC=18 cm , find AE .
View SolutionIn a ABC, D and E are points on the sides AB and AC respectively. For each of the following cases show that DEBC : AB=12 cm, AD=8 cm, AE=12 cm and AC=18 cm . (ii) AB=5.6 cm, AD=1.4 cm, AC=7.2 cm and AE=1.8 cm
View SolutionIn Figure DandE are two points on BC such that BD=DE=EC Show that ar(ABD)=ar(ADE)=ar(AEC)
View SolutionIn a ABC , D and E are points on the sides AB and AC respectively such that DEBC If AD=4 , AE=8 , DB=x−4 , and EC=3x−19 , find x (ii) If AD=8 cm , AB=12cm and AE=12 cm , find CE . (iii) If AD=4 cm , DB=4.5 cm and AE=8 cm , find AC .
View SolutionIn a ABC , D and E are points on the sides AB and AC respectively such that DEBC If AD=2 cm , AB=6 cm and AC=9 cm , find AE . (ii) If ADBD=45 and EC=2.5 cm , find AE . (iii) If AD=x , DB=x−2 , AE=x+2 and EC=x−1 , find the value of x .
View Solution