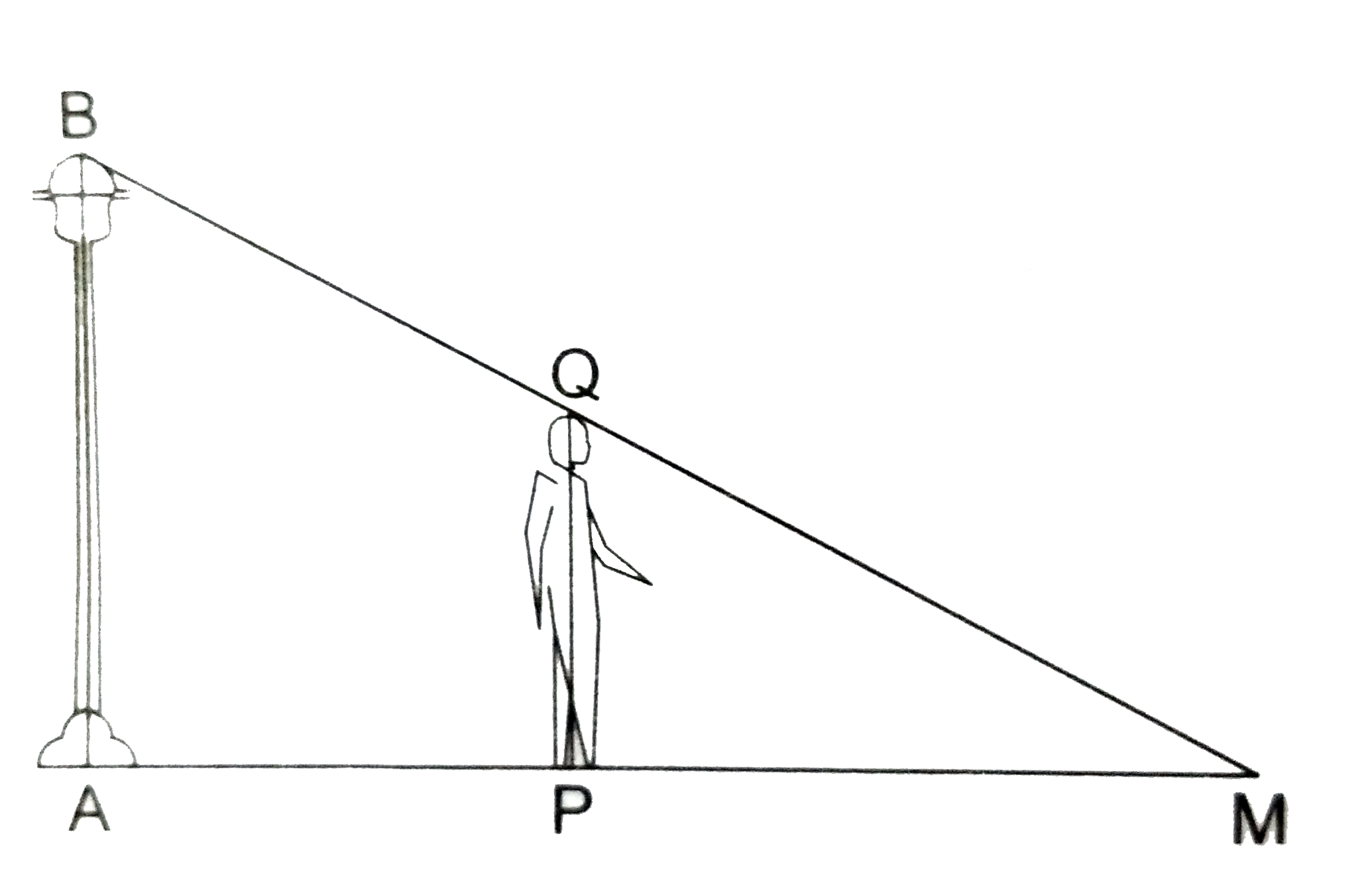
A girl of height 90 cm is walking away from the base of a lamp-post at a speed of 1.2 m/s. If the lamp is 3.6 m above the ground, find the length of her shadow after 4 seconds.
Let the girl of height be at point D on the ground from the lamp post after 4 seconds. Therefore,
AD=1.2 m/sec ×4 sec =4.8 m =480 cm
Suppose the length of the shadow of the girl be x cm when she at position D. Therefore,
BD=x cm
In ∆BDE and ∆BAC,
∠BDE=∠BAC (90°)
∠DBE=∠ABC (Common)
Thus, ∆BDE∼∆BAC (AA similarity)
BEBC=DEAC=BDAB
( Corresponding sides are proportional )
90360=x480+x
⇒4x=480+x
⇒4x−x=480
⇒3x=480
⇒x=160
Hence length of her shadow after 4 seconds is 160 cm.
Topper's Solved these Questions
Similar Questions
A girl of height 90 cm is walking away from the base of a lamp-post at a speed of 1.2 m/s. If the lamp is 3.6 m above the ground, find the length of her shadow after 4 seconds.
View SolutionA girl of height 100 cm is walking away from the base of a lamp post at a speed of 1.9 m/s . If the lamp is 5 m above the ground , find the length of her shadow after 4 seconds (in metres )
View Solution
Knowledge Check
- Question 1 - Select One
A man of height 1.8 metre is moving away from a lamp post at the rate of 1.2m/sec. If the height of the lamp post be 4.5 metre, then the rate at which the shadow of the man is lengthening is
A0.4m/secB0.8m/secC1.2m/secDNone of these - Question 2 - Select One
A man of height 'h' is walking away from a street lamp with a constant speed 'v'. The height of the street lamp is 3h. The rate at which the length of the man's shadow is increasing when he is at a distance 10 h from the base of the street lamp is
Av/2Bv/3C2vDv/6 - Question 3 - Select One
A man of height 'h'is walking away from a street lamp with a constant speed'v'.The height of the street lamp is 3hThe rate at which of the length of man's shadow is increasingwhen he is at a distance 10hfrom the base of the street lamp is:
Av/2Bv/3C2vDv/6
A lamp is 3.3 m be the lamp post and CD= 110 cm tall walks away from the base of this lamp post at a speed of 0.8 m/s. find the length of the shadow of boy after 4 seconds.
View SolutionA lamb is 3.3 m above the ground. A boy 110 cm tall walks away from the base of this lamp at a speed of 0.8 m/s. Find the length of the shadow of the boy after 4 second.
 View Solution
View SolutionA man of height 180 cm is moving away from a lamp post at the rate of 1.2 meter per second. If the height of the lamp post is 4.5 meters, find the rate at which
(i) his shadow is lengthening. (ii) the tip of the shadow is moving.View SolutionThe foot of a ladder is 6 m away from its wall and its top reaches a window 8 m above the ground, Find the length of the ladder.
View SolutionA man 2 metres tall walks away from a lamp post 5 metres height at the rate of 4.8 km/hr. The rate of increase of the length of his shadow, is
View Solution

