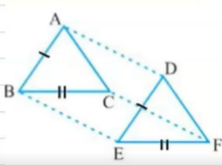
If ABC and DEF are two triangles such that AB,BC are respectively equal and parallel to DE,EF, then show that (i) quad ABED is a parallelogram (ii) quad BCFE is a paralellogram (iii) AC=DF (iv) ΔABC≅ΔDEF
(i) Consider the quadrilateral ABED We have, AB=DE and AB∥DE
One pair of opposite sides are equal and parallel.
Therefore,ABED is a parallelogram.
(ii) In quadrilateral BEFC ,
we have BC=EF and BC ∥ EF.
One pair of opposite sides is equal and parallel. therefore, BEFC is a parallelogram.(III) Since ACFD is parallelogram.
AC=DF ∣ As Opposite sides of a parallelogram ACFD
(iV) In triangles ABC and DEF, we have AB=DE ∣ (opposite sides of ABED
BC=EF ∣ (Opposite sides of BEFC
and CA=FD Opposite. sides of ACFD.Using the SSS criterion of congruence,
△ABC≅△DEF
Topper's Solved these Questions
Similar Questions
If ABC and DEF are two triangles such that AB,BC are respectively equal and parallel to DE,EF, then show that (i) quad ABED is a parallelogram (ii) quad BCFE is a paralellogram (iii) AC=DF (iv) ΔABC≅ΔDEF
View SolutionIf ABC and DEF are two triangles such that AB, BC are respectively equal and parallel to DE, EF, then show that Quadrilateral ABED is a parallelogram Quadrilateral BCFE is a parallelogram AC=DF (iv) ABC ≅ DEF
View Solution
Knowledge Check
If in a triangle AB=a,AC=b and D,E are the mid-points of AB and AC respectively, then DE is equal to
Aa4−b4Ba2−b2Cb4−a4Db2−a2In a ΔABC,D,E,F are the mid -points of the sides BC, CA and AB respectively, the vector AD is equal to
A−−→BE+−−→CFB−−→BE−−−→CFC−−→CF−−−→BED−−−→BE−−−→CF
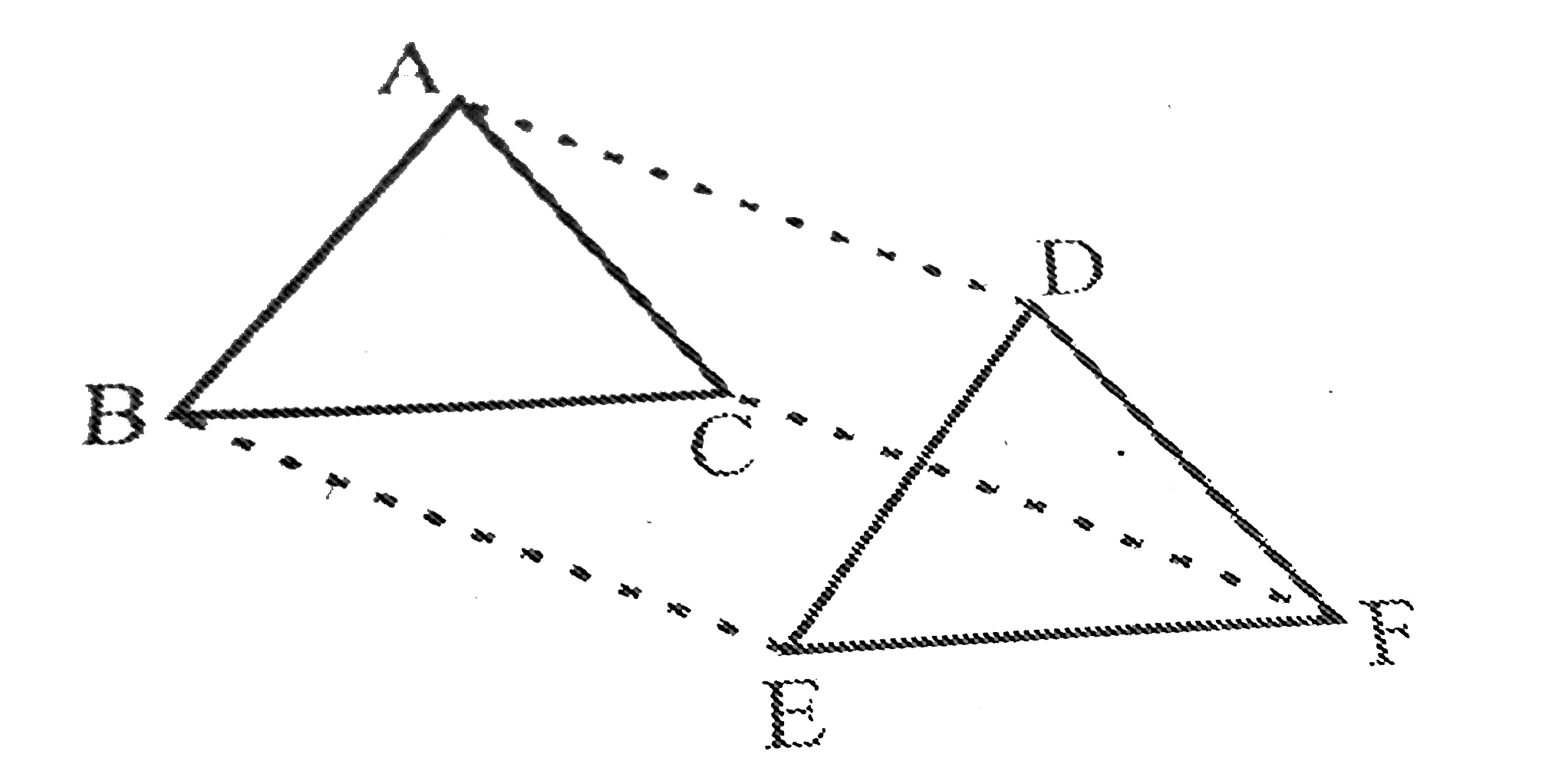
In ΔABCand ΔDEF, AB = DE, AB ∣∣ DE, BC = EF and BC ∣∣ EF. Vertices A, B and C are joined to vertices D, E and F respectively (see Fig. 8.22).Show that
(i) quadrilateral ABED is a parallelogram
(ii) quadrilateral BEFC is a parallelogram
(iii) AD || CF and AD = CF
(iv) quadrilateral ACFD is a parallelogram
(v) AC = DF
(vi) ∆ ABC ≅ ∆ DEF.
View SolutionIn a parallelogram ABCD,E,F are any two points on the sides AB and BC respectively. Show that ar(ADF)=ar(DCE).
View SolutionD, E and F are the mid-points of the sides BC, CA and AB, respectively of and equilateral ΔABC. Show that ΔDEF is also an equilateral triangle.
View SolutionIn the adjoining figure D, E and F are the mid-points of the sides BC, CA and AB respectively of ΔABC. Prove that:
(i) □BDEF is a parallelogram
(ii) area of ΔDEF=14× area of ΔABC
(iii) area of □BDEF=12× area of ΔABC

View SolutionD, E and F are the mid-points of the sides BC, CA and AB of a triangle ABC. Show that
BDEF is a parallelogram
View SolutionIn an equilateral triangle ABC, D, E, F are the mid-points of AB, BC and AC respectively.The quadrilateral BEFD is are a
(A) Square
(B) Parallelogram
(C) Rectangle
(D) Rhombus
View Solution