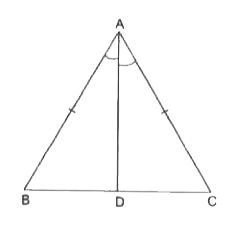
If two isosceles triangles have a common base, prove that the line joining their vertices bisects them at right angles.

Video Solution

Text Solution

Verified by Experts

Too prove=AD bisects BBC at right angles
Proof=2N $∠ A B C a n s ∠ A D C$
AB=AC(given)
BD=CD(given)
BC=BC(common)
$∠ A B C ≅ ∠ A D C (S S S)$
$∠ 1 = ∠ 2$
$I n ∠ A B E and ∠ A C E$
AB=AC
$∠ a = ∠ 2$
AE=AE(common)
$∠ A B E ≅ ∠ A C E (S A S)$
BE=CE
$∠ 3 = ∠ 4$
$∠ 3 + ∠ 4 = 180^{\circ}$
$2 ∠ 3 = 180^{\circ}$
$∠ 3 = 90^{\circ}$ .

Updated on:21/07/2023

Find the in-centre of the right angled isosceles triangle having one vertex at the origin and having the other two vertices at (6, 0) and (0, 6).
A $3 (2 + \sqrt{2}), 3 (2 + \sqrt{2})$
B $3 (2 - \sqrt{2}), 3 (2 - \sqrt{2})$
C $2 + \sqrt{2}, 2 + \sqrt{2}$
D $2 - \sqrt{2}, 2 - \sqrt{2}$
The ratio of the area of two isosceles triangles having the same vertical angle (i.e. angle between equal sides) is 1:4. The ratio of their heights is
A $1 : 4$
B $2 : 5$
C $1 : 2$
D $3 : 4$