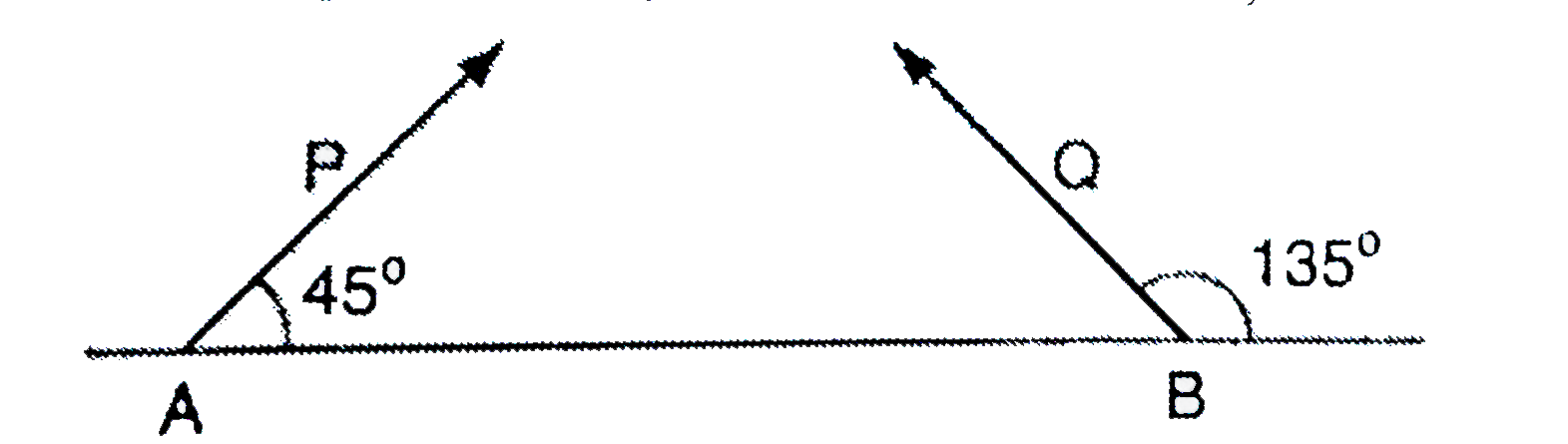
Particles P and Q of mass 20g and 40g respectively are simu ltaneously proejected from points A and B on the ground. The initial velocities of P and Q make 45∘ and 135∘ angles respectivley with the horizontal AB as shown in the Fig. 5.44 Each particle has an initial speed of 49m/s . the separation AB is 249m. both particles travel in the same vertical plane and undergo a collision. After collision P retraces its path. Determine the position of q when it hits the grou.d How much time after the collision does the particle Q take to reach the ground? (Take g=9.8m/s2)
The correct Answer is:3.53
The horizontal velocities of both the particle are the same and since both are projected simultaneously, these particle will meet exactly in the middle of AB (horizontally). Since
R=u2sin2θg=245m

⇒The collision takes place at the maximum height where the velocities of both the particles will be in the horizontal direction.
H=u2sin2θ2g=(49)2×sin2452×9.8=61.25m
Applying conservation of linear momentum in the horizontal direction with the information that P retraces its path, therefore its momentum will be the same in magnitude but different in direction.
Linear momentum of system before collision = Linear momentum of system after collision.
mp→vp+mQ→vQ=mp→vp+mQ→vQ
Here we have mp=20gm=m(say)andmQ=40gm=2m(say),
mux+2m−(ux)=m(−ux)+2m→vQ
Which gives →vQ=0
Since vQ=0, therefore the particle Q falls down vertically so it falls down on the mid-point of AB. Now considering vertical motion of Q
Using Sy−uyt+12ayt2
Here uy=0,sy=–61.25manday=–9.8m/s2 ⇒ −61.25=0.t−129.8t2.
Which gives t = 3.53 sec.

Similar Questions
Particles P and Q of mass 20g and 40g respectively are simu ltaneously proejected from points A and B on the ground. The initial velocities of P and Q make 45∘ and 135∘ angles respectivley with the horizontal AB as shown in the Fig. 5.44 Each particle has an initial speed of 49m/s . the separation AB is 249m. both particles travel in the same vertical plane and undergo a collision. After collision P retraces its path. Determine the position of q when it hits the grou.d How much time after the collision does the particle Q take to reach the ground? (Take g=9.8m/s2)

View SolutionA particle is projected from the ground with an initial velocity of 20m/s at angle fo 30∘ with the horizontal. What is the magnitude of chang in velocity in 0.5scond ? ( g= 10 m//s^2).
View Solution
Knowledge Check
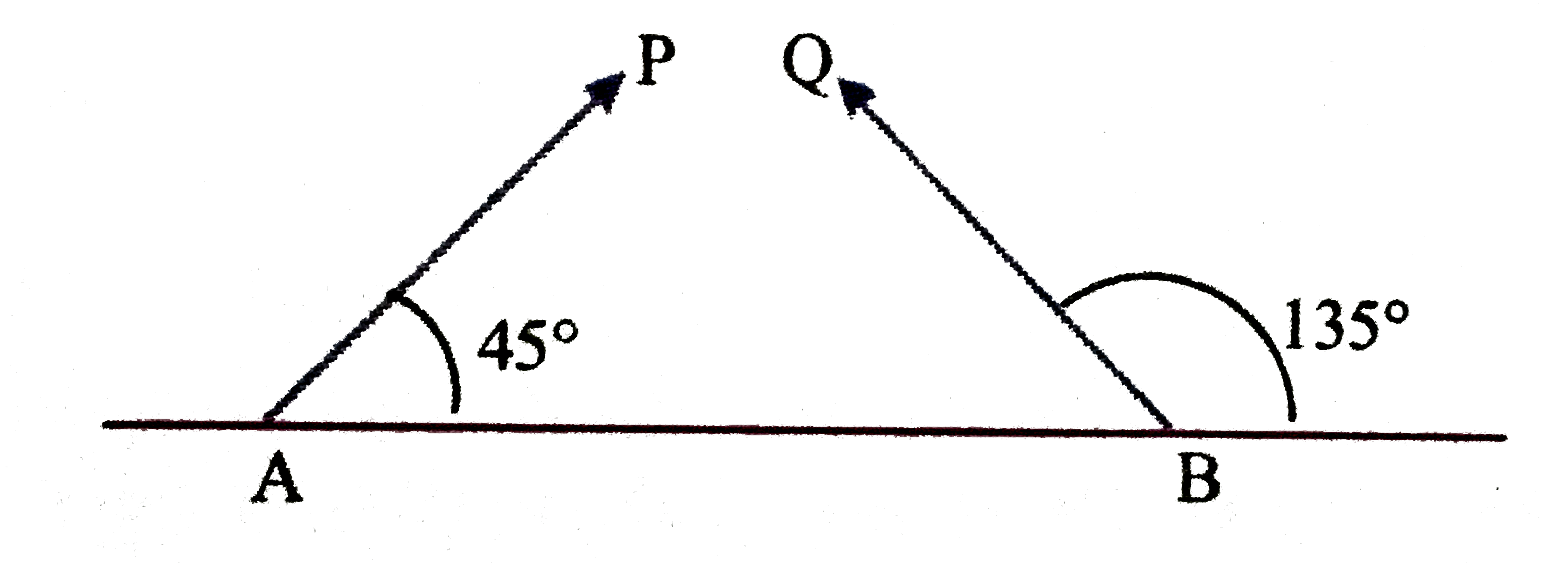
Particles P and Q of masses 20g and 40g, respectively, are projected from positions A and B on the ground. The initial velocities of P and Q make angles of 45∘ and 135∘, respectively with the horizontal as shown in the fig. Each particle has an initial speed of 49m/s. The separation AB is 245m. Both particles travel in the same vertical plane and undergo a collision. After the collision P retraces its path. The separation of Q from its initial position when it hits the ground is
 A245mB2453mC2452mD245√2m
A245mB2453mC2452mD245√2mTwo particles P & Q are projected simultaneously from a point O on a level ground in the same vertical plane with the same speed in directions making angle of 30∘ and 60∘ respectively with the horizontal.
ABoth reach the ground simultaneouslyBP reaches the ground earlier than QCBoth strike the same point on the level groundDThe maximum height attained by Q is thrice that attained by PA particle is projected from ground with some initial velocity making an angle of 45∘ with the horizontal. It reaches a height of 7.5m above the ground while it travels a horizontal distance of 10m from the point of projection. The initial speed of the projection is
A5m/sB10m/sC20m/sD40m/s
Two particles are simultaneously thrown in horizontal direction from two points on a riverbank, which are at certain height above the water surface. The initial velocities of the particles are v1=5m/sandv2=7.5m/s respectively. Both particles fall into the water at the same time. First particles enters the water at a point s = 10 m from the bank. Determine
(a) the time of flight of the two particles,
(b) the height from which they are thrown,
(c) the point where the second particle falls in water.
View SolutionTwo particles P and Q of masses 2 kg and 4 kg initially at a distance of 2m from each other are released. If the particles move under mutual attraction, then find speed of particles P when speed of Q is 5 cm/hour. Also find the separation between these particles at this instant.
View SolutionA particle is projected with velocity 50 m/s at an angle 60∘ with the horizontal from the ground. The time after which its velocity will make an angle 45∘ with the horizontal is
View SolutionTwo particles A and B are thrown simu ltaneously from the same point at the same angle of projection but with the two different initial velocities (v+u) and (v-u) respectively. Which of the following statements will b e true in respect of their motions?
View SolutionA particle is projected with an initial velocity of 200m/s in a direction making an angle of 30∘ with the vertical. The horizontal distance covered by the particle in 3s is
View Solution
