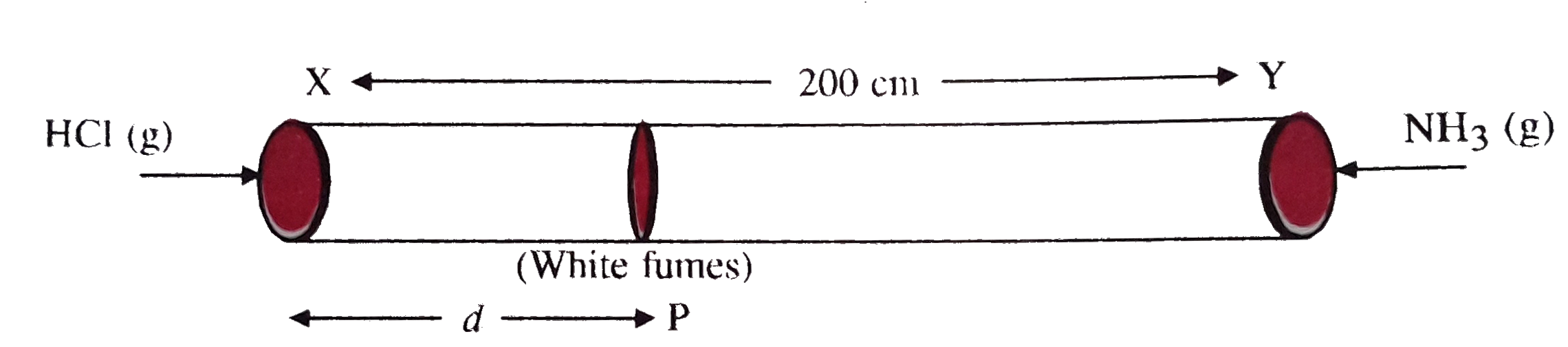
A straight glass tube has two inlets x and y at two ends. The length of the tube is 200cm. HCl gas through inlet x and NH3 gas through inlet y are allowed to enter the tube at the same time. White flames first appear at a point P inside the tube. Find the distance of P from x.
Mass of the HCl(g)=1+35.5=36.5g
Molar mass of NH3(g)=14+3×1=17.0g
Accroding to Graham's law of diffusion,
Rate of diffusion of HCl (g)Rate of diffusion ofNH2(g)=√(MNH3MHCl)=√17.036.5=0.6824
Let the distance between the points X and P = d cm
∴ Distance between te point Y and P=(200−d)cm
The distance travelled by the vapours of gas is directly proportional to rate of diffusion ∴d200−d=Rate of diffusion ofHCl(g)Rate of diffusion ofNH3(g)=0.6824
or d=0.6824×200−0.6824d
or d+0.6824=0.6824×200=136.48
or 1.6824d=136.48 or d=136.481.6824=81.12
Thus, the distance between points P and X =81.12cm
Topper's Solved these Questions
- STATES OF MATTER : GASES AND LIQUIDSBOOK - DINESH PUBLICATIONCHAPTER - STATES OF MATTER : GASES AND LIQUIDSEXERCISE - Value Based Question
3 Videos
- STATES OF MATTER : GASES AND LIQUIDSBOOK - DINESH PUBLICATIONCHAPTER - STATES OF MATTER : GASES AND LIQUIDSEXERCISE - Practice
40 Videos
- STATES OF MATTER : GASES AND LIQUIDSBOOK - DINESH PUBLICATIONCHAPTER - STATES OF MATTER : GASES AND LIQUIDSEXERCISE - Additional Important Questions
22 Videos
- STATES OF MATTER (SOLID STATE CHEMISTRY)BOOK - DINESH PUBLICATIONCHAPTER - STATES OF MATTER (SOLID STATE CHEMISTRY)EXERCISE - ULTIMATE PREPARATORY PACKAGE
21 Videos
- STRUCTURE OF ATOMBOOK - DINESH PUBLICATIONCHAPTER - STRUCTURE OF ATOMEXERCISE - Reason Type Questions
1 Videos
Similar Questions
A straight glass tube has two inlets x and y at two ends. The length of the tube is 200cm. HCl gas through inlet x and NH3 gas through inlet y are allowed to enter the tube at the same time. White flames first appear at a point P inside the tube. Find the distance of P from x.
View SolutionThough the two ends of a glass tube of length 200 cm, hydrogen chlorine gas and ammonia are allowed to enter. At what distance ammonium chloride will first appear ?
View Solution
Knowledge Check
A straight glass tube as shown, has 2 inleta X and Y at the two ends of 200 cm long tube. HCl gas through inlet X and NH3 gas through inlet Y are allowed to enter in the tube at the same time and pressure at a point P inside the tube. The distance of point P from X is:
A118.9cmB81.1cmC91.1 cmD108.9cmA bottle of dry NH3 and another bottle of dry HCl connected through a long tube are opened simultaneously at both ends of the tube. The white ring (NH4Cl) first formed will be
AAt the centre of the tubeBNear the HCl bottleCNear the ammonia bottleDThroughout the length of the tubeTwo balls of mass 'm' and 'm10' hang at the two ends of a string that passes through a fixed tube and one of the balls lies in the tube, while the other is outside of the tube. The mass 'm10' is rotating about PQ, as shown in figure. Coefficient of friction between the tube and the ball is '1'. Ball which is insid the tube remains in rest and the time period of revolution of the smaller ball is maximum . Find the angle 'θ' which is made by the string with vertical PQ.
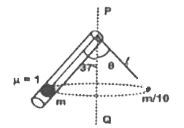 A53∘B30∘C60∘D37∘
A53∘B30∘C60∘D37∘
Through the two ends of a glass tube of length 200cm hydrogen chloride gas and ammonia are allowed to enter At what distance ammonium chloride will first appear ? .
View SolutionFind the equation of a curve passing through the point (1.1) if the perpendicular distance of the origin from the normal at any point P(x, y) of the curve is equal to the distance of P from the x-axis.
View SolutionA line through point A(1,3) and parallel to the line x-y+1 = 0 meets the line 2x-3y + 9 = 0 at point P. Find distance AP without finding point P.
View SolutionA steel tube of length 1.00 m is struck at one end. A person with his er close to the othr end hears the sound of the blow twice one travelign through the body of the tube and the other through the air in the tube. Find the time gap between the two hearings. Use the table in the next for speeds of sound in various substances.
View SolutionA small particle of mass m moving inside a heavy, hollow and straight tube along the tube axis undergoes elastic collision at two ends. The tube has no friction and it is closed at one end by a flat surface while the other end is fitted with a heavy movable flat piston as shown in figure. When the distance of the piston from closed end is L=L0 the particle speed is v=v0 The piston is moved inward at a very low speed V such that V<<dLLv0, where dL is the infinitesimal displacement of the piston. Which of the following statements(s) is(are) correct?
View Solution
