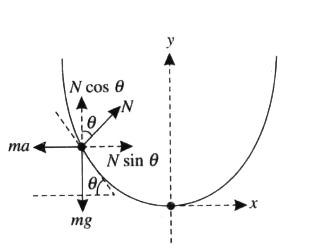
A piece of wire is bent in the shape of a parabola (y-axis vertical) with a bead of mass m on it. The bead can slide on the wire without friction. It stays at the lowest point of the parabola when the wire is at rest. The wire is now accelerated parallel to the x-axis with a constant acceleration a. The distance of the new equilibrium position of the bead, where the bead can stay at rest with respect to the wire, from the y-axis is:
The correct Answer is:B
Similar Questions
A piece of wire is bent in the shape of a parabola y=kx^(2) (yaxis vertical ) with a bead of mass m on it . The bead can slide on the wire without friction . It stays at the lowest pint of the parabola when the wire is at rest.The wire is now accelerated paralle to the x-axis with a constant acceleration a. The distance of the new equilibrium position of the bead. Find the X-coordinate where the bead can stay at rest with respect to the wire?
View SolutionParabola to a piece of string y = kx^(2) (y-axis vertical) is folded into a shape above which a bead of m mass, the bead can slip without friction. When the wire is stationary, the bead is at its minimum point. The parallel is accelerated. From the y axis in the position of pearl equinox x_(0) At a distance where the pearl is at rest with respect to the wire x_(0) What will be the value of?
05:03View Solutionचित्रानुसार परवलय की समीकरण x2=4 ay है । इसकी ऊर्ध्वाधर अक्ष तथा शीर्ष मूल बिंदु पर है, इस परवलय तार पर m द्रव्यमान की मनका स्थित है। तार का फ्रेम स्थिर एवं ऊर्ध्वाधर तल में है तथा मनका (मनका) बिना घर्षण के परवलय पर फिसल सकता है। तार फ्रेम पर y=4a बिंदु से मनका स्थिरावस्था से छोड़ा जाता है। जब मनका y=a स्थिति पर पहुंचता है तो इसका स्पर्शरेखीय त्वरण है:
 03:21View Solution
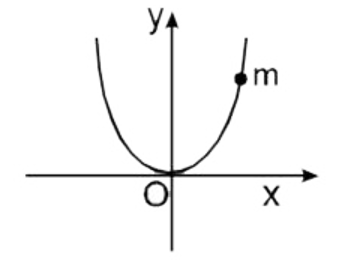
03:21View SolutionA bead of mass m is located on a parabolic wire with its axis vertical and vertex at the origin as shown in figure and whose equastion is x2=4ay. The wire frame is fixed and the bead is released from the point y=4a on the wire frame from rest. The tangential acceleration of the bead when it reaches the position given by y=a is
 05:40View Solution
05:40View SolutionA piece of wire is bent in the shape of a parabola y=kx^(2) (y-axis vertical) with a bead of mass m on it. The bead can slide on the wire without friction. It stays at the lowest point of the parabola when the wire is at rest. The wire is now accelerated parallel to the x-axis with a constant acceleration a. The distance of the new equilibrium position of the bead, where the bead can stay at rest with respect to the wire, from the y-axis is:
04:04View SolutionA piece of wire is bent in the shape of a parabola y=kx^(2) (y-axis vertical) with a bead of mass m on it. The bead can slide on the wire without friction. It stays at the lowest point of the parabola when the wire is at rest. The wire is now accelerated parallel to the x-axis with a constant acceleration a. The distance of the new equilibrium position of the bead, where the bead can stay at rest with respect to the wire, from the y-axis is:
04:16View SolutionA bead of mass m is located on a parabolic wire with its axis vertical and vertex at the origin as shown in figure and whose equastion is x^(2) =4ay . The wire frame is fixed and the bead is released from the point y=4a on the wire frame from rest. The tangential acceleration of the bead when it reaches the position given by y=a is
03:10View SolutionA piece of wire is bent in the shape of a parabola y=kx^(2) (y-axis vertical) with a bead of mass m on it. The bead can slide on the wire without friction. It stays at the lowest point of the parabola when the wire is at rest. The wire is now accelerated parallel to the x-axis with a constant acceleration a. The distance of the new equilibrium position of the bead, where the bead can stay at rest with respect to the wire, from the y-axis is:
05:42View Solution