A string of length 1m is fixed at one end and carries a mass of 1 kg at the other end. The string makes 2/π revolutions per second around a vertical axis passing through the fixed end. Calculate (i) angle of inclination of the string with the vertical, (ii) the tension in the strong and (iii) the linear velocity of the mass.
As shown in Fig. 7.67, for vertical equilibrium of mass m
Tcosθ=mg……(i)
While for circular motion
Tsinθ=(mv2/r)=mrω2 ……..(ii)
(a) So , from Eqn . (ii) , T=[mrω2/sinθ]=mLω2
[as r = L sin θ]
∴ T=10−1×1×42=1.6N [ as ω=2πf=2π×(2/π)=4]
(b) From Eqn. (i) , cos θ=mgT=10−1×101.6=58,
i.e., θ=cos−1(5/8)
(c) v=rω=Lsinθ×ω [ as r = L sin θ]
or v=1×0.78×4=3.12 m/s [ as sin θ=0.78]
Topper's Solved these Questions
- FRICTION AND CIRCULAR MOTION BOOK - GRB PUBLICATIONCHAPTER - FRICTION AND CIRCULAR MOTION EXERCISE - Problem For Practice
23 Videos
- FRICTION AND CIRCULAR MOTION BOOK - GRB PUBLICATIONCHAPTER - FRICTION AND CIRCULAR MOTION EXERCISE - OBJECTIVE QUESTIONS ( only one choice is correct )
80 Videos
- FORCE AND NEWTONS LAWS OF MOTIONBOOK - GRB PUBLICATIONCHAPTER - FORCE AND NEWTONS LAWS OF MOTIONEXERCISE - Comprehension
12 Videos
- MOTION IN A STRAIGHT LINEBOOK - GRB PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Comprehension type Queston
14 Videos
Similar Questions
A string of length 1 m is fixed at one end and carries a mass of 100 gm at the other end. The string makes 2/πrevolutions per second about a vertical axis through the fixed end. The angle of inclination of the string with the vertical, and the linear velocity of the mass will respec- tively be - (in M.K.S. system)
View SolutionA string of length 50cm is fixed at one end and carries a mass of 200g at the other end. The string makes 5/2π revolution per second around the verticle axis through the fixed end. Calculate (a) the tension in the string (b) the angle of incilination of the string with verticle and (c) linear velocity of the mass.
View Solution
Knowledge Check
A string of length 1 m is fixed at one end and carries a mass of 100 gm at the other end. The string makes (2/π) revolutions per second around vertical axis through the fixed end. Calculate the tension in the string-
A1.0 NB1.6 NC2ND4NA string of length l=1m is fixed at one end carries a mass of 100gm at other end. The string makes √5/(π) revolutions per second about a verticle axis passing through its second end. What is the angle of inclination of the string with the vertical?
A30∘B45∘C60∘D75∘A string of length L is fixed at one end and carries a mass M at the other end. The string makes 2/π revolution per second around the vertical axis through the fixed end as shown in the figure, then tension in the string is.
 AMLB2MLC4MLD16ML
AMLB2MLC4MLD16ML
A string of length L is fixed at one end and carries a mass M at the other end. The string makes 2π rev/s around the vertical axis through the fixed end as shown in figure, then tension in string is
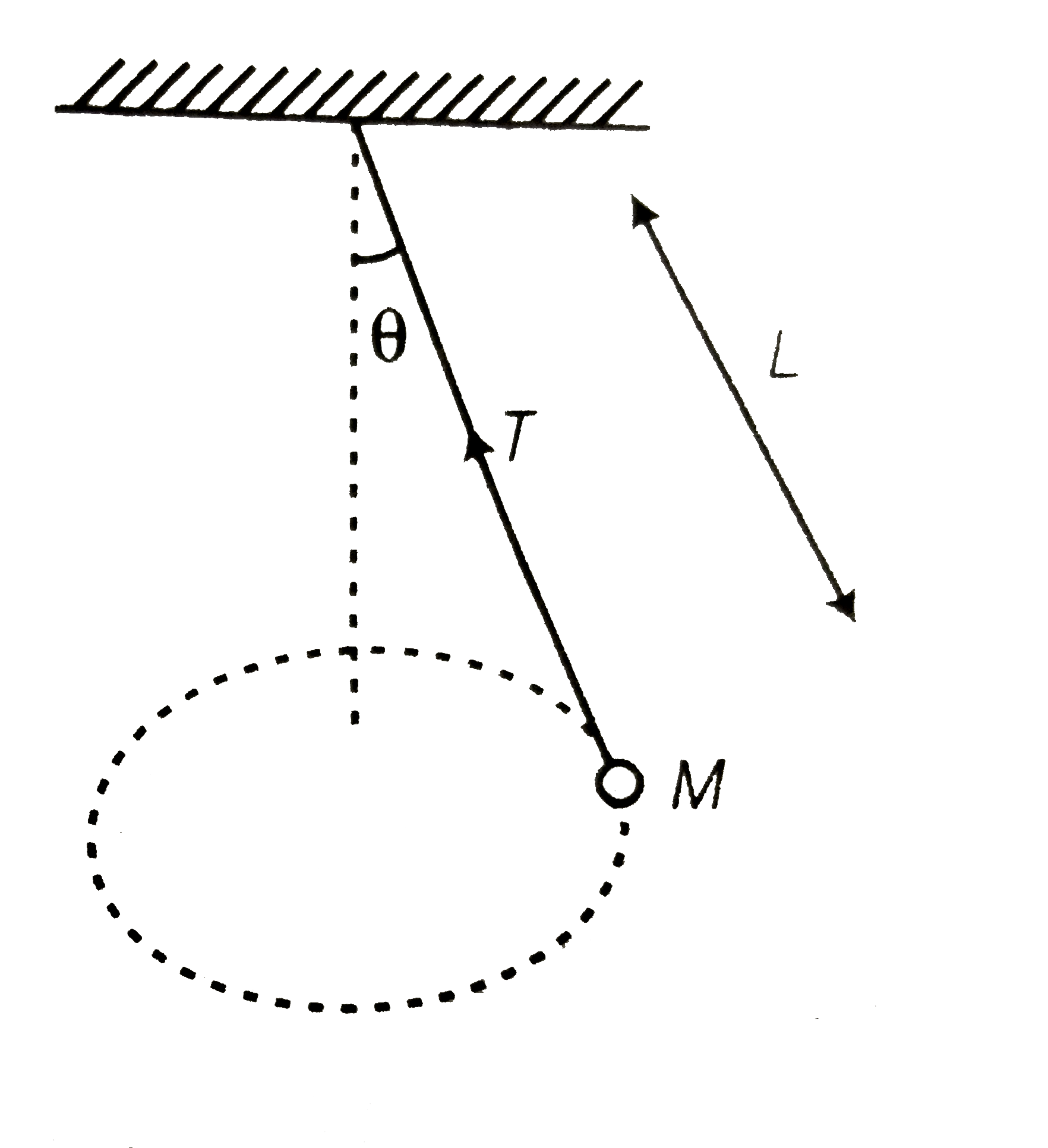
View SolutionA string of length 0.5 m carries a bob of mass 0.1 kg at its end. If this is to be used as a concial pendulum of period 0.4πs s, calculate the angle of inclination of the string with the vertical and the tension in the string .
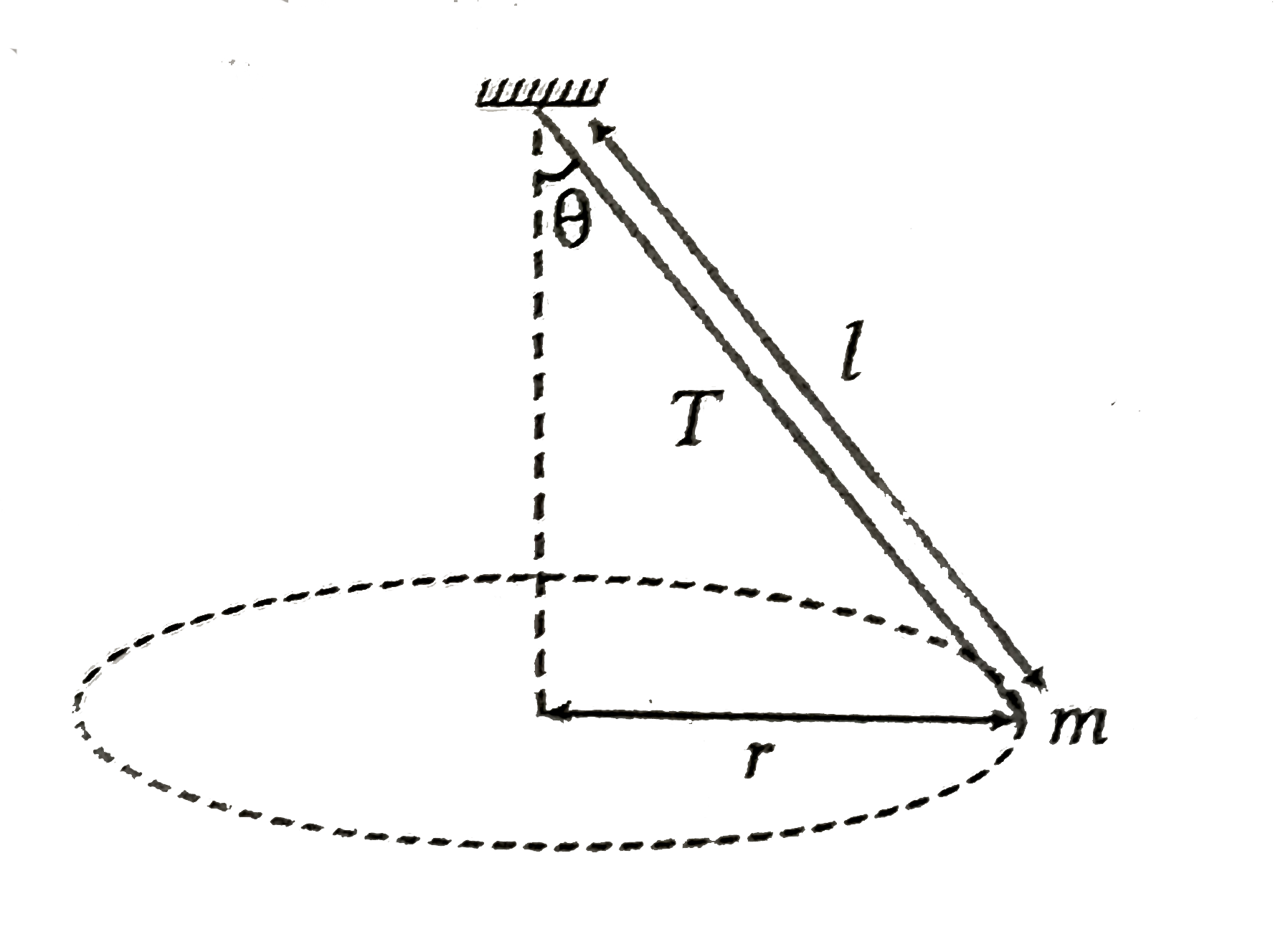
View SolutionA string of length l fixed at one end carries a mass m at the other end. The strings makes 2πrevs−1 around the axis through the fixed end as shown in the figure, the tension in the string is
View SolutionIn a conical pendulum arrangement, a string of length 1 m is fixed at one end with a bob of mass 100 g and the string makes 2πmvs−1around a vertical axis through a fixed point. The angle of inclination of the string with vertical is: (Take g=10ms−1)
View SolutionIn a conical pendulum arrangement, a string of length 1 m is fixed at one end with a bob of mass 100g and the string makes 4radpersec around a vertical axis through a fixed point. The angle of inclination of the string with vertical is: (Take g=10ms−1)
View Solution
