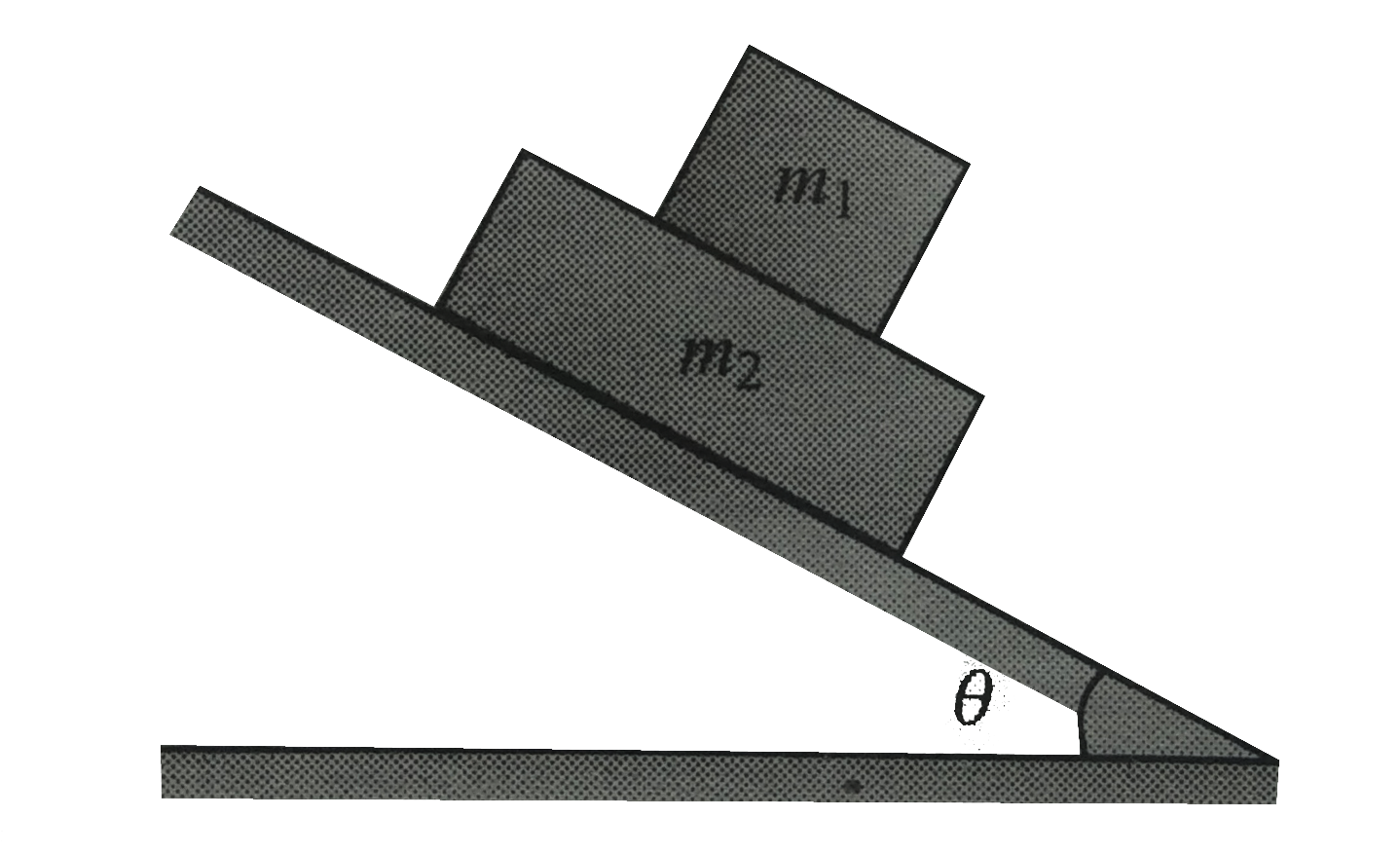
Two blocks m1 and m2 are allowed to move without friction. Block m1 is on block m2 and m2 slides on smooth fixed incline as shown. The angle of inclination of inclined plane is θ
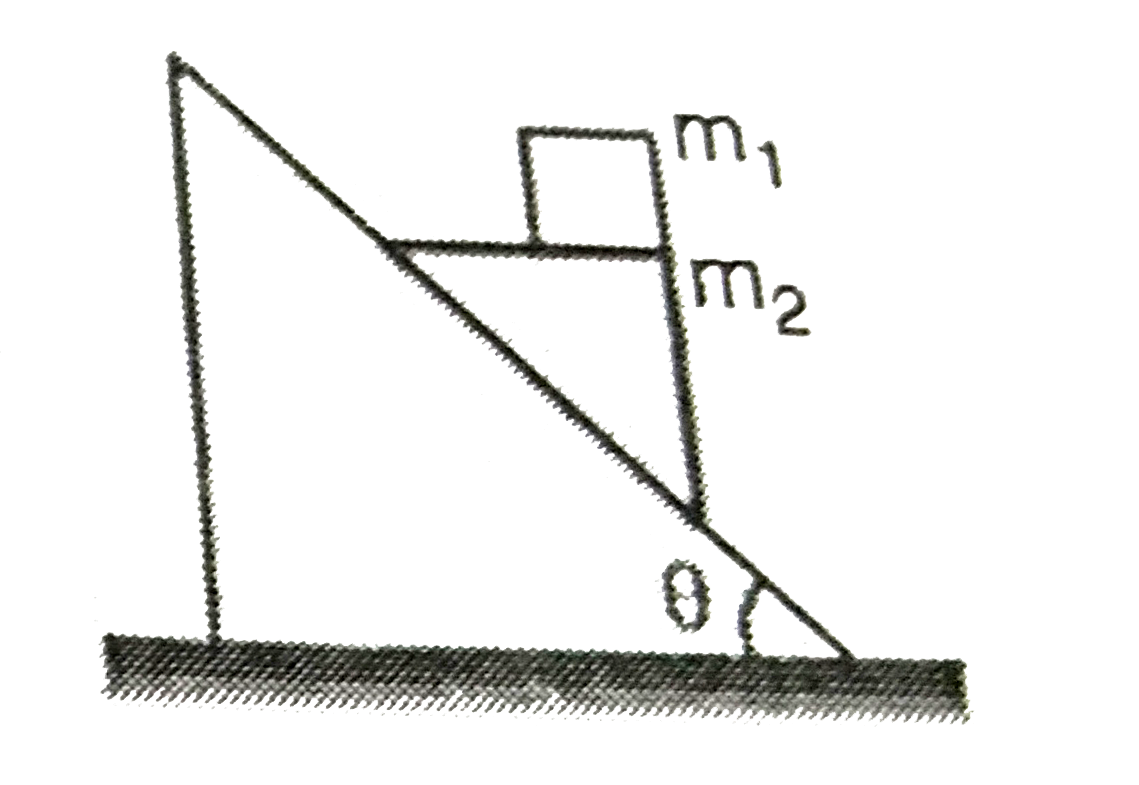
The acceleration of m1 with respect to ground is:
(m1+m2)gsin2θm2+m1sin2θ
(m1+m2)gsin2θm1+m2sin2θ
(m1+m2)gsin2θm2−m1sin2θ
(m1+m2)gsin2θm1−m2sin2θ
The correct Answer is:A
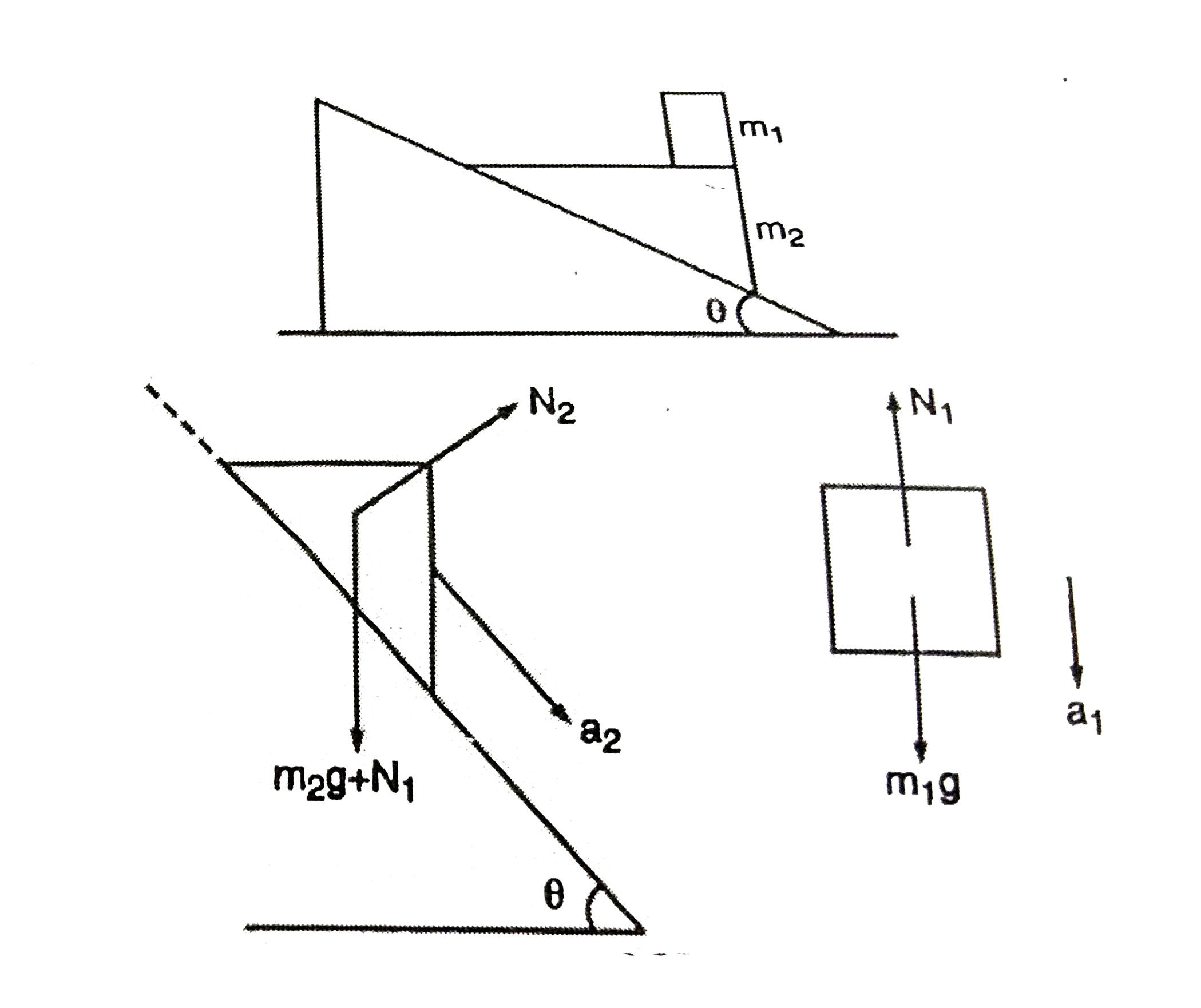
m1g−N1=m1a1 ….(i)
(N1+m2g)sinθ=m2a2 ….(ii)
From (i) and (ii)
[(m1g−m1a1)+m2g]sinθ=m2a2
(m1+m2)gsinθ−m1a1sinθ=m2a2 ....(iii)
Block m1 can move only in vertical direction as there is no force in horizontal direction. Since acceleration along normal at point of contact of the block and wedge should be equal.
So, a1=a2sinθ ...(iv)
Topper's Solved these Questions
- FORCE AND NEWTONS LAWS OF MOTIONBOOK - GRB PUBLICATIONCHAPTER - FORCE AND NEWTONS LAWS OF MOTIONEXERCISE - Integer question
15 Videos
- BASIC MATHEMATICSBOOK - GRB PUBLICATIONCHAPTER - BASIC MATHEMATICSEXERCISE - Problems For Practice
35 Videos
- FRICTION AND CIRCULAR MOTION BOOK - GRB PUBLICATIONCHAPTER - FRICTION AND CIRCULAR MOTION EXERCISE - Comprehension type
11 Videos
Similar Questions
A block of mass m is released from the top of fixed inclined smooth plane. if theta is the angle of inclination then vertical accelertion of block is
View SolutionA cubical block of mass m is sliding on an inclined right angle trough as shown in the figure. If coefficient of friction is μ , find the acceleration of the block.
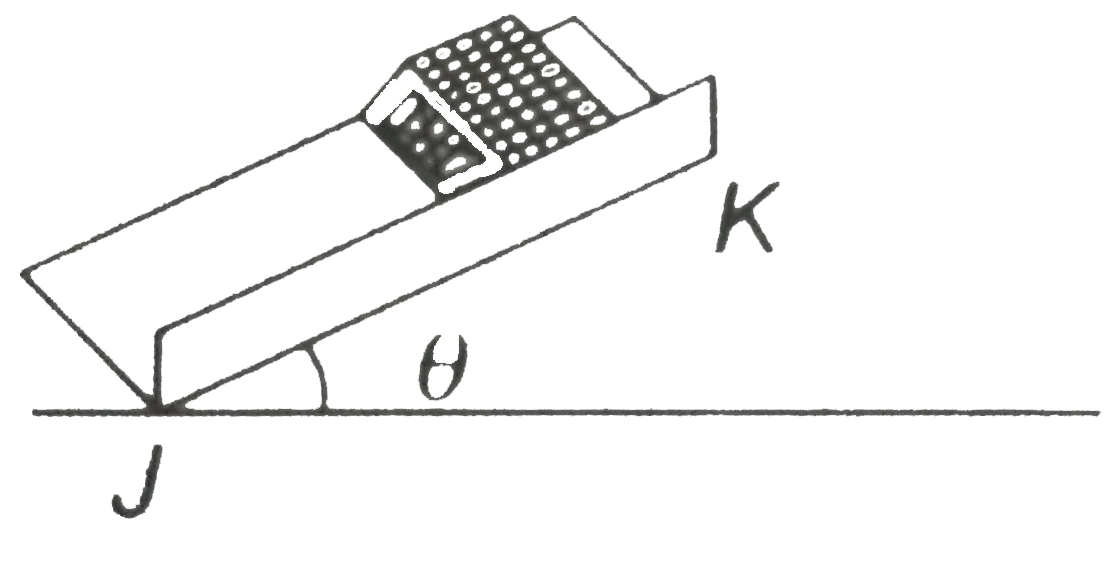
View Solution
Knowledge Check
Two blocks m1 and m2 are allowed to move without friction Block m1 is on block m2 and m2 slides on smooth fixed incline as shown The angle of inclination of inclined plane is θ
 .
.
Normal reaction on m1 is .Am1gB(m1+m2)gCm1m2cos2θm2+m1sin2θDm1g[1−(m1+m2)sin2θ]m1+m2sin2θBlock M slides down on frictionless incline as shown. The minimum friction coefficient so that m does not slide with respect to M would be :
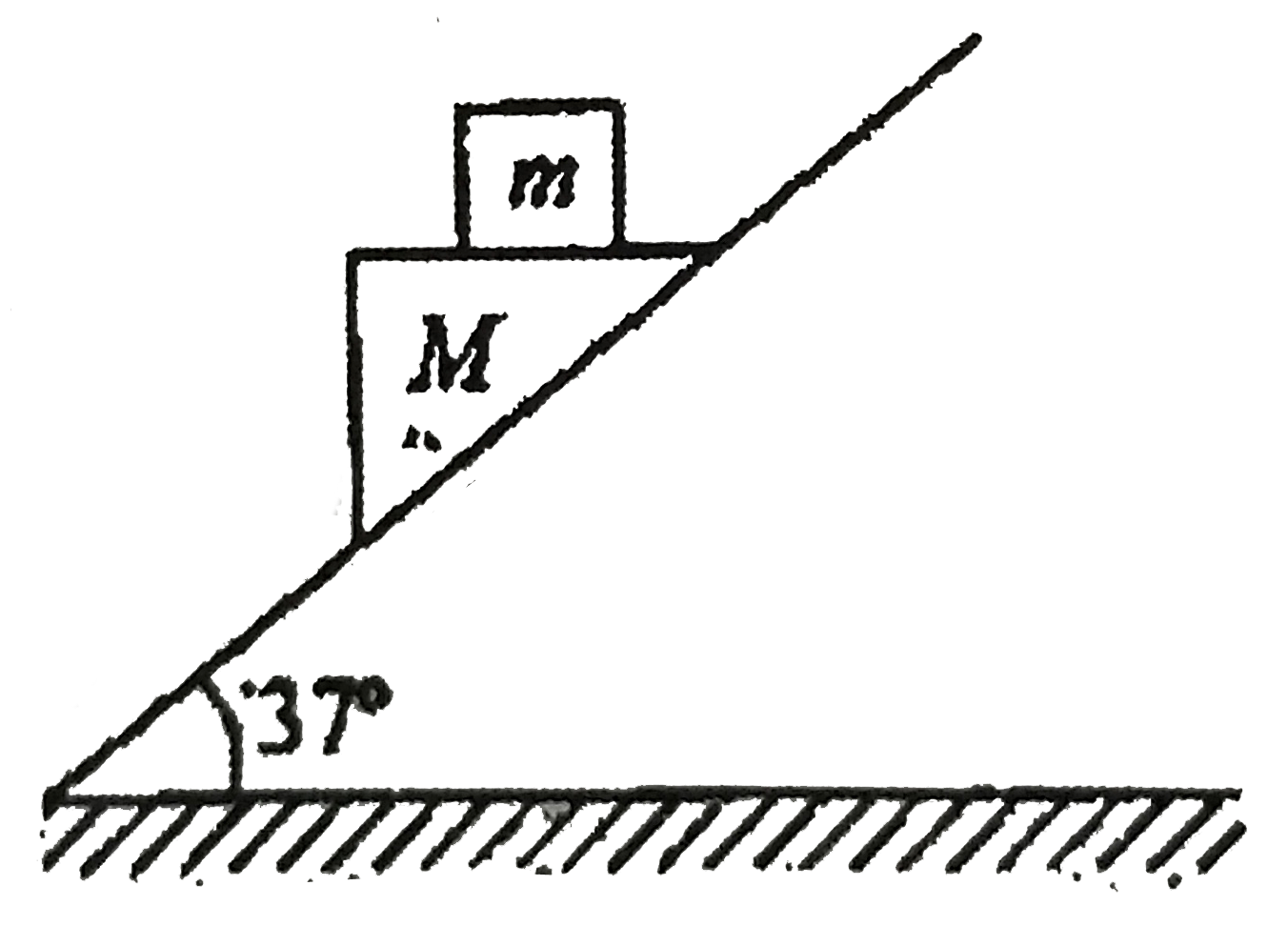 A14B12C34DNone of these
A14B12C34DNone of theseTwo block of masses m1 and m2 are connected as shown in the figure. The acceleration of the block m2 is:
 Am2gm1+m2Bm1gm1+m2C4m2g−m1gm1+m2Dm2gm1+4m2
Am2gm1+m2Bm1gm1+m2C4m2g−m1gm1+m2Dm2gm1+4m2
In fig Block m2 is loaded on block m1 . If there is no relative sliding between the block and the inclined plane is smooth , find the friction force between the block
View SolutionA block of mas m begins to slide down on an inclined plane of inclination θ. The force of friction will be
View Solution
Two blocks of masses m1 and m2 are connected as shown in the figure. The acceleration of the block m2 is :
View SolutionA block can slide on a smooth inclined plane of inclination θ kept on the floor of a lift. When the lift is descending with a retardation a , the acceleration of the block relative to the incline is
View SolutionTwo masses M1andM2 are attached to the ends of string which passes over the pulley attached to the top of a double inclined plane. The angles of inclination of the inclined planes are αandβ. Take g=10ms−2. If M1=M2 and α=β, what is the acceleration of the system?
View Solution
