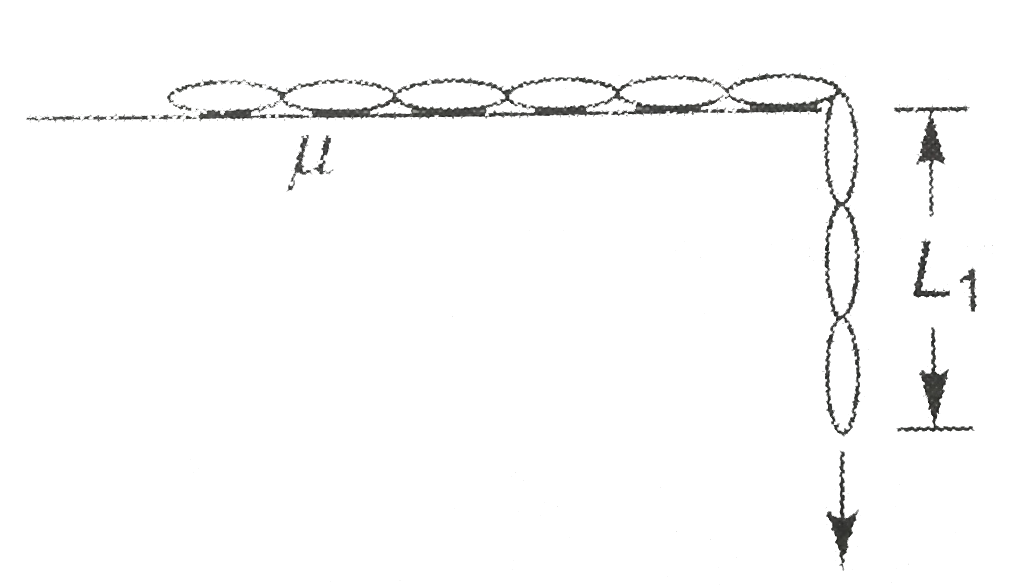
Assuming the length of a chain to be L and coefficient of static friction $μ$ . Compute the maximum length of the chain which can be held outside a table without sliding.

Video Solution

Text Solution

Verified by Experts

If y is the maximum length of the chain which can hang outside the table without sliding , then for equilibrium of the chain , the weight of hanging part must be balanced by force of friction on the portion on the table :

$W = f_{L} ... ... .$ (i)
But from Fig . 7.37 ,
$W = \frac{M}{L}$ yg and $R = W' = \frac{M}{L} (L - y) g$
So that , $f_{L} = μ R = μ \frac{M}{L} (L - y) g$
Substituting these values of W and $f_{L}$ in Eqn. (i) we get
$\frac{M}{L} y g = μ \frac{M}{L} (L - y)$ g
which on simplification yields $y = μ L (1 + μ)$ .

Updated on:21/07/2023

A uniform rope of length l lies on a table. If the coefficient of friction is $μ$ , then the maximum length L of the part of this rope which can overhang from the edge of the table without sliding down is
A $\frac{l}{μ}$
B $\frac{l}{μ + 1}$
C $\frac{μ l}{1 + μ}$
D $\frac{μ l}{μ - 1}$
A uniform rope of length l lies on a table if the coefficient of friction is , then the maximum length l, of the part of this rope which can over hang from the edge of the table without sliding down is -
A $\frac{l}{μ}$
B $\frac{l}{μ} + 1$
C $\frac{μ l}{μ + 1}$
D $\frac{μ l}{μ - 1}$
A uniform rope of length l lies on a table . If the coefficient of friction is $μ$ , then the maximum length $l_{1}$ of the part of this rope which can overhang from the edge of the table without sliding down is :
A $\frac{1}{μ}$
B $\frac{1}{μ + 1}$
C $\frac{μ l}{μ + 1}$
D $\frac{μ l}{μ - 1}$