A particle moves in circle of radius R with a constant speed v. Then, find the magnitude of average
acceleration during a time interval πR2v.
3√2V2/πR
2√2V2/πR
3V2/πR
2V2/πR
The correct Answer is:B
To find the magnitude of the average acceleration of a particle moving in a circle of radius R with a constant speed v during a time interval of πR2v, we can follow these steps:
Step 1: Determine the distance traveled
The distance d traveled by the particle in the given time interval can be calculated using the formula:
d=v⋅t
Substituting the time interval t=πR2v:
d=v⋅(πR2v)=πR2
Step 2: Find the angle covered
The distance πR2 corresponds to an angle θ in radians. The relationship between the arc length s, radius R, and angle θ is given by:
s=Rθ
Thus, we can find θ:
πR2=Rθ⟹θ=π2 radians
This means the particle moves through a quarter of a circle.
Step 3: Determine the initial and final velocity vectors
Assuming the particle starts at point A (0, R) and moves to point B (R, 0) in the quarter circle:
- Initial velocity →vi at point A (0, R) is directed tangentially to the circle, which is horizontally to the right:
→vi=(v,0)
- Final velocity →vf at point B (R, 0) is directed tangentially to the circle, which is vertically downwards:
→vf=(0,−v)
Step 4: Calculate the change in velocity
The change in velocity Δ→v is given by:
Δ→v=→vf−→vi=(0,−v)−(v,0)=(−v,−v)
Step 5: Find the magnitude of the change in velocity
The magnitude of the change in velocity is:
|Δ→v|=√(−v)2+(−v)2=√2v2=v√2
Step 6: Calculate the average acceleration
The average acceleration →aavg is defined as the change in velocity divided by the time interval:
→aavg=Δ→vt=v√2πR2v=2v2√2πR
Thus, the magnitude of the average acceleration is:
|→aavg|=2√2v2πR
Final Answer
The magnitude of the average acceleration during the time interval πR2v is:
|→aavg|=2√2v2πR
---
The distance d traveled by the particle in the given time interval can be calculated using the formula:
d=v⋅t
Substituting the time interval t=πR2v:
d=v⋅(πR2v)=πR2
The distance πR2 corresponds to an angle θ in radians. The relationship between the arc length s, radius R, and angle θ is given by:
s=Rθ
Thus, we can find θ:
πR2=Rθ⟹θ=π2 radians
This means the particle moves through a quarter of a circle.
Assuming the particle starts at point A (0, R) and moves to point B (R, 0) in the quarter circle:
- Initial velocity →vi at point A (0, R) is directed tangentially to the circle, which is horizontally to the right:
→vi=(v,0)
- Final velocity →vf at point B (R, 0) is directed tangentially to the circle, which is vertically downwards:
→vf=(0,−v)
The change in velocity Δ→v is given by:
Δ→v=→vf−→vi=(0,−v)−(v,0)=(−v,−v)
The magnitude of the change in velocity is:
|Δ→v|=√(−v)2+(−v)2=√2v2=v√2
The average acceleration →aavg is defined as the change in velocity divided by the time interval:
→aavg=Δ→vt=v√2πR2v=2v2√2πR
Thus, the magnitude of the average acceleration is:
|→aavg|=2√2v2πR
The magnitude of the average acceleration during the time interval πR2v is:
|→aavg|=2√2v2πR
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - MOTIONCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE - 3 SECTION -A
14 Videos
- CIRCULAR MOTIONBOOK - MOTIONCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE - 3 SECTION - B
8 Videos
- CIRCULAR MOTIONBOOK - MOTIONCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE -1
41 Videos
- CENTRE OF MASSBOOK - MOTIONCHAPTER - CENTRE OF MASSEXERCISE - Exercise - 4 Level-II
20 Videos
- COMMUNICATION SYSTEMBOOK - MOTIONCHAPTER - COMMUNICATION SYSTEMEXERCISE - EXERCISE - 3
6 Videos
Similar Questions
A particle is moving in a circle of radious R with constant speed. The time period of the particle is T = 1s. In a time interval T6, if the difference between average speed and the magnitude of average velocity of the particle is 2m/s, then report 49R50, where R is the radius of the circle (in metres) (take π=3.14 )
View SolutionA particle moves in a circle of radius R=2122m with constant speed 1m/s. Find,
(a) magnitude of average velocity and
(b) magnitude of average acceleration in 2 s.
View Solution
Knowledge Check
A particle moves in a circle of radius 5 cm with constant speed and time period 0.2πs. The acceleration of the particle is
A25ms−2B36ms−2C5ms−2D15ms−2A particle moves in a circle of radius 5 cm with constant speed and time period 0.2π. The acceleration of the particle is:
A15m/s2B25m/s2C36m/s2D5m/s2A particle moves in a circle of radius 5 cm with constant speed and time period 0.2πs. The acceleration of the particle is
A25m/s2B36m/s2C5m/s2D15m/s2
A particle is moving with a constant speed v in a circle. What is the magnitude of average velocity after half rotation?
View SolutionIf the body is moving in a circle of radius r with a constant speed v , its angular velocity is
View SolutionA particle is moving in a circle of radius r with speed v as shown in the figure. The magnitude of change in velocity in moving from P to Q is
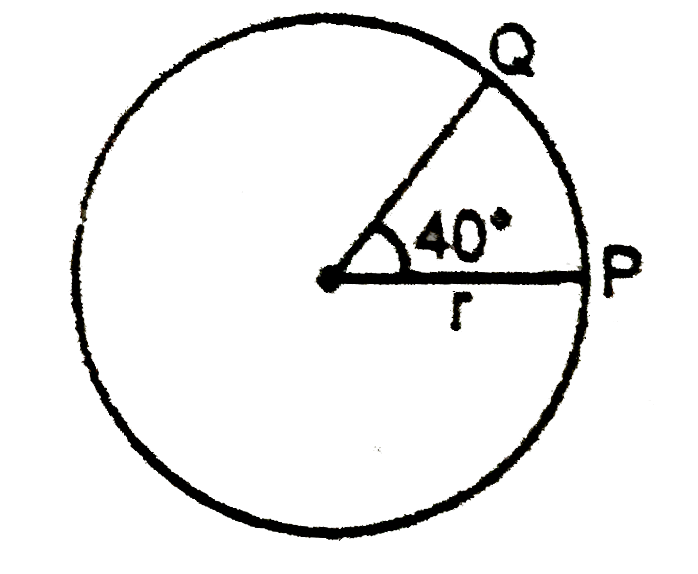
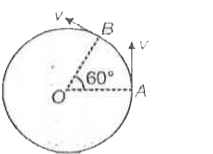
View SolutionA particle is moving in a circle of radius r having centre at O with a constant speed v . The magnitude of change in velocity in moving from A to B is
View SolutionA particle moves along a circle of radius R with a constant angular speed ω. Its displacement (only magnitude) in time t will be
View Solution
