(a) Prove the theorem of perpendicular axes.
(Hint : Square of the distance of a point (x, y) in the x-y plane from an axis through the origin and perpendicular to the plane is $^(x 2) + y^{2})$ .
(b) Prove the theorem of parallel axes.
(Hint : If the centre of mass of a system of n particles is chosen to be the origin $\sum m_{i} r_{i} = 0$ ).

Video Solution

Text Solution

Verified by Experts

(a) The theorem of perpendicular axes : According to this theorem, the moment of inertia of a plane lamina (i.e., a two dimensional body of any shape $/$ size) about any axis OZ perpendicular to the plane of the lamina is equal to sum of the moments of inertia of the lamina about any two mutually perpendicular axes OX and OY in the plane of lamina, meeting at a point where the given axis OZ passes through the lamina. Suppose at the point R m {particle is situated moment of inertia about Z axis of lamina
=moment of inertia of body about r-axis
=moment of inertia of body about y-axis.

(b) Theorem of parallel axes : According to this theorem, moment of inertia of a rigid body about any axis AB is equal to moment of inertia of the body about another axis KL passing through centre of mass C of the body in a direction parallel to AB, plus the product of total mass M of the body and square of the perpendicular distance between the two parallel axes. If h is perpendicular distance between the axes AB and KL, then Suppose rigid body is made up of n particles m1, m2, __mn, mn at perpendicular distance $r_{1}, r_{2}, r_{1} r_{n}$ respectively from the axis KL passing through centre of mass C of the body.
If h is the perpendicular distance of the particle of mass m from KL, then

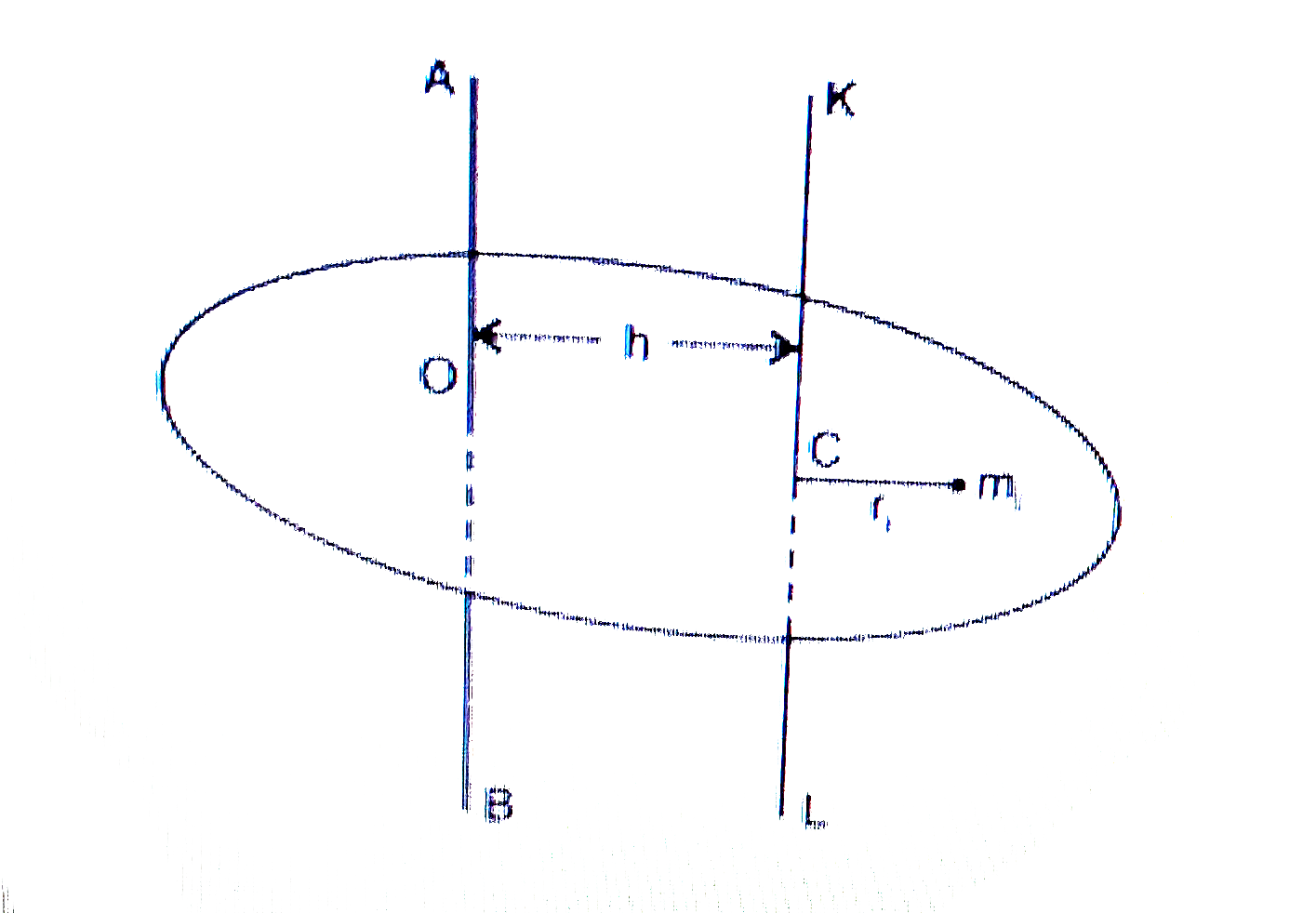
The perpendicular distance of $i^{t h}$ particle from the axis
$A B = (r_{i} + n)$
or $I_{A B} = \sum i m_{i} {(r_{i} + h)}_{i}^{2}$
$= \sum i m_{i} (r_{i}^{2} + h^{2} + 2 r_{i} h)$
$= \sum i m_{i} r_{i}^{2} + \sum i m_{i} h^{2} + 2 h \sum i m_{i} r_{i}$
As the body is balanced about the centre of mass, the algebraic sum of the moments of the weights of all particles about an axis passing through C must be zero.
$\sum i (m_{i} g) r_{i} = 0 or g \sum i m_{i} r_{i}$
or $\sum i m_{i} r_{i} = 0$
From equation (ii), we have
$I_{A B} = \sum i m_{i} r_{i}^{2} + (\sum m_{i}) h^{2} + 0$
or $I_{A B} = I_{K L} + M h^{2}$
where $I_{K L} = \sum i m_{i} r_{i}^{2} and M = \sum m_{i}$

Updated on:21/07/2023

By the theorem of perpendicular axes, if a body be in X-Z-plane then :-
A $I_{X} - I_{Y} = I_{Z}$
B $I_{Z} + I_{Y} = I_{X}$
C $I_{Z} + I_{x} = I_{Y}$
D $I_{y} + I_{Z} = I_{X}$
The equation of a plane passing through origin and perpendicular to the line x=2y=3z is
Ax+2y+3z=0
B3x+2y+z=0
C6x+3y+2z=0
D6x-3y+2z=0
Equation of the passing through the origin and perpendicular to the planes $x + 2 y + z = 1$ , $3 x - 4 y + z = 5$ is
A $x + 2 y - 5 z = 0$
B $x - 2 y - 3 z = 0$
C $x - 2 y + 5 z = 0$
D $3 x + y - 5 z = 0$