Find the magnitude of F1andF2. If F1,F2 make angle 30∘and45∘ with F3 and magnitude of F3 is 10 N.(given →F1+F2=→F3)
∣∣→F3∣∣=F1cos30∘+F2cos45∘&F2sin45∘=F1sin30∘
⇒10=√3F12+F2√2,F2√2=F12
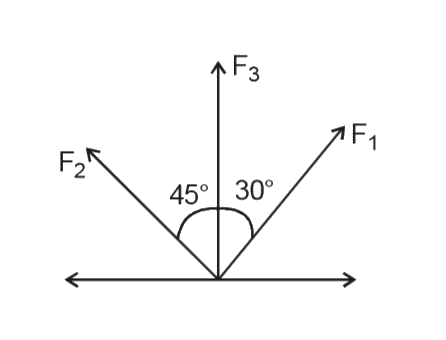
⇒F1=20√3+1&F2=20√2√3+1 .
Topper's Solved these Questions
- VECTOR & CALCULUSBook:MOTIONChapter:VECTOR & CALCULUSExercise:PHYSICAL EXAMPLE
Explore 11 Videos
- VECTOR & CALCULUSBook:MOTIONChapter:VECTOR & CALCULUSExercise:EXERCISE -1
Explore 76 Videos
- VECTOR Book:MOTIONChapter:VECTOR Exercise:Exercise - 3
Explore 18 Videos
- WAVE MOTION Book:MOTIONChapter:WAVE MOTION Exercise:Exercise - 3 Section - B (Previous Years Problems)
Explore 7 Videos
Similar Questions
Knowledge Check
The equation of a curve is y=f(x). The tangents at (1,f(1)),(2,f(2)) and (3,f(3)) make angle 30∘,60∘and45∘ respectively with +ive direction of x-axis then the value of
∫32f'(x)f''(x)dx+∫31f''(x)dx is :A√3B1√3C−√3D−1√3A smooth track in the form of a quarter circle of radius 6m lies in the vertical plane. A particle moves from P1 to P2 under the action of forces →F1,→F2 and →F3 Force →F1 is 20N, Force →F2 is always 30N in magnitude. Force →F3 always acts tangentiallly to the track and is of magnitude 15N. Select the correct alternative (s) :
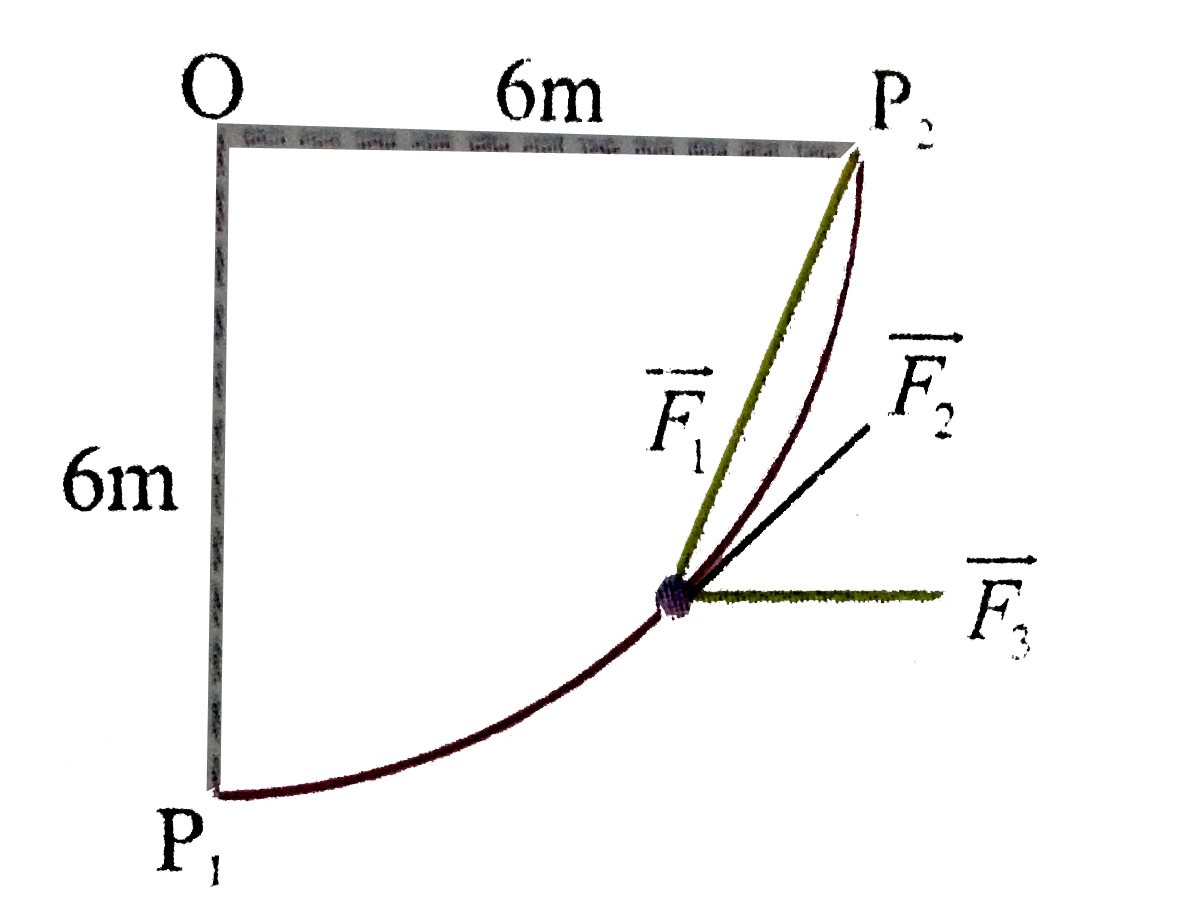 .AWork done by →F1 is 120JBWork done by →F2 is 180JCWork done by →F3 is 45πJD→F1 is conservative in nature.
.AWork done by →F1 is 120JBWork done by →F2 is 180JCWork done by →F3 is 45πJD→F1 is conservative in nature.In above example correct relation between magnitude of f1 and f2 is
Af1>f2Bf2>f1Cf1=f2Dnot possible to decide due to insufficient data.
Two forces →F1=2ˆi+2ˆjNand→F2=3ˆi+4ˆkN are acting on a particle
(a) Find the resultant force acting on particle
(b) (b) Find the angle between−→F1&−→F2
(c) Find the componant of force −→F1 along force −→F2.
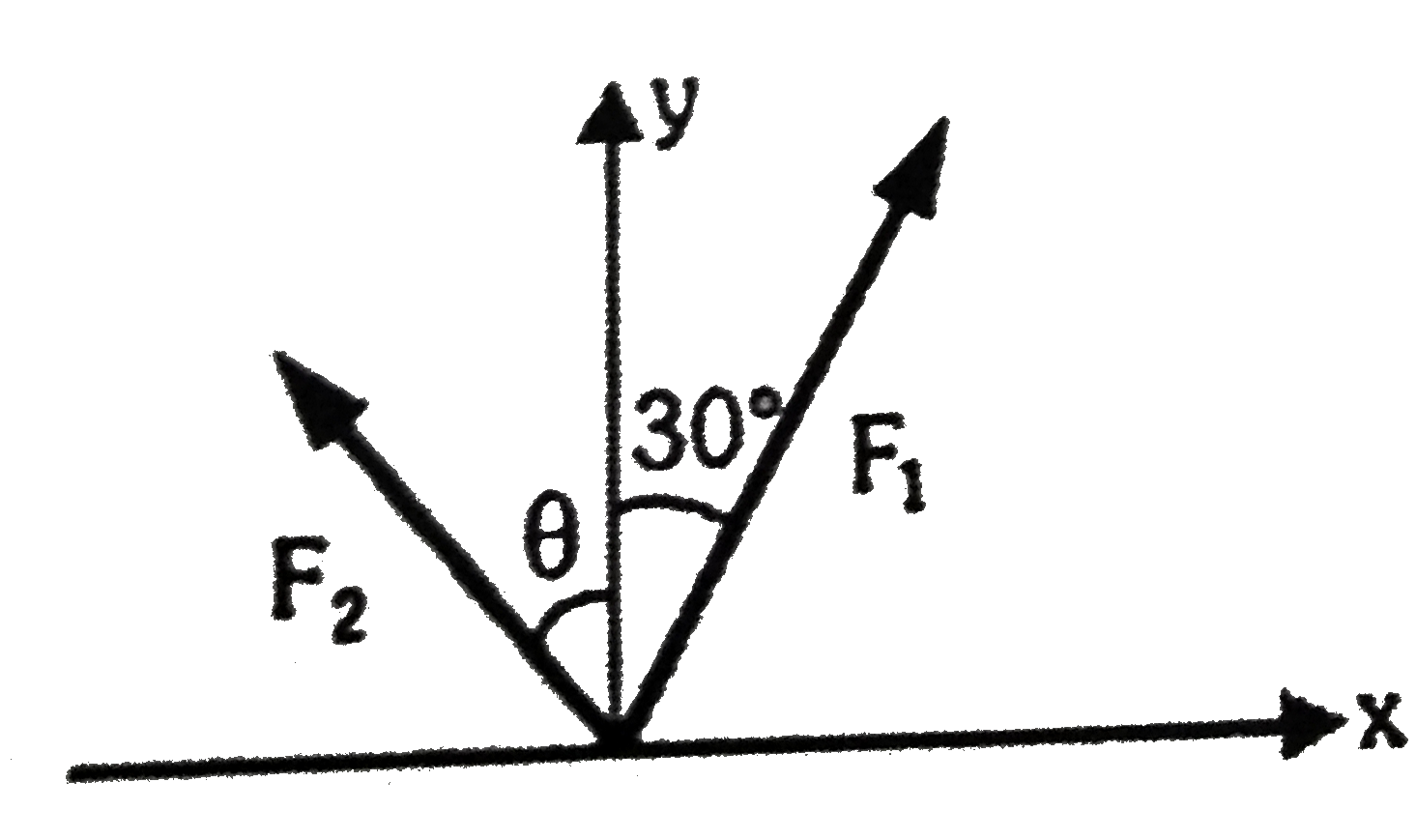
View SolutionIn the figure, F1 and F2, the two unkown forces give a resultant force of 80√3N along the y-axis. It is required that F2 must have minimum magnitude. Find tht magnitude of F1 and F2
View SolutionFour forces act on a particle as shown in the figure such that net force is zero. Then consider following statement :−
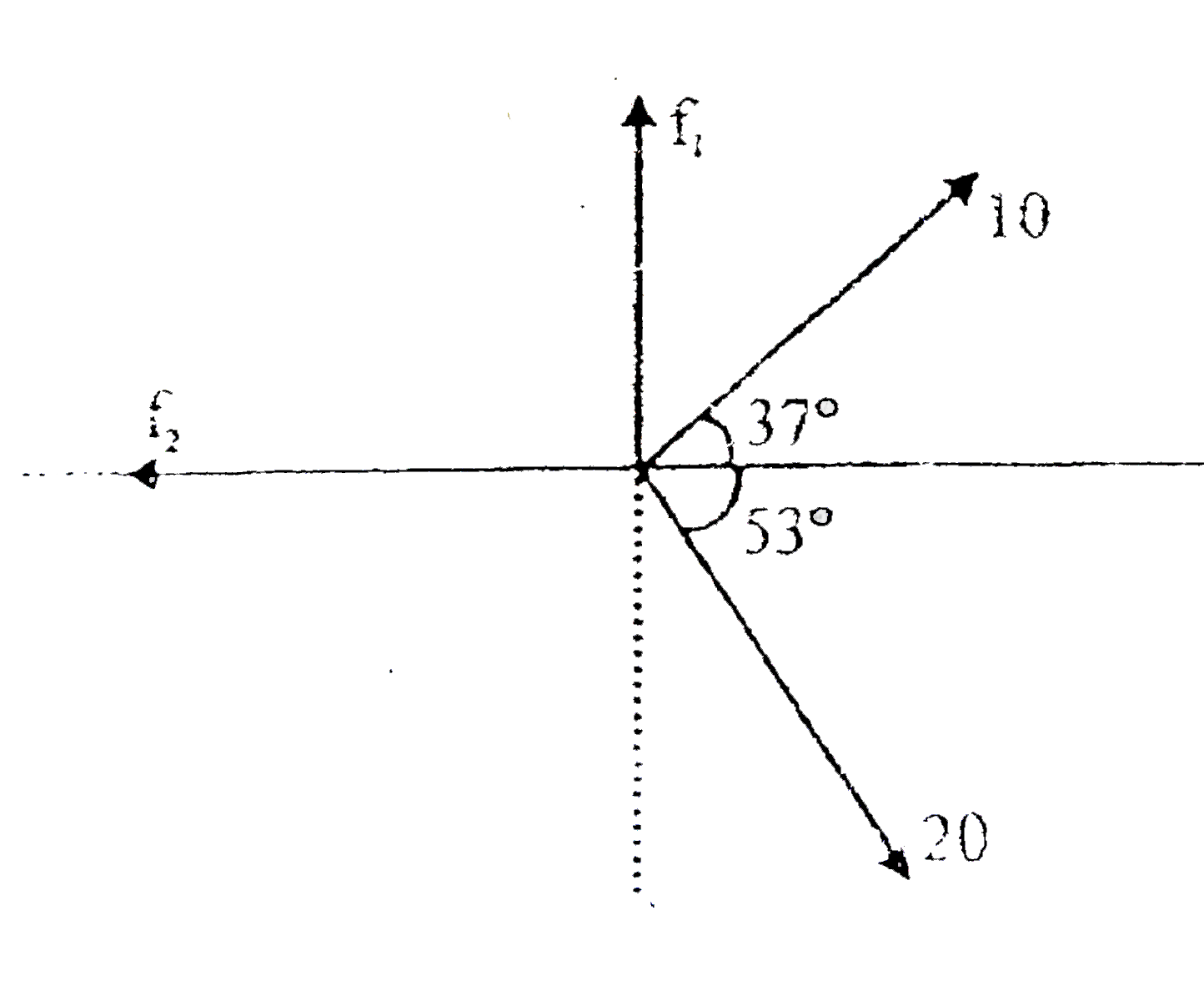
(A) Magnitude of →f1 is 10N
(B) Magnitude of →f1 is 20N
(C) Magnitude of →f2 is 10N
(D) Magnitude of →f2 is 20N
View SolutionThree concurrent force are F1,F2 and F3. Angle between F1 and F2 is 30∘and between F1 and F3 is 120∘. Under these conditions forces cannot remain in equilibrium.
At least one angle should be greater then 180∘.
View SolutionLet F1 be the magnitude of the gravitational force exerted on the Sun by Earth and F2 be the magnitude of the force exerted on Earth by the Sun. Then:
View Solution