Four forces act on a particle as shown in the figure such that net force is zero. Then consider following statement :−
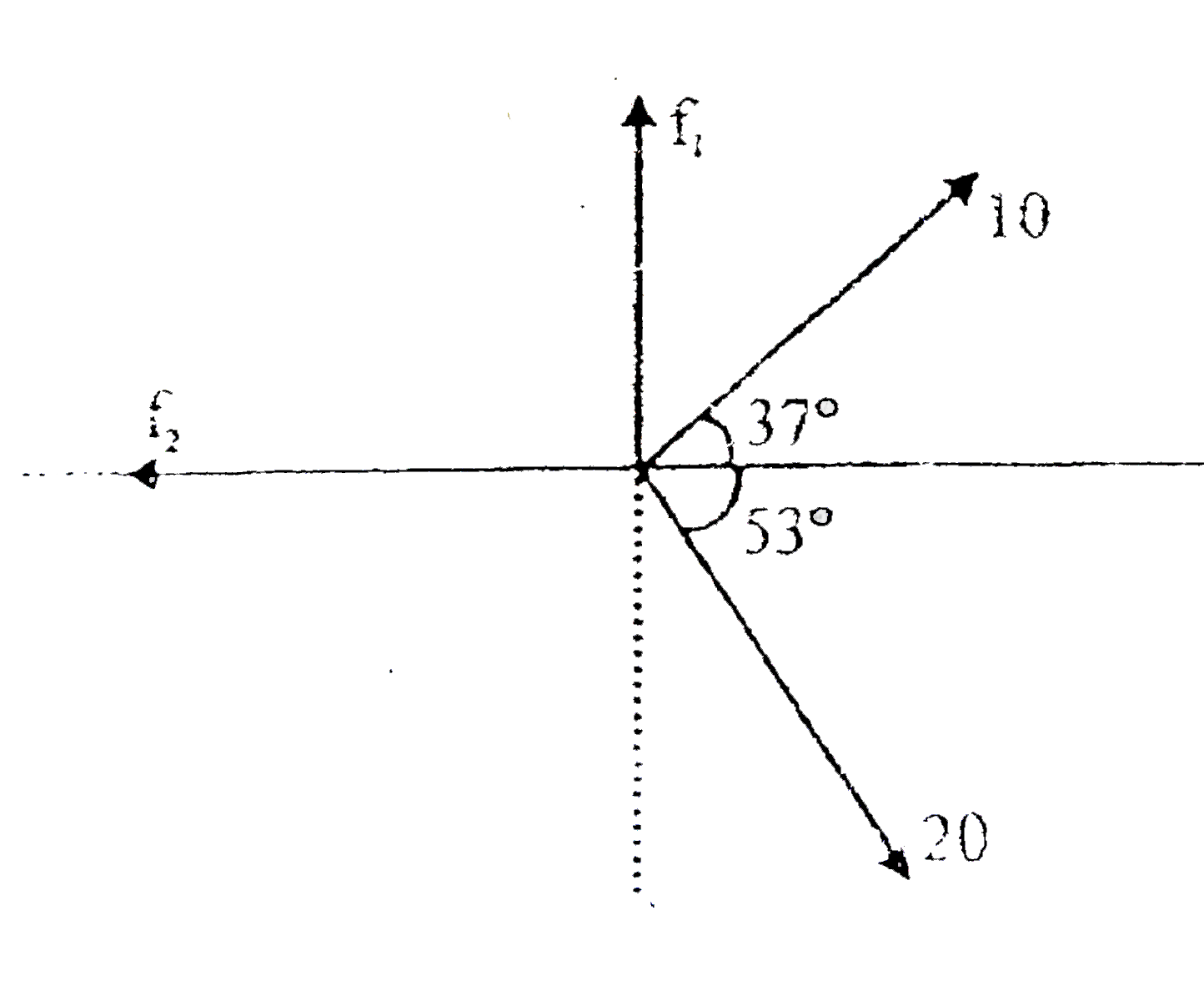
(A) Magnitude of →f1 is 10N
(B) Magnitude of →f1 is 20N
(C) Magnitude of →f2 is 10N
(D) Magnitude of →f2 is 20N
Only A
Only C
Only D
Only A & D
The correct Answer is:D
f1=−10sin37∘+20sin53∘
f2=10cos37∘+20cos53∘
Topper's Solved these Questions
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
- TEST PAPER 1BOOK - ALLENCHAPTER - TEST PAPER 1EXERCISE - PHYSICS
11 Videos
Similar Questions
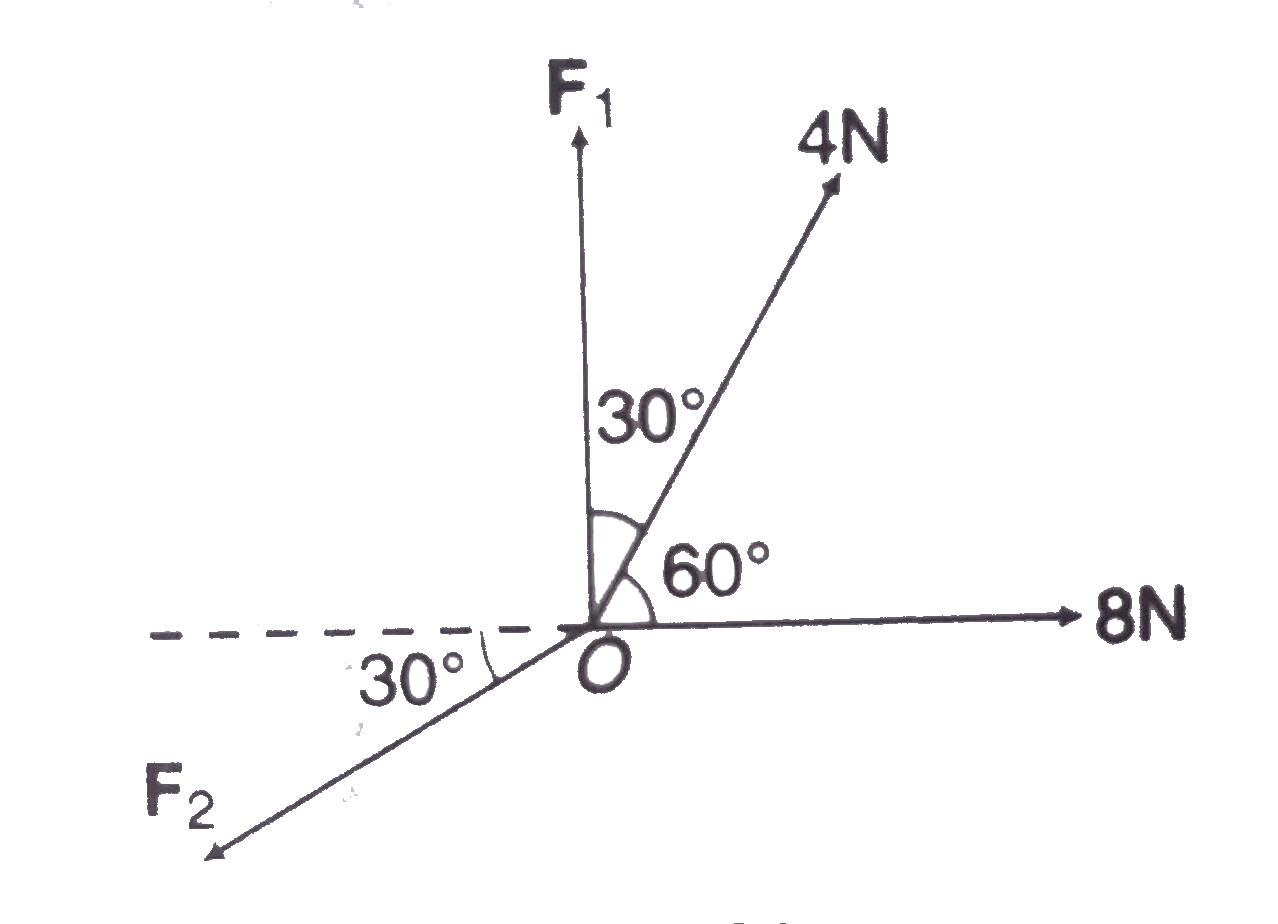
An object is in equilibrium under four concurrent forces in the direction shown in figure. Find the magnitudes of F1 and F2
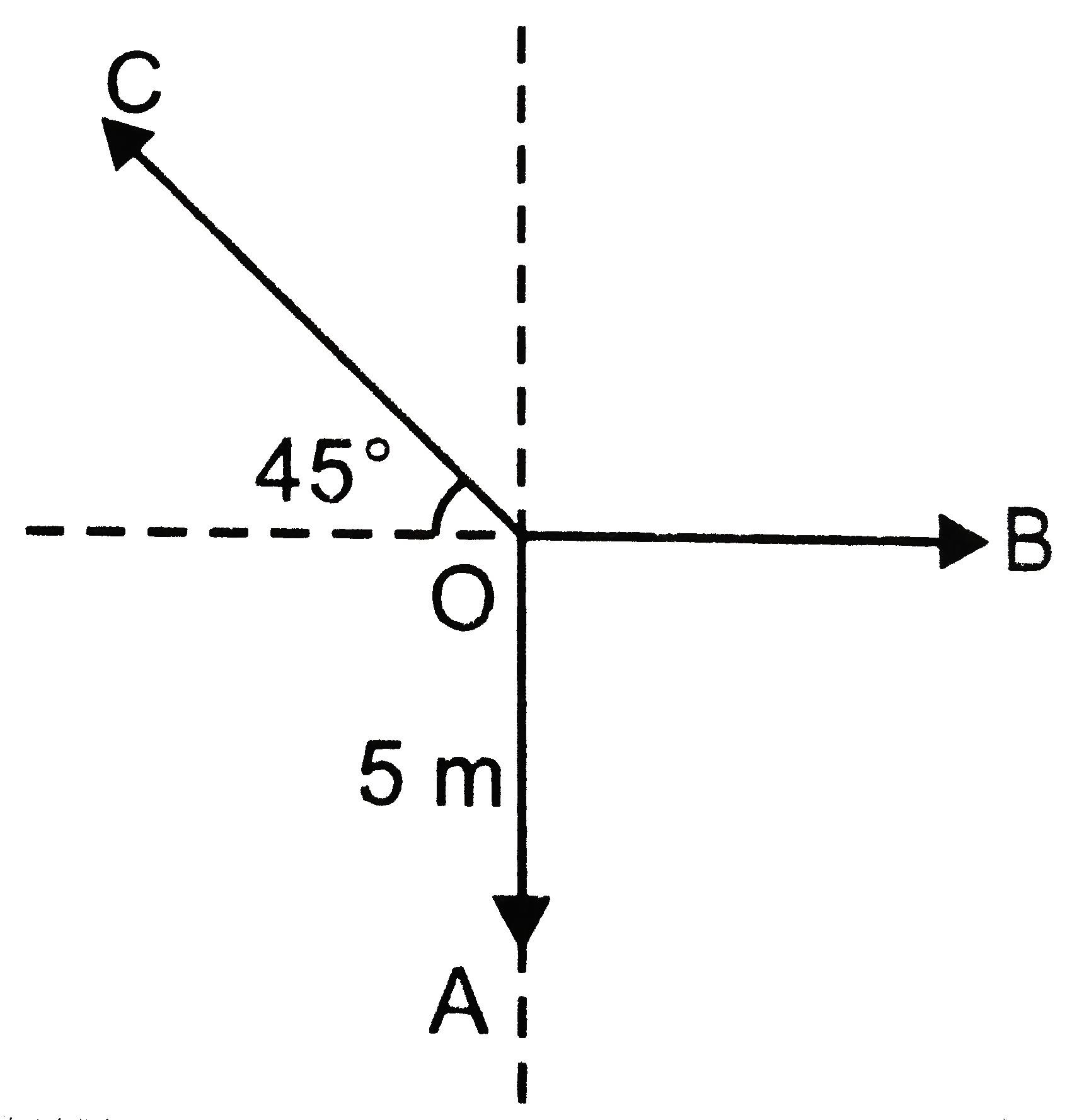
View SolutionThe sum of three vectors shown in Fig. 2 ( c) . 77. is zero .
 .
.
(a) What is the magnitude of the vector −−→OB?<br>(b)Wˆisthemagnitudeofthe→−−→r vec (OC) ?
View Solution
Knowledge Check
Two forces of mangitude F1 and F2 are acting on an object.The magnitude of the net force Fnet on the object will be in the range.
AF1≤Fnet≤F2B|F1−F2|/2≤Fnet≤(F1+F2)/2C|F1−F2|≤Fnet≤F1+F2D|F1−F2|≥Fnet|F1+F2|Six vector →a through →f have the magnitudes and direction indicated in the figure. Which of the following statements is true?
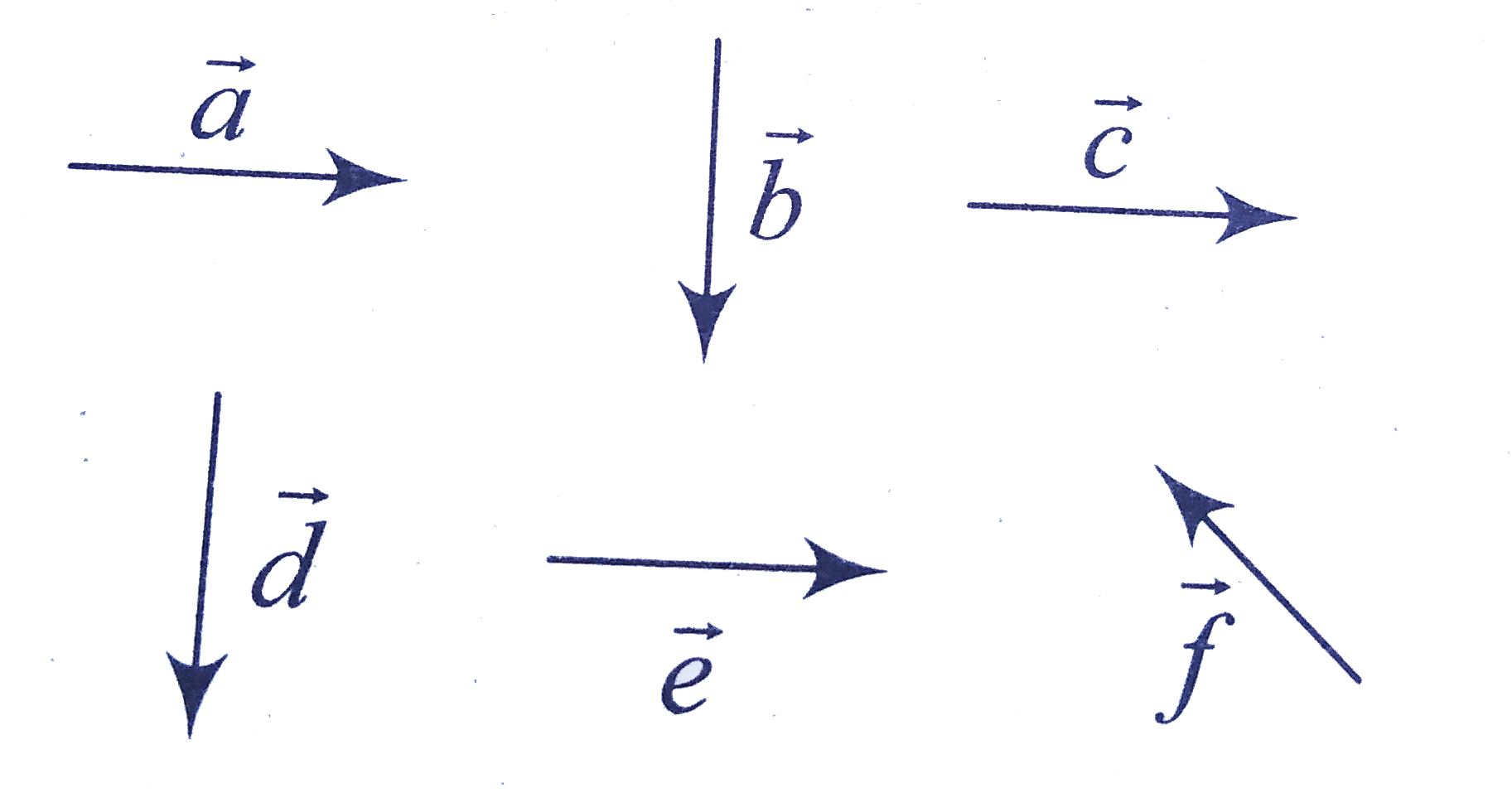 A→b+→e=→fB→b+→c=→fC→d+→c=→fD→d+→e=→f
A→b+→e=→fB→b+→c=→fC→d+→c=→fD→d+→e=→fIf →F is the force acting in a particle having position vector →r and →τ be the torque of this force about the origin, then
A→r.→τ≠0and→F.→τ=0B→r.→τ>0and→F.→τ<0C→r.→τ=0and→F.→τ=0D→r.→τ=0and→F.→τ≠0
A smooth track in the form of a quarter circle of radius 6m lies in the vertical plane. A particle moves from P1 to P2 under the action of forces →F1,→F2 and →F3 Force →F1 is 20N, Force →F2 is always 30N in magnitude. Force →F3 always acts tangentiallly to the track and is of magnitude 15N. Select the correct alternative (s) :
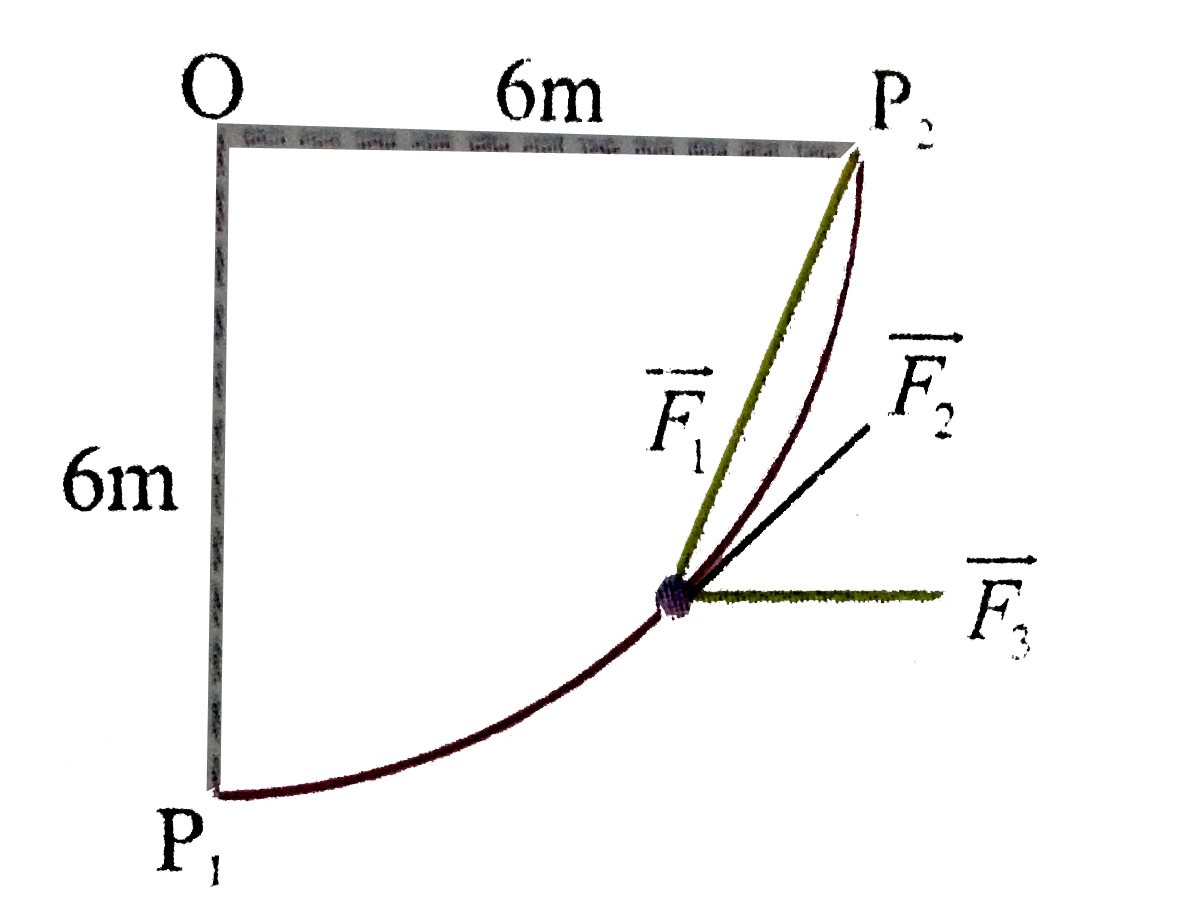 .
.
View SolutionIf →F=(5ˆi+2ˆj−4ˆk)N, the magnitude of force is
View SolutionTwo vector →Vand→V have equal magnitudes. If magnitude of →A+→B is equal to n time the magnitude of →A−→B, then angel to between →Aand→B is
View SolutionSix forces are acting on a particle. Angle between two adjacent force is 60∘. Five of the forces have magnitude F1 and the sixth has magnitude F2 . The resultant of all the forces will have magnitude of:
View SolutionThe forces each of 20N act on a body at 120∘ The magnitude and direction of resultant is
View Solution
