A man is running with a speed of 4 m/s, if he feels that rain drops are hitting him vertically with speed 3 m/s (along unit vector −ˆj) then what can be the velocity of rain:
3ˆi−4ˆj
4ˆi+3ˆj
−3ˆi−4ˆj
−4ˆi−3ˆj
The correct Answer is:D
To solve the problem, we need to analyze the situation using the concept of relative velocity. Let's break it down step by step.
Step 1: Understand the Given Information
- The man is running with a speed of 4m/s.
- He perceives the rain to be falling vertically downwards with a speed of 3m/s (along the unit vector −^j).
Step 2: Define the Velocities
- Let the velocity of the man be represented as:
→Vman=4^i
(assuming he is running in the positive x-direction).
- The velocity of the rain with respect to the man is given as:
→Vrain, man=−3^j
Step 3: Use the Relative Velocity Equation
The relative velocity of rain with respect to the man can be expressed as:
→Vrain, man=→Vrain−→Vman
From this, we can rearrange to find the velocity of the rain:
→Vrain=→Vrain, man+→Vman
Step 4: Substitute the Known Values
Substituting the known values into the equation:
→Vrain=(−3^j)+(4^i)
This simplifies to:
→Vrain=4^i−3^j
Step 5: Conclusion
Thus, the velocity of the rain is:
→Vrain=4^i−3^j
- The man is running with a speed of 4m/s.
- He perceives the rain to be falling vertically downwards with a speed of 3m/s (along the unit vector −^j).
- Let the velocity of the man be represented as:
→Vman=4^i
(assuming he is running in the positive x-direction).
- The velocity of the rain with respect to the man is given as:
→Vrain, man=−3^j
The relative velocity of rain with respect to the man can be expressed as:
→Vrain, man=→Vrain−→Vman
From this, we can rearrange to find the velocity of the rain:
→Vrain=→Vrain, man+→Vman
Substituting the known values into the equation:
→Vrain=(−3^j)+(4^i)
This simplifies to:
→Vrain=4^i−3^j
Thus, the velocity of the rain is:
→Vrain=4^i−3^j
Topper's Solved these Questions
Similar Questions
A person standing on a road has to hold his umbrella at 600 with the verticcal to keep the rain away. He throws the umbrella an starts running at 20ms−1. He finds that rain drops are hitting his head vertically. Find the speed of the rain drops wigh respect to (a) the road (b) the moving person.
View SolutionA man standing on a road has to hold his umbrella at 30∘ with the vertical to keep the rain away. He throws the umbrella and starts running at 10km/hr. He finds that rain drop are hitting his head vertically. Find the speed of rain drops with respect to (a) road (b) the moving man.
View Solution
Knowledge Check
A man running with velocity 3m/s finds that raindrops are hitting him vertically with a speed of 4m/s.
Magnitude of velocity of the raindrops isA3m/sB4m/sC5m/sD6m/sA man running with velocity 3m/s finds that raindrops are hitting him vertically with a speed of 4m/s.
Raindrops are falling at an angle θ with the vertical. Value of θ isA37∘B53∘C45∘D60∘A man running at a speed of 5 km/h finds that the rain is falling vertically. When the stops running, the finds that the rain is falling at an angle of 60∘ with the horizontal. The velocity of rain with respect to running man is
A5√3 km/hB5√32 km/hC4√35 km/hD5√3 km/h
A person standing on a road has to hold his umbrellat at 45∘ with the vertical to deep the rain away . He throws the umbtella and starts running at 30ms−1 . He find that the rain drops are hitting his head vertically. Fing the speed of the rain drops with respect to (a) road (b) the moving person.
View SolutionWhen a boy is stationary, he observes that rain drops are falling at 30∘ to vertical direction. When he starts running with speed 10ms, he observed that rain drops are falling vertically downwards. Calculate the actual velocity of rain drops.
View SolutionA man walking with a speed of 3 km/h finds the rain drops falling vertically downwards. When the man increases his speed to 6km/h he find that the rain drops are falling making an angle of 30∘ with the vertical . Find the speed of the rain drops ( in km/h)
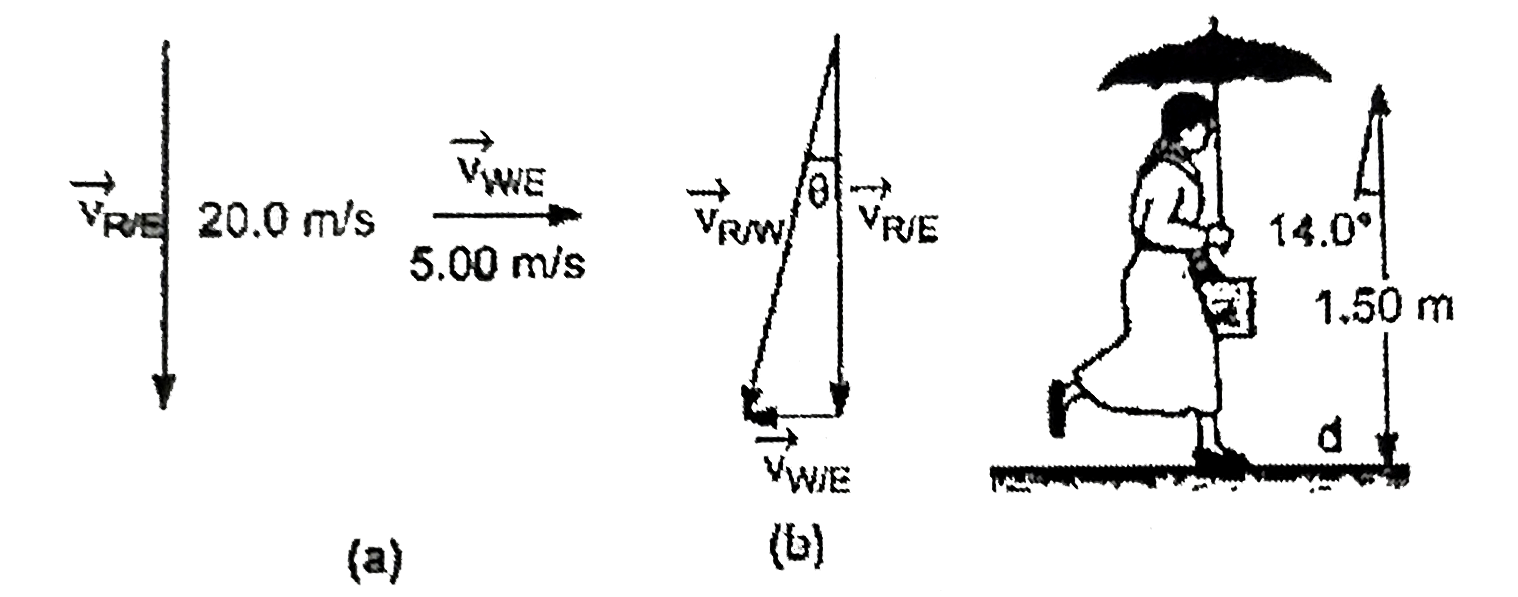
View SolutionA woman is running through rain at a speed of 5.00 m/s. Rain is falling vertically at a speed of 20.0 m/s. (a) What is the velocity of the rain relative to the woman? (b) How far in front of her would an umbrella have to extend to keep the rain off if she holds the umbrella 1.50 m above her feet?
View SolutionA man holds an umbrella at 30∘ with the vertical to keep himself dry. He, then, runs at a speed of 10ms−1, and find the rain drops to be hitting vertically. Study the following statement and find the correct options.
i. Velocity of rain w.r.t. Earth is 20ms−1
ii. Velocity of rain w.r.t. man is 10√3ms−1.
iii. Velocity of rain w.r.t. Earth is 30ms−1
iv. Velocity of rain w.r. t. man is 10√2ms−1 .
View Solution
