A rod of length L is hinged from one end. It is brought to a horizontal position and released. The angular velocity of the rod, when it is in vertical position, is
√2gL
√3gL
√g2L
√gL
To find the angular velocity of a rod of length L when it is in the vertical position after being released from the horizontal position, we can use the principle of conservation of energy. Here’s the step-by-step solution:
Step 1: Understand the Initial and Final Positions
- The rod is initially in a horizontal position and is hinged at one end. When released, it rotates downwards to a vertical position.
- The center of mass of the rod is located at L2 from the hinge.
Step 2: Calculate the Change in Potential Energy
- When the rod is horizontal, the height of the center of mass is L2.
- When the rod is vertical, the height of the center of mass is 0.
- The loss in potential energy (PE) as the rod moves from horizontal to vertical is given by:
ΔPE=mgh=mg(L2)
where m is the mass of the rod and g is the acceleration due to gravity.
Step 3: Calculate the Kinetic Energy at the Vertical Position
- The kinetic energy (KE) of the rod when it reaches the vertical position can be expressed in terms of its angular velocity ω:
KE=12Iω2
- The moment of inertia I of the rod about the hinge is:
I=13mL2
- Therefore, the kinetic energy becomes:
KE=12(13mL2)ω2=mL2ω26
Step 4: Apply Conservation of Energy
- According to the law of conservation of energy, the loss in potential energy equals the gain in kinetic energy:
ΔPE=KE
- Substituting the expressions we derived:
mg(L2)=mL2ω26
Step 5: Simplify the Equation
- Cancel m from both sides (assuming m≠0):
g(L2)=L2ω26
- Multiply both sides by 6:
3gL=L2ω2
- Divide both sides by L (assuming L≠0):
3g=Lω2
Step 6: Solve for Angular Velocity ω
- Rearranging gives:
ω2=3gL
- Taking the square root:
ω=√3gL
Final Answer
The angular velocity of the rod when it is in the vertical position is:
ω=√3gL
---
- The rod is initially in a horizontal position and is hinged at one end. When released, it rotates downwards to a vertical position.
- The center of mass of the rod is located at L2 from the hinge.
- When the rod is horizontal, the height of the center of mass is L2.
- When the rod is vertical, the height of the center of mass is 0.
- The loss in potential energy (PE) as the rod moves from horizontal to vertical is given by:
ΔPE=mgh=mg(L2)
where m is the mass of the rod and g is the acceleration due to gravity.
- The kinetic energy (KE) of the rod when it reaches the vertical position can be expressed in terms of its angular velocity ω:
KE=12Iω2
- The moment of inertia I of the rod about the hinge is:
I=13mL2
- Therefore, the kinetic energy becomes:
KE=12(13mL2)ω2=mL2ω26
- According to the law of conservation of energy, the loss in potential energy equals the gain in kinetic energy:
ΔPE=KE
- Substituting the expressions we derived:
mg(L2)=mL2ω26
- Cancel m from both sides (assuming m≠0):
g(L2)=L2ω26
- Multiply both sides by 6:
3gL=L2ω2
- Divide both sides by L (assuming L≠0):
3g=Lω2
- Rearranging gives:
ω2=3gL
- Taking the square root:
ω=√3gL
The angular velocity of the rod when it is in the vertical position is:
ω=√3gL
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - BITSAT GUIDECHAPTER - ROTATIONAL MOTIONEXERCISE - BITSAT Archives
9 Videos
- NEWTONS LAWS OF MOTION AND FRICTIONBOOK - BITSAT GUIDECHAPTER - NEWTONS LAWS OF MOTION AND FRICTIONEXERCISE - BITSAT Archives
23 Videos
- SCALARS AND VECTORSBOOK - BITSAT GUIDECHAPTER - SCALARS AND VECTORSEXERCISE - All Questions
34 Videos
Similar Questions
A rod of length L is hinged from one end. It is brought to horizontal position and released. The angular velocity of the rod when it is in vertical position. Is
View SolutionA rod of length L is hinged from one end. It is brought to the horizontal position and released. The angular velocity of the rod when it is in the vertical position is √Kg/L. Then find the value of K.
View Solution
Knowledge Check
A rod of length L is hinged from one end. It is brought to a horizontal position and released. The angular velocity of the rod, When it is in verticle position is
A√2gLB√3gLC√g2LD√gLA uniform thin rod of length l and mass m is hinged at a distance l/4 from one of the end and released from horizontal position as shown in Fig. The angular velocity of the rod as it passes the vertical position is
 A2√5g7lB2√6g7lC√3g7lD2√gl
A2√5g7lB2√6g7lC√3g7lD2√glIn the above problem, if the rod is released from horizontal position, the angular velocity of the rod as it passes the vertical position is (l=length fo rod)
A√12g5lB√2g3lC√3glD√3g7l
A rigid rod of mass m & length l is pivoted at one of its ends. If it is released from its horizontal position, find the speed of the centre of mass of the rod when it becomes vertical.
View SolutionConsider a uniform rod of mass M and length L is hinged at one end as shown. Initially the rod is vertical, it is slightly pushed and released. Find the angular velocity of rod and liner velocity of its one end when the rod becomes horizontal. Also calculate angular velocity of rod when it has rotated by an angle θ(θ<90∘).
View SolutionA rod is released from the horizontal position as shown. It is free to rotate about hinge. Find angular velocity of rod when it become vertical?
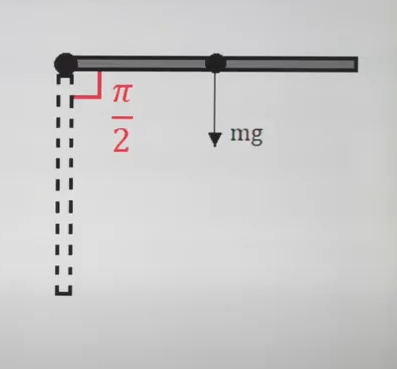
View SolutionA thin bar of mass M and length L is free to rotate about a fixed horizontal axis through a point at its end. The bar is brought to a horizontal position and then released. The axis is perpendicular to the rod. The angular velocity when it reaches the lowest point is
View SolutionTwo particles each of mass m are attached at end points of a massless rod AB of length l. Rod is hinged at point C as shown. Rod is released from rest from horizontal position. At the instant when rod reaches its vertical position as shown, which of the following is / are correct:
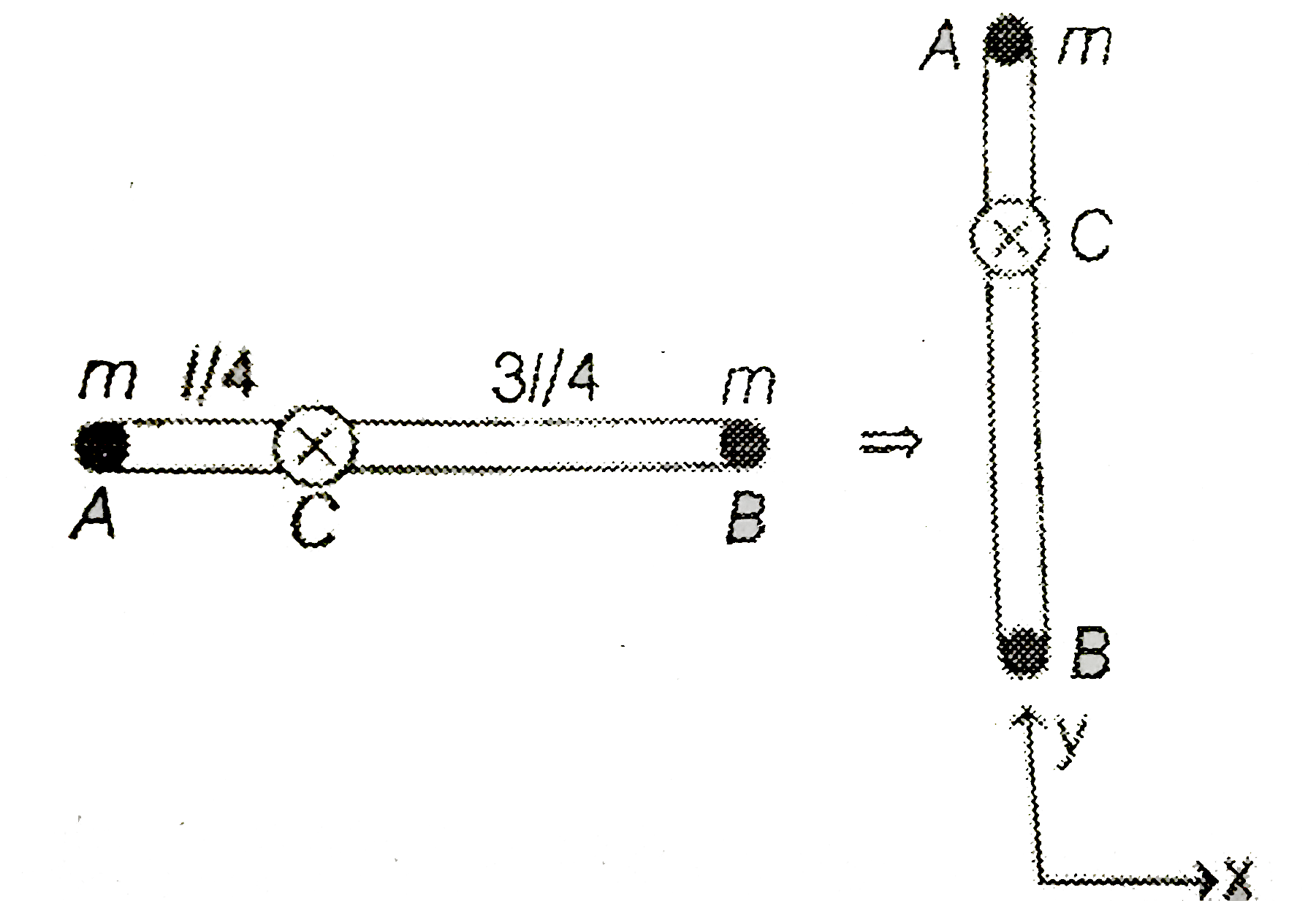
View Solution
