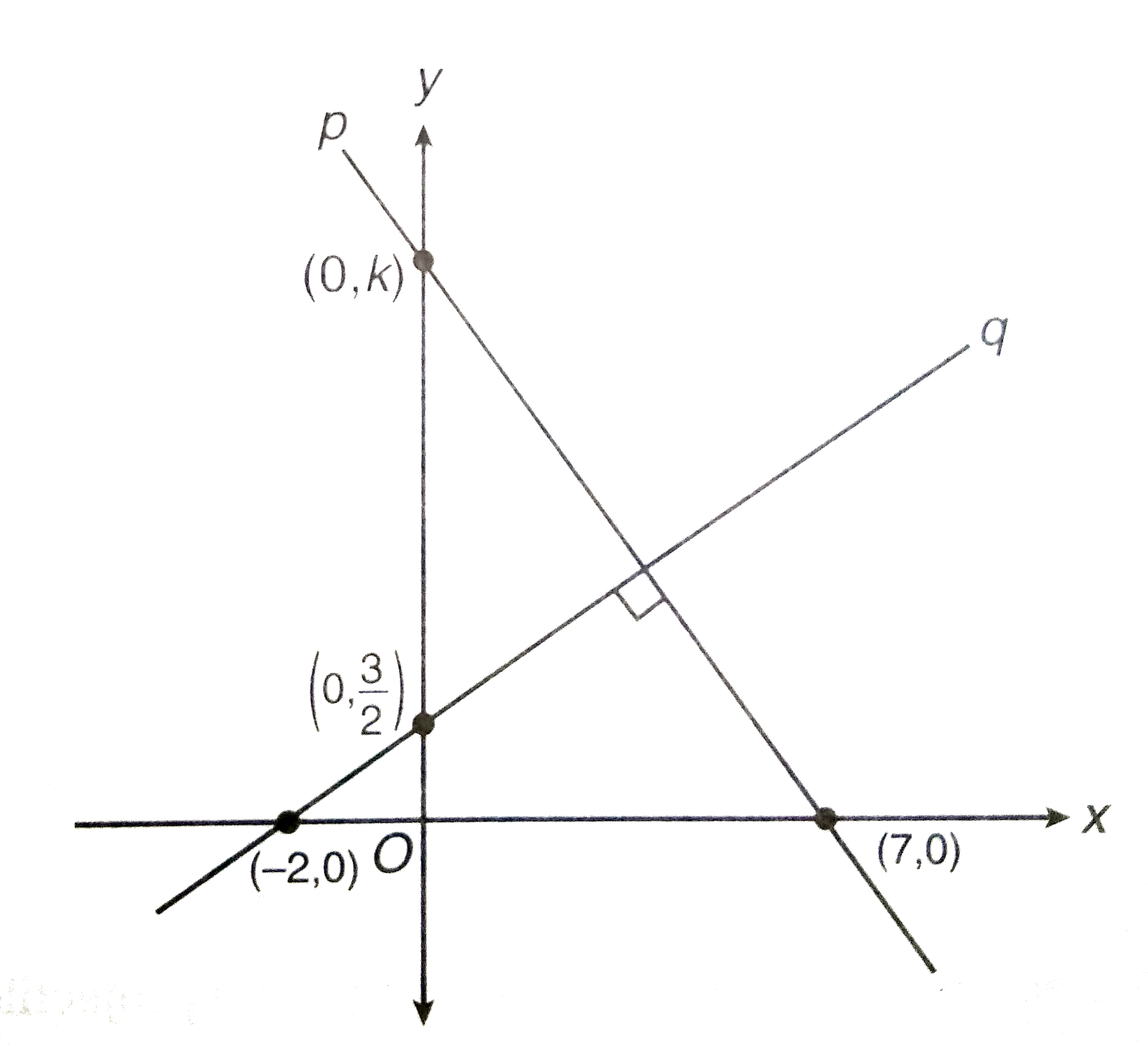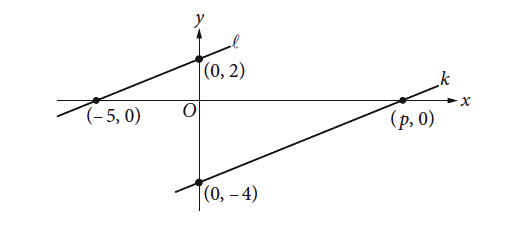
In the xy-plane above, line l is parallel to line k. What is the value of p ?
A
4
B
5
C
8
D
10
Video Solution
Text Solution
Choice D is correct. Since lines l and k are parallel, the lines have the same slope. Line l passes through the point (-5, 0) and (0, 2), so its slope is 0−2−5−0, which is 25. The slope of line k must also be 25. Since line k has slope 25 and passes through the points (0, -4) and (p, 0), it follows that −4−00−p=25,or4p=25. Multiplying each side of 4p=25 by 5p gives 20 = 2p, and therefore, p = 10.
Choices a, B, and C are incorrect and may result from conceptual or calculation errors.
|
Similar Questions
Knowledge Check
If the line y+2x+3=0 is parallel to the line 2y-px-4=0 , what is the value of p ?
A−4B−2C2D4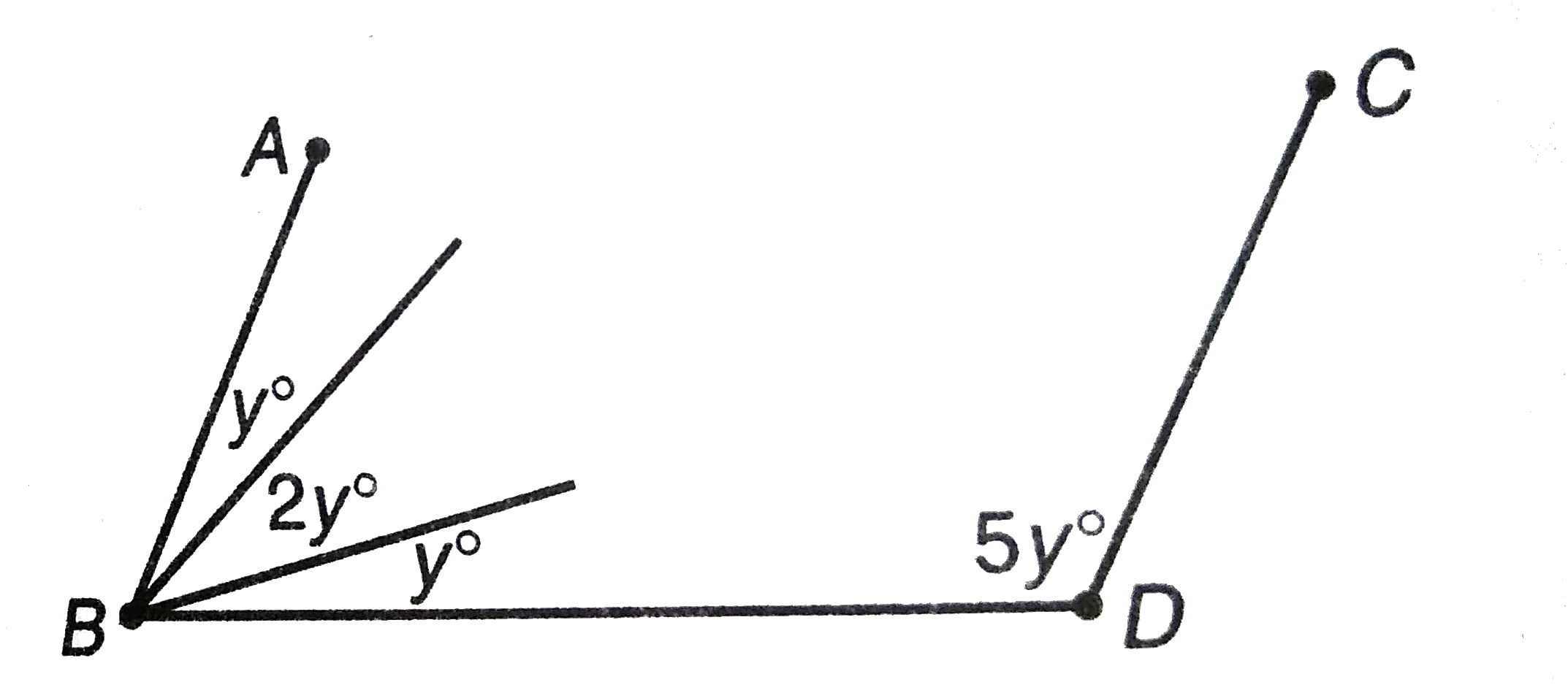
In the figure above, if line segment AB is parallel to line segment CD, what is the value of y?A12B15C18D20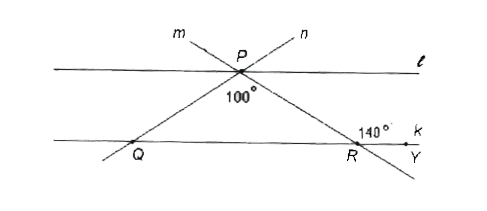
In the figure above, line l is parallel to line k. Transversals m and n intersect at point P on l and intersect k at point R and Q, respectively. Point Y is on k, the measure of ∠PRY is 140∘, and the measures of ∠QPR is 100∘. How many of the angles formed by rays l, k, m, and n have measure 40∘?A4B6C8D10
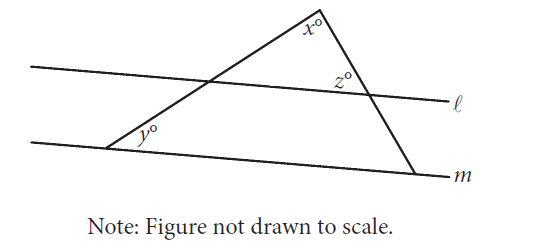
In the figure above, lines l and m are parallel, y = 20, and z = 60. What is the value of x ?
View SolutionLine p contains the points (−1,8)and(9,k). If line p is parallel to line q whose equation of 3x+4y=7. What is the value of k?
View Solution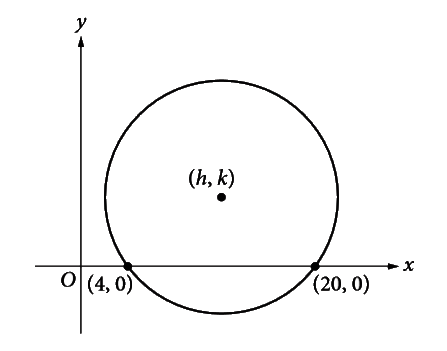
In the xy-plane above, the circle has center (h, k) and radius 10. What is the value of k ?
View SolutionWhere line XY is parallel to line QU.
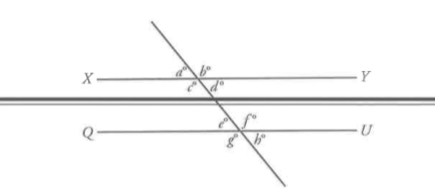
If a+e=150, find f.
View Solution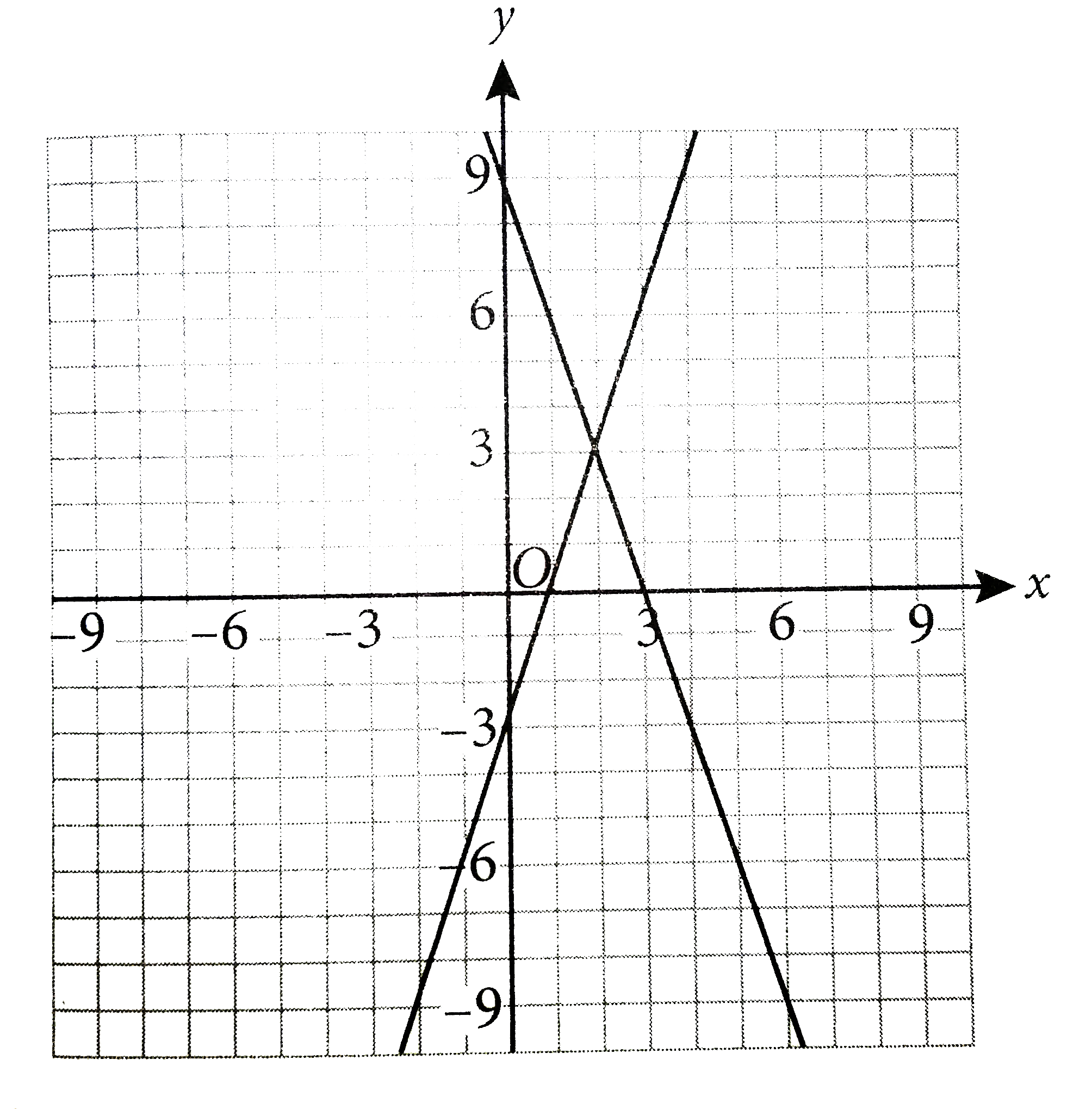
The lines graphed in the xy-plane above represent a system of two linear equations. What is the solution (x,y) to the system?
View Solution