A charge of 8 mC is located at the origin. Calculate the work done in taking a small charge of −2×10−9 from a point P(0,0,3cm) to a point Q (0,4cm,0) via a point R(0,6cm,9cm).
To solve the problem of calculating the work done in moving a charge from point P to point Q via point R, we will follow these steps:
Step 1: Understand the Configuration
We have a charge Q=8mC=8×10−3C located at the origin (0,0,0). We need to move a charge q=−2×10−9C from point P (0,0,3 cm) to point Q (0,4 cm,0) via point R (0,6 cm,9 cm).
Step 2: Calculate the Electric Potential at Points P and Q
The electric potential V due to a point charge is given by the formula:
V=k⋅Qr
where k=9×109N m2/C2 is Coulomb's constant, and r is the distance from the charge to the point where the potential is being calculated.
For Point P (0,0,3 cm):
The distance r1 from the origin to point P is:
r1=3cm=0.03m
Thus, the potential at P is:
VP=9×109⋅8×10−30.03=72×1060.03=2.4×109V
For Point Q (0,4 cm,0):
The distance r2 from the origin to point Q is:
r2=√02+0.042+02=0.04m
Thus, the potential at Q is:
VQ=9×109⋅8×10−30.04=72×1060.04=1.8×109V
Step 3: Calculate the Work Done
The work done W in moving the charge q from point P to point Q is given by:
W=q(VQ−VP)
Substituting the values:
W=−2×10−9⋅(1.8×109−2.4×109)
W=−2×10−9⋅(−0.6×109)
W=1.2J
Final Answer
The work done in moving the charge from point P to point Q via point R is 1.2J.
---
We have a charge Q=8mC=8×10−3C located at the origin (0,0,0). We need to move a charge q=−2×10−9C from point P (0,0,3 cm) to point Q (0,4 cm,0) via point R (0,6 cm,9 cm).
The electric potential V due to a point charge is given by the formula:
V=k⋅Qr
where k=9×109N m2/C2 is Coulomb's constant, and r is the distance from the charge to the point where the potential is being calculated.
The distance r1 from the origin to point P is:
r1=3cm=0.03m
Thus, the potential at P is:
VP=9×109⋅8×10−30.03=72×1060.03=2.4×109V
The distance r2 from the origin to point Q is:
r2=√02+0.042+02=0.04m
Thus, the potential at Q is:
VQ=9×109⋅8×10−30.04=72×1060.04=1.8×109V
The work done W in moving the charge q from point P to point Q is given by:
W=q(VQ−VP)
Substituting the values:
W=−2×10−9⋅(1.8×109−2.4×109)
W=−2×10−9⋅(−0.6×109)
W=1.2J
The work done in moving the charge from point P to point Q via point R is 1.2J.
Topper's Solved these Questions
- ELECTRIC POTENTIAL AND ELECTRIC FLUXBOOK - SL ARORACHAPTER - ELECTRIC POTENTIAL AND ELECTRIC FLUXEXERCISE - Problem
15 Videos
- ELECTRIC POTENTIAL AND ELECTRIC FLUXBOOK - SL ARORACHAPTER - ELECTRIC POTENTIAL AND ELECTRIC FLUXEXERCISE - Problems for self practive
61 Videos
- CURRENT ELECTRICITYBOOK - SL ARORACHAPTER - CURRENT ELECTRICITYEXERCISE - Problems For Self
101 Videos
- ELECTRICAL INSTRUMENTSBOOK - SL ARORACHAPTER - ELECTRICAL INSTRUMENTSEXERCISE - Problem for self practice
81 Videos
Similar Questions
A charge of 8 mC is located at the origin. Calculate the work done in taking a small charge of −2×10−9C from a point P (0,0,3 cm) to a point Q (0,4 cm, 0) via a point R (0,6 cm, 9cm).
View SolutionA charge of 8 mC is located at the origin. Calculate the work done in taking a small charge of −2×10−9C from a point P(0,0,3 cm) to a point Q10,4 cm, 0) via a point R(0,6 cm, 9 cm).
View Solution
Knowledge Check
Calculate the work done in taking a charge −2×10−9C from A to B via C (in diagram )
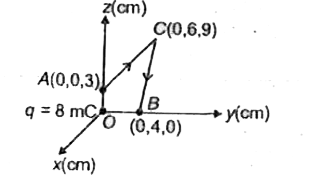 A0.2 jouleB1.2 jouleC2.2 jouleDzero
A0.2 jouleB1.2 jouleC2.2 jouleDzeroAs per this diagram a point charge +q is placed at the origin O. Work done in taking another point charge −Q from the point A(0,a) to another point B(a,0) along the staight path AB is:
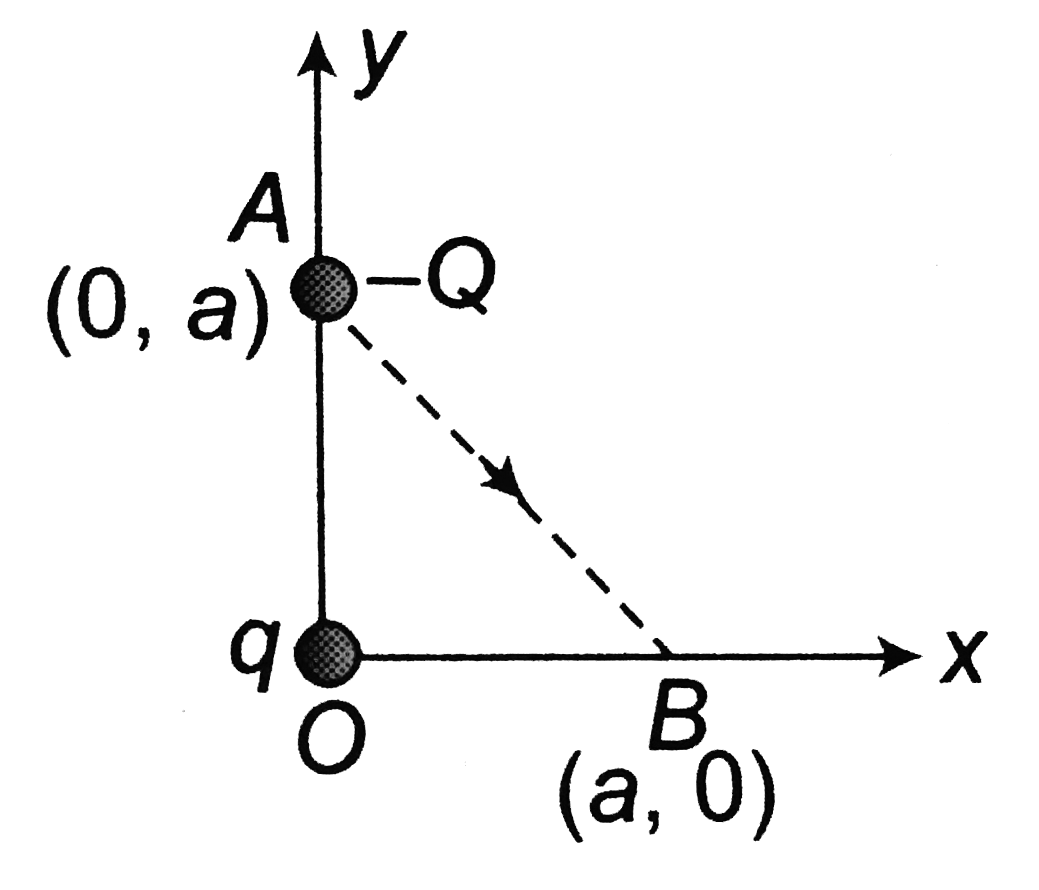 A(−qQ4πε01a2)√2aBzeroC(qQ4πε01a2)1√2D(qQ4πε01a2)1√2a
A(−qQ4πε01a2)√2aBzeroC(qQ4πε01a2)1√2D(qQ4πε01a2)1√2aAs per this diagram a point charge +q is placed at the origin O. Work done in taking another point charge −Q from the point A(0,a) to another point B(a,0) along the staight path AB is:
 AzeroB(−qQ/4πε0)(1/a2)√2aC(qQ/4πε0)(1/a2).a/√2D(qQ/4πε0)(1/a2)√2a
AzeroB(−qQ/4πε0)(1/a2)√2aC(qQ/4πε0)(1/a2).a/√2D(qQ/4πε0)(1/a2)√2a
(a) Calculate the potential at a point P due to a charge of 4×10−7C located 9 cm away.
(b) Hence obtain the work done in bringing a charge of 2×10−9C from infinity to the point P. Does the answer depend on the path along which the charge is brought ?
View SolutionA charge of 0.5 nC is placed at the centre of an equilateral triangle of side 10 cm, calculate the work done in bringing a charge of 0.1 nC from infinity to any vertices of the triangle.
View SolutionTwo charges −qand+q are located at points A(0,0,−a)andB(0,0+a). How much work is done in moving a test charge from point (b,0,0) to Q(−b,0,0) ?
View Solution(i) Can two equpotential surfaces intersect each other ? Give reason.
(ii) Two charges +qand−q are located at points A (0,0,−2)andB(0,0,2) respectively. How much work will be done in moving a test charge from point P(4,0,0) to (−5,0,0) ?
View SolutionTwo charges - q and + q are located at points A(0, 0, -a) and B(0, 0, +a) respectively. How much work is done in moving a test charge from point P(7,0,0) to Q(-3,0,0) ?
View Solution