Two particles A and B are located in x−y plane at points (0,0) and (0,4m). They simultaneoulsy start moving with velocities vA=2ˆjm/s and vB=2ˆim/s. Select the correct alternative(s)
the distance between them is constant
The distance between them first decreases and then increases
the shortest distance between them is 2√2m
Time after which they are at minimum distances is 1s
The correct Answer is:B, C, D
Step by step video, text & image solution for Two particles A and B are located in x-y plane at points (0,0) and (0,4m). They simultaneoulsy start moving with velocities v_(A)=2hatjm//s and v_(B)=2hatim//s. Select the correct alternative(s) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - COMPREHENSION_TYPE19videos
KINEMATICS 1
- BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - MATCH THE COLUMN10videos
KINEMATICS 1
- BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE15videos
KINEMATICS 1
- BOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE11videos
KINEMATICS
- BOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery39videos
LAWS OF MOTION
Similar Questions
Two particles A and B located at points (0,−10√3) and (0,0) in xy plane. They start moving simultaneously at time t=0 with constant velocities →VA=5ˆim/s and →VB=−5√3ˆjm/s, respectively. Time when they are closest to each other is found to be K/2 second. Find K. All distance are given in meter.
View SolutionTwo particles A and B are projected simultaneously in the directins shown in figure with velocities vA=20m/sandvB=10m/s respectively. They collide in air after 12 s. Find
(a) the angle θ
(b) the distance x.
. View Solution
Knowledge Check
- Question 1 - Select One
Two particles A and B locat at (0,0) and (4,4) respectively start moving simu lataneou ly with velocities vA=−4ˆi and VB=−4ˆj:
Athe shortest distance between them is 4√2mBthe shortest distance between them first decreases and then increasesCthe distance between them increases from the beginningDthe magnitude of relative velocity of A w.r.t. B is 4m/s - Question 2 - Select One
Two projectile A and B located at (0,0) and (4.4) respectively start moving simu ltaneou lsy with velocities VA=−4ˆi and VB=4ˆj:
Athe shortest distance between them is 4√2mBthe shortest distance between them first decreases and then increasesCthe distance between them increases from the beginningDthe magnitude of relative velocity of A w.r.t. B is 4m/s - Question 3 - Select One or More
Acceleration of a particle which is at rest at x=0 is →a=(4−2x)ˆi. Select the correct alternative (s).
AMaximum speed of the particle is 4 unitsBParticle further comes to rest at x=4CParticle oscillates about x=2DParticle will continuously acceleration along the x-axis.
Two particles A and B start at O travel in opposite directions along the circular path at constant speed vA=0.7m/s and vB=1.5m/s respectively. Determine the time when they collide and the magnitude of the acceleration of B just before this happening. (radius=5m)
View SolutionTwo particles A and B are moving in the x−y plane such that their velocity components are vx=1m/s,vy=1√3m/s (for A) and vx=2m/s,vy=2m/s (for B). If both the particles start moving from the same point, what is the angle between their paths ?
View SolutionTwo particles A and B are projected simultaneously in the direction shown in the figure with velocities VA=20m/s and VB=10m/s respectively. If they collide in air after 1/2 sec. then
View SolutionTwo particles start moving simultaneously from points (0, 0) and (1, 0) respectively in the OXY plane with uniform velocities v1 and v2 as shown in the figure. It is found that they collide. Then
View SolutionTwo particles A and B are located at point (0m,50m) at t=0. The particle A moves withy a constant velocity and the particle B moves on a circle of radius 50 m having centre at origin (0,0) as shown in figure. The pasrticle B has a constant speed 5πm/s. What will be the velocity of particle A so that both the pasrticles reach at point (50m,0m) simultaneously.
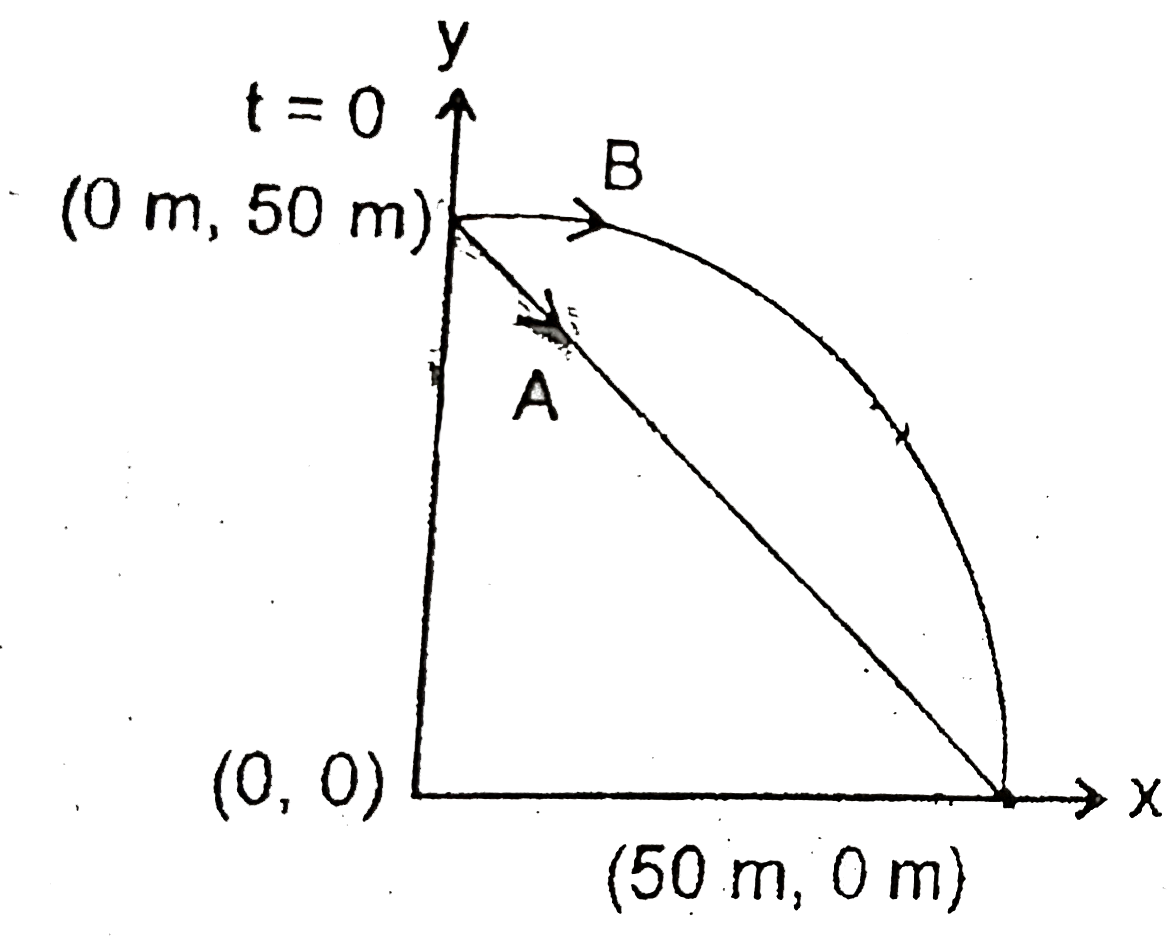View Solution