A plane mirror is placed at origin parallel of y-axis, facing the positive x-axis. An object starts from (2m, 0, 0) with a velocity of (2ˆi+2ˆj)m/s. The relative velocity of image with respect to object is along
positive x-axis
negative x-axis
positive y-axis
negative y-axis
The correct Answer is:B
To solve the problem, we need to analyze the motion of the object and its image in relation to the plane mirror. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the setup
- The plane mirror is located at the origin (0, 0) and is parallel to the y-axis, facing the positive x-axis.
- The object starts at the point (2m, 0, 0) with a velocity of vo=(2^i+2^j)m/s.
Step 2: Determine the position of the image
- The image of the object in a plane mirror is located at an equal distance behind the mirror. Since the object is at (2, 0), the image will be at (-2, 0).
Step 3: Find the velocity of the image
- The velocity of the image is equal in magnitude but opposite in direction to the velocity of the object. Therefore, if the object has a velocity vo=(2^i+2^j), the velocity of the image vi will be:
vi=−vo=(−2^i−2^j)m/s
Step 4: Calculate the relative velocity of the image with respect to the object
- The relative velocity vrel of the image with respect to the object is given by:
vrel=vi−vo
Substituting the values:
vrel=(−2^i−2^j)−(2^i+2^j)=−4^i−4^j
Step 5: Determine the direction of the relative velocity
- The relative velocity vrel=−4^i−4^j indicates that the relative velocity is directed towards the negative x-axis and negative y-axis.
Conclusion
- The relative velocity of the image with respect to the object is directed along the line that makes a 45-degree angle with the negative x-axis, pointing towards the third quadrant.
Final Answer
The relative velocity of the image with respect to the object is along the direction of −^i−^j.
---
- The plane mirror is located at the origin (0, 0) and is parallel to the y-axis, facing the positive x-axis.
- The object starts at the point (2m, 0, 0) with a velocity of vo=(2^i+2^j)m/s.
- The image of the object in a plane mirror is located at an equal distance behind the mirror. Since the object is at (2, 0), the image will be at (-2, 0).
- The velocity of the image is equal in magnitude but opposite in direction to the velocity of the object. Therefore, if the object has a velocity vo=(2^i+2^j), the velocity of the image vi will be:
vi=−vo=(−2^i−2^j)m/s
- The relative velocity vrel of the image with respect to the object is given by:
vrel=vi−vo
Substituting the values:
vrel=(−2^i−2^j)−(2^i+2^j)=−4^i−4^j
- The relative velocity vrel=−4^i−4^j indicates that the relative velocity is directed towards the negative x-axis and negative y-axis.
- The relative velocity of the image with respect to the object is directed along the line that makes a 45-degree angle with the negative x-axis, pointing towards the third quadrant.
The relative velocity of the image with respect to the object is along the direction of −^i−^j.
Topper's Solved these Questions
- RAY OPTICSBook:DC PANDEYChapter:RAY OPTICSExercise:B. More than one option is correct
Explore 20 Videos
- RAY OPTICSBook:DC PANDEYChapter:RAY OPTICSExercise:C. comprehension type question
Explore 14 Videos
- RAY OPTICSBook:DC PANDEYChapter:RAY OPTICSExercise:Integer type q.
Explore 15 Videos
- PROPERTIES OF MATTERBook:DC PANDEYChapter:PROPERTIES OF MATTERExercise:Integer
Explore 8 Videos
- ROTATIONBook:DC PANDEYChapter:ROTATIONExercise:(C) Chapter Exercises
Explore 39 Videos
Similar Questions
An object is placed at (0, 0) and a plane mirror is placed,inclined 30° with the x axis.
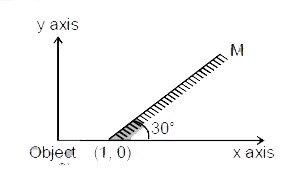
(a) Find the position of image.
(B) If the object starts moving with velocity 1ˆim/s and the mirror is fixed find the velocity of image.
View SolutionA point object is placed (0,0) and a plane mirror 'M' is placed, inclined 30∘ with the x axis.
(a) Find the position of image.
(b) If the object starts moving with velocity 1ˆim/s and the mirror is fixed find th e velocity of image.
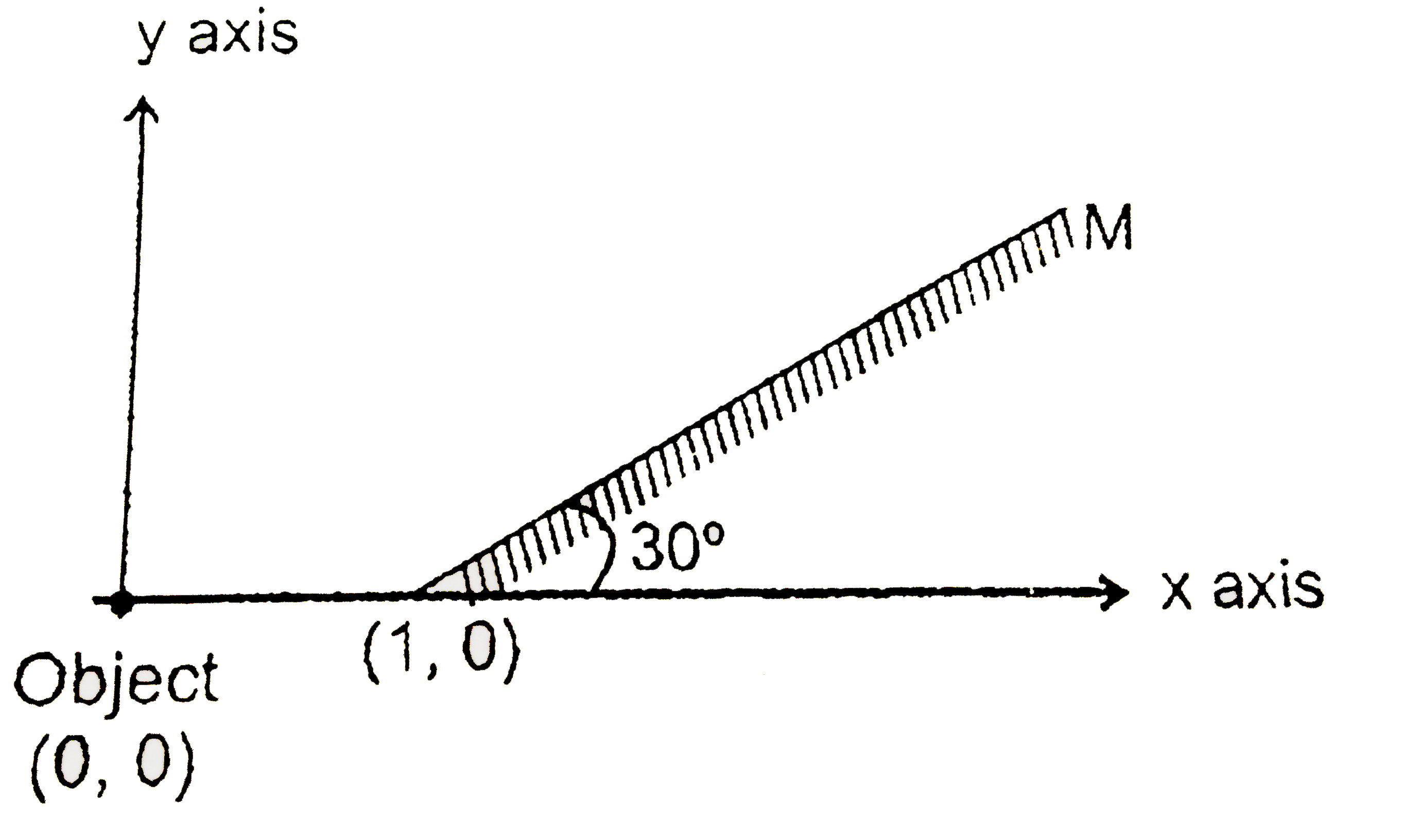
View Solution
Knowledge Check
A plane mirror is placed along the x-axis facing negative y-axis. The mirror is fixed, A point object is moving with 3ˆi+4ˆj in front of the plane mirror. The relative velocity of image with respect to its object is
 A−8ˆjB8ˆjC3ˆi−4ˆjD−6ˆi
A−8ˆjB8ˆjC3ˆi−4ˆjD−6ˆiA plane mirror placed at the origin has ˆi as the normal vector to its reflecting surface. The mirror beings to translate with a velocity ˆi+ˆj+ˆk. At the same time an object which was initially at ˆi+ˆj starts moving with a velocity (ˆi+ˆj)m/s Now choose the correct options.
AInitial position of the image will be −ˆi+ˆjBThe velocity of the image will be ˆi+ˆjCThe velocity of the imahe relative to the object will be zeroDThe velocity of the image relative to the mirror will be −ˆkThe velocity of a projectile at the initial point A is (2ˆi+3ˆj)m/s.Its velocity (in m//s) at point B is
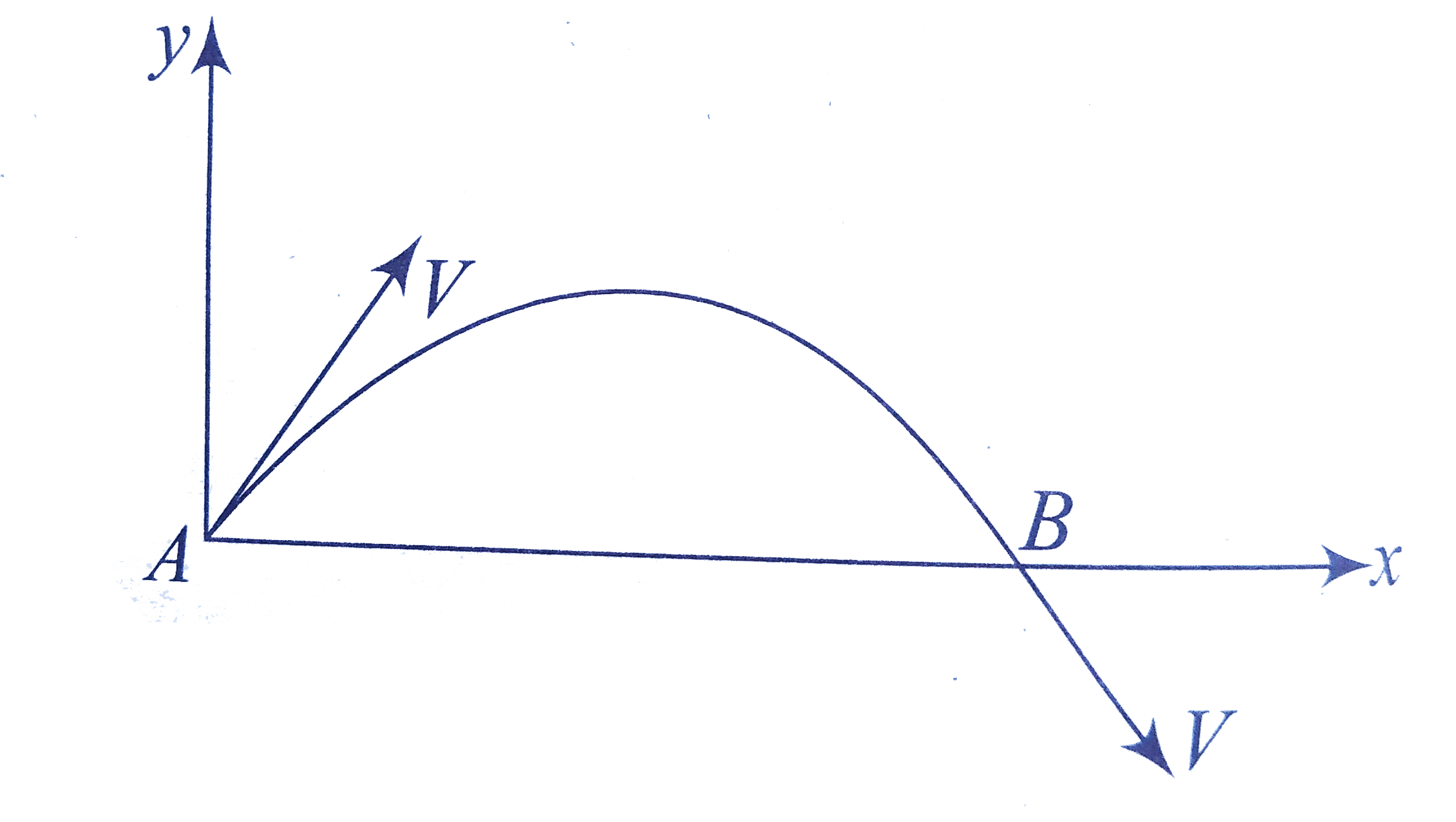 A−2ˆi−3ˆjB−2ˆi+3ˆjC2ˆi−3ˆjD2ˆi+3ˆj
A−2ˆi−3ˆjB−2ˆi+3ˆjC2ˆi−3ˆjD2ˆi+3ˆj
Velocity of a swimmer with respect to a river is (ˆi+4ˆj)ms and velocity of the river flow is (2ˆj)ms then velocity of swimmer with respect to ground is (in m/s)
View SolutionVelocity of mass 'A' of 2kg is VA=(3ˆi+2ˆj)ms and velocity of mass 'B' of 4kg is VB=3ˆjms .Then find the velocity of mass B with respect to velocity of center of mass.
View SolutionA plane mirror is moving with velocity 4ˆi+5ˆj+8ˆk. A point object in front of the mirror moves with a velocity 3ˆi+4ˆj+5ˆk. Here ˆk is along the normal to the plane mirror and facing towards the object. The velocity fo the image is
View SolutionA plane mirror is moving with velocity −2ˆi−3ˆj+4ˆk. A point object in front of the mirror move with a velocity −3ˆi+4ˆj−4ˆk.
There ˆj is along the normal to the plane mirror and facing towards the object. Find velocity of image.
View SolutionA plane mirror is moving with velocity 4(ˆi)+5(ˆj)+8(ˆk). A point object in front of the mirror moves with a velocity 3(ˆi)+4(ˆj)+5(ˆk). Here, ˆk is along the normal to the plane mirror and facing towards the object. The velocity of the image is
View Solution