One mole of an ideal monatomic gas undergoes the following four reversible processes :
Step 1 – it is first compressed adiabatically from volume 8.0m3 to 1.0m3.
Step 2 – then expanded isothermally at temperature T1 to volume 10.0m3.
Step 3 – then expanded adiabatically to volume 80.0m3.
Step 4 – then compressed isothermally at temperature T2 to volume 8.0m3.
Then T1/T2
2
4
6
8
The correct Answer is:B
Step by step video, text & image solution for One mole of an ideal monatomic gas undergoes the following four reversible processes : Step 1 – it is first compressed adiabatically from volume 8.0m^(3) to 1.0 m^(3). Step 2 – then expanded isothermally at temperature T_(1) to volume 10.0 m^(3). Step 3 – then expanded adiabatically to volume 80.0 m^(3). Step 4 – then compressed isothermally at temperature T_(2) to volume 8.0 m^(3). Then T_(1)//T_(2) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
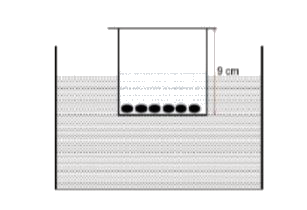
A wide bottom cylindrical massless plastic container of height 9 cm has 40 identical coins inside it and is floating on water with 3 cm inside the water. If we start putting more of such coins on its lid. It is observed that after N coins are put, its equilibrium changes from stable to unstable. Equilibrium in floating is stable if the geometric center of the submerged portion is above the center of mass of the object) The value of N is closed to
View SolutionAn ideal gas expands isothermally from volume V1 to V2 and is then compressed to original volume V1 adiabatically. Initialy pressure is P1 and final pressure is P3. The total work done is W. Then
View SolutionInitial pressure and volume of a gas are P and V respectively. First it is expanded isothermally to volume 4 V and then compressed adiabatically to volume V. The final pressure of gas will be (given γ=32)
View SolutionOne mole of an ideal monatomic gas undergoes the following four reversible processes : Step1 : It is first compressed adiabatically from volume V_(1) to 1m^(3) . Step 2 : then expanded isothermally to volume 10 m^(3) . Step 3 : then expanded adiabatically to volume V^(3) . Step 4 : then compressed isothermally to volume V_(1) . If the efficiency of the above cycle is 3/4 then V_(1) is,
View SolutionAn ideal gas at initial temperature T0 and initial volume V0 is expanded adiabatically to a volume 2V0 The gas is then expanded isothermally to a volume 5V0 , and there after compressed adiabatically so that the temperature of the gas becomes again T0 . If the final volume of the gas is αV0 then the value of constant α is
View SolutionThe initial state of 1 mol of an ideal gas is (P_(1),V_(1),T_(1)) . The gas is expanded by a reversible isothermal process and also by a reversible adiabatic process separately. If final volume of the gas is same in both the processes, and changes in internal energy in the isothermal and adiabatic processes are DeltaU_(1) and DeltaU_(2) respectively, then-
View SolutionAn ideal gas of volume V and pressure P expands isothermally to volume 16 V and then compressed adiabatically to volume V . The final pressure of gas is [γ=1.5]
View SolutionTwo moles of an ideal gas have an initial kelvin temperature T_i and absolute pressure P_i the gas undergoes a reversible isothermal compression from an initial volume V_i to a final volume 0.5 V_i The ratio of the molar specific heat capacity at constant pressure to that at constant volume, gamma ,for diatomic hydrogen gas is 7/5. In an adiabatic compression, the gas, originally at atmospheric pressure, is compressed from an original volume of 0.30 m^3 to 0.15 m^3 What is the final pressure of the gas?
View SolutionThe work done by one mole of a monatomic ideal gas (γ=5/3 ) in expanding adiabatically is 825 J . The initial temperature and volume of the gas are 393 K and 0.100 m3 obtain the final temperature of the gas .
View SolutionAn ideal gas expands isothermally from volume V_(1) to V_(2) and is then compressed to original volume V_(1) adiabatically. Initialy pressure is P_(1) and final pressure is P_(3) . The total work done is W . Then
View SolutionA given mass of gas at a pressure of 0.75 m of mercury is compressed isothermally until its volume is reduced to 3/4th of its volume. It is then made to expand adiabatically to a volume 1/5 times greater than its original volume. Calculate the final temperature if its initial temperature was 290 K.(Y=1.4)
View SolutionAn ideal gas expands isothermally from volume V_(1) to V_(2) and is then compressed to original volume V_(1) adiabatically. Initialy pressure is P_(1) and final pressure is P_(3) . The total work done is W . Then
View SolutionAn ideal gas of volume V and pressure P expands isothermally to volume 16 V and then compressed adiabatically to volume V . The final pressure of gas is [ gamma = 1.5]
View SolutionAn ideal gas expands isothermally from volume V1 to V2 and is then compressed to original volume V1 adiabatically. Initialy pressure is P1 and final pressure is P3. The total work done is W. Then
View SolutionA gas is compressed isothermally to half its volume. The same gas is compressed separately to half its volume by adiabatic process, then
View Solution