What is the force on q?
(√2−12)q216πε0d2
q216πε0d2
q232πε0d2
(√2q216πε0d2
To find the force on the charge q placed between two earthed planes, we can follow these steps:
Step 1: Understand the Setup
We have two earthed planes, which means they are at zero potential. A charge +q is placed at a distance d from both planes. Since the planes are earthed, they will influence the electric field in the region between them.
Step 2: Determine the Electric Field
The electric field E due to an infinite plane sheet of charge is given by:
E=σ2ϵ0
where σ is the surface charge density and ϵ0 is the permittivity of free space. For earthed planes, the electric field will be directed away from the positive charges towards the negative charges.
Step 3: Calculate the Forces Acting on Charge q
1. Force due to the left plane (F1):
- The electric field E1 due to the left plane at the position of charge q is directed towards the left plane.
- The force F1 on charge q is given by:
F1=qE1
2. Force due to the right plane (F2):
- The electric field E2 due to the right plane at the position of charge q is directed towards the right plane.
- The force F2 on charge q is given by:
F2=qE2
Step 4: Calculate the Resultant Force
Since the forces F1 and F2 are in opposite directions, the net force F on charge q can be calculated as:
F=F1−F2
Step 5: Substitute the Electric Fields
Assuming both planes have the same surface charge density, the magnitudes of the electric fields will be equal:
E1=E2=E
Thus, the net force becomes:
F=qE−qE=0
However, if the planes have different charge densities, we would need to calculate E1 and E2 separately based on their respective charge densities.
Step 6: Final Expression
If we assume the charge densities are equal, the forces cancel each other out. If they are not equal, we would need to calculate the difference in electric fields and substitute back into the force equations.
Conclusion
The force on charge q depends on the electric fields created by the planes. If they are equal, the net force is zero. If they differ, we would calculate the resultant force based on the difference in electric fields.
We have two earthed planes, which means they are at zero potential. A charge +q is placed at a distance d from both planes. Since the planes are earthed, they will influence the electric field in the region between them.
The electric field E due to an infinite plane sheet of charge is given by:
E=σ2ϵ0
where σ is the surface charge density and ϵ0 is the permittivity of free space. For earthed planes, the electric field will be directed away from the positive charges towards the negative charges.
1. Force due to the left plane (F1):
- The electric field E1 due to the left plane at the position of charge q is directed towards the left plane.
- The force F1 on charge q is given by:
F1=qE1
- The electric field E2 due to the right plane at the position of charge q is directed towards the right plane.
- The force F2 on charge q is given by:
F2=qE2
Since the forces F1 and F2 are in opposite directions, the net force F on charge q can be calculated as:
F=F1−F2
Assuming both planes have the same surface charge density, the magnitudes of the electric fields will be equal:
E1=E2=E
Thus, the net force becomes:
F=qE−qE=0
However, if the planes have different charge densities, we would need to calculate E1 and E2 separately based on their respective charge densities.
If we assume the charge densities are equal, the forces cancel each other out. If they are not equal, we would need to calculate the difference in electric fields and substitute back into the force equations.
The force on charge q depends on the electric fields created by the planes. If they are equal, the net force is zero. If they differ, we would calculate the resultant force based on the difference in electric fields.
Topper's Solved these Questions
Similar Questions
Consider three charges q1,q2 and q3 each equal to q at the vertices of an equilateral triangle of side 'l' what is the force on any charge due to remaining charges.
View SolutionConsider the charges q,q and -q placed at the vertices of an equilateral triangle of each side l. What is the force on each charge ?
View Solution
Knowledge Check
A particle carrying charge + q is held at the center of a square of each side arranged on the square as shown in Fig. If q = 2 muC, what is the net force on the particle?
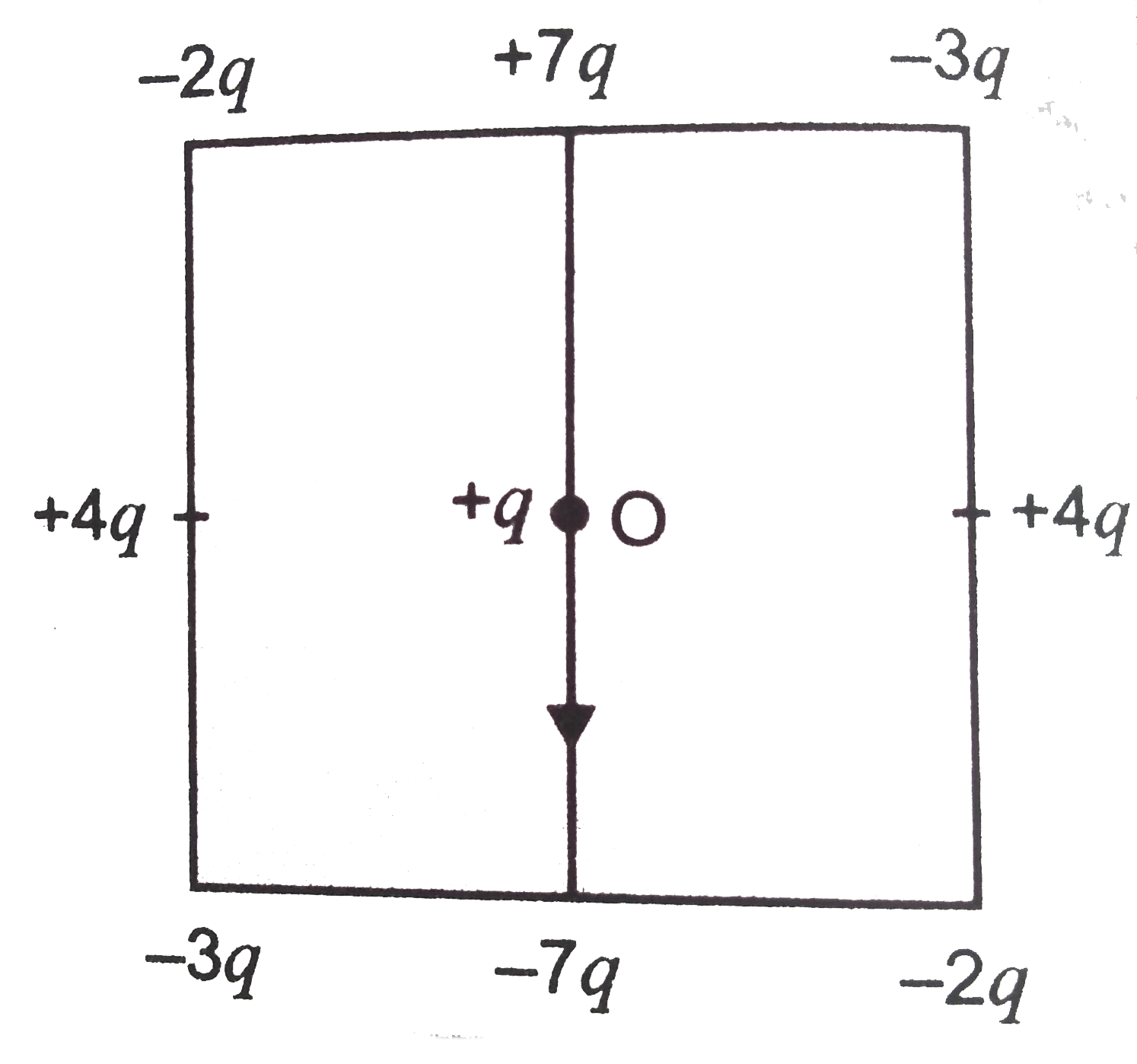 A36×14×4×10−3NB16×14×4×10−3NC26×14×4×10−3ND6×14×4×10−3N
A36×14×4×10−3NB16×14×4×10−3NC26×14×4×10−3ND6×14×4×10−3NThree charges q1,q2,q3 each equal to q placed at the vertices of an equilateral triangle of side l . What will be the force on a charge Q placed at the centroid of triangle ?
A4NB5NC10NDZeroPoint charge are placed at the verticas of a squre of side a as shown in fig. What should be the sign of charge q and magnitude of the ratio |q/Q| so that
(i) net force on each Q is zero?
(ii) net force on each q is zero?
Is it possible that the entire system could be in electrostatic equilibrium?
 A12√2 20√2B12√2 2√2C12√2 12√2D12√12 2√2
A12√2 20√2B12√2 2√2C12√2 12√2D12√12 2√2
Consider three charges q1,q2 and q3 each equal to q at the verticles of an equilateral triangle of side 'l' what is the force on any charge due to remianing charges.
View SolutionWhat is the force acting on charge (q) moving in a direction perpendicular to a magnetic field (B) with velocity v?
View SolutionFour charge Q,q,Q and q are kept at the four corners of a square as shown below. What is the relation between Q and q, so that the net force on a charge q is zero ?
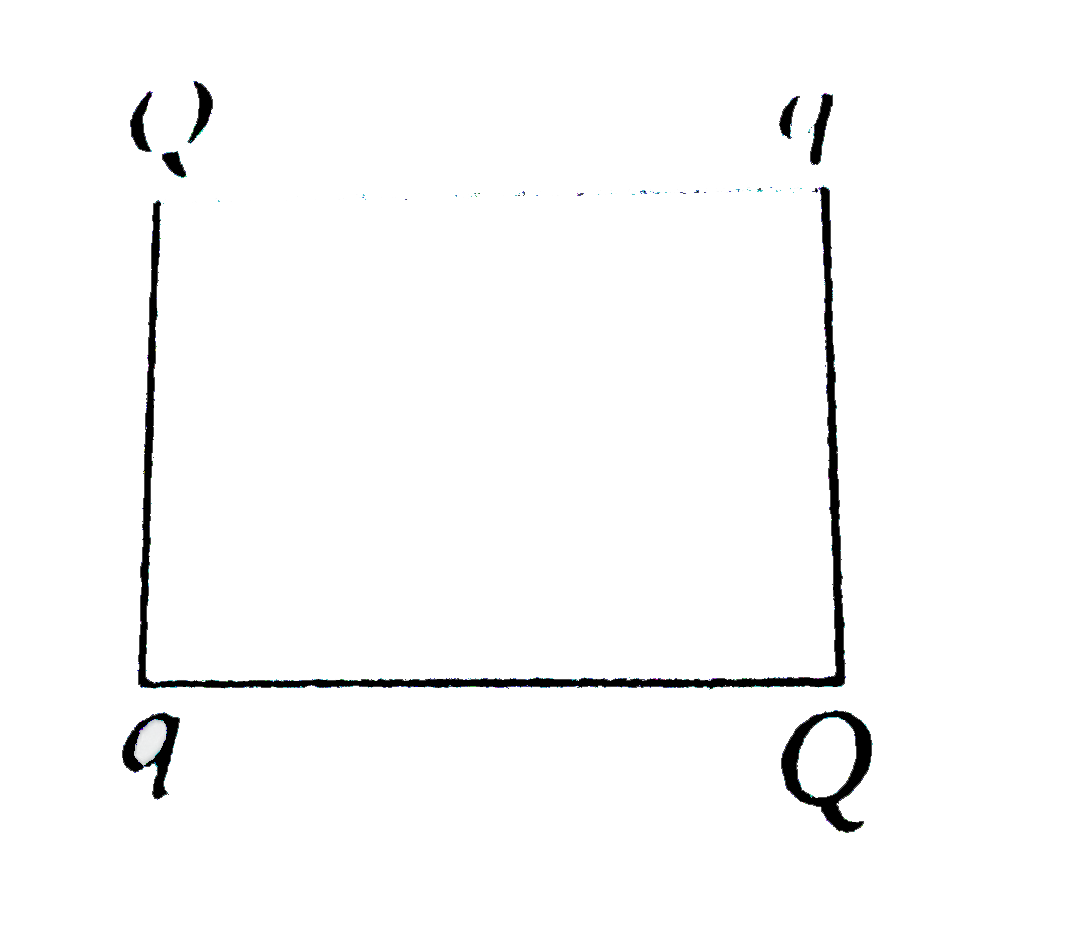
View SolutionWhat is the direction of the force acting on a charged particle q, moving with a velocity →v a uniform magnetic field →B ?
View SolutionWhat is the direction of the force acting on a charged. Particle q moving with a velocity →v in a uniform magnetic field →B ?
View Solution
