Figure shows a loop track whose lower part ends into a circular track of radius R and centre O.
A small solid sphere of mass M rolls without slipping along the loop track from the end A at a height 6R from the bottom of the track. What is the horizontal force acting on the sphere, when it rises up to the point P in level with the centre O of the circular part ?

307Mg
407Mg
507Mg
607Mg
The correct Answer is:C
The ball descends through a vertical distance 6R−R=5R
∴ Loss in P.E. of the ball = mg (5R) ….(1)
Kinetic energy of the ball at P
=KTranslational+KRotational
=12mv2+12Iω2
=12mv2(12×25MR2×v2R2)=710mv2 .....(2)
By the principle of conservation of energy.
mg(5R)=710mv2 ∴v2=50gR7 ....(3)
At point P, the velocity of the ball is tangentially upwards.
∴ A centripetal force acts on the ball along PO, i.e, it is along the horizontal.
∴ Horizontal force acting on the ball
=Mv2R=MR(50gR7)=507Mg
Topper's Solved these Questions
Similar Questions
A small solid sphere rolls without slipping along the track shown in figure. The sphere starts from rest from a height h above the bottom of a loop of radius R which is much larger than the radius of the sphere r. The minimum value of h for the sphere to complete the loop is:

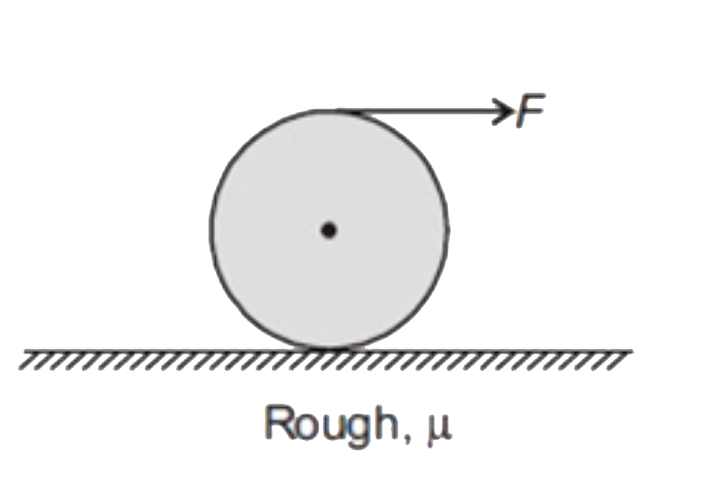
View SolutionA solid sphere of mass M and radius R is pulled by a force F as shown in figure . If the sphere does not slip over the surface , then frictional force acting on the sphere is
View Solution
Knowledge Check
A solid sphere of mass m and radius R rolls without slipping on a horizontal surface such that vc.m.=v0.
AThe kinetic energy of rotation is 15mv20.BThe total kinetic energy is 710mv20.CThe mechanical energy (assume the ground as reference) is mgR+710mv20.DAll options are correct.A small solid sphere of radius r rolls down an incline without slipping which ends into a vertical loop of radius R. Find the height above the base so that it just loops the loop
A52RB52(R−r)C2510(R−r)D2710R−17r10A sphere of mass M rolls without slipping on rough surface with centre of mass has constant speed v0. If mass of the sphere is m and its radius be R, then the angular momentum of the sphere about the point of contact is.
A45Mv0R(−ˆk)B95Mv0R(−ˆk)C85Mv0R(−ˆk)D75Mv0R(−ˆk)
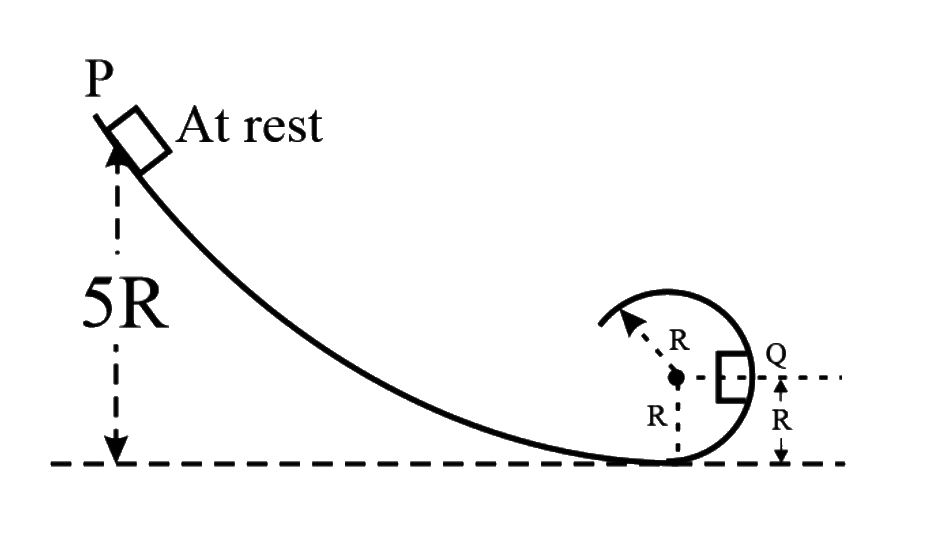
A small block of mass m slides along a smooth frictional track as shown in the figure. If it starts from rest at P, what is the resultant force acting on it at Q?
View SolutionA small solid marble of mass M and radius r rolls down along the loop track, without slipping. Find the height h above the base, from where it has to start rolling down the incline such that the sphere just completes the vertical circular loop of radius R.
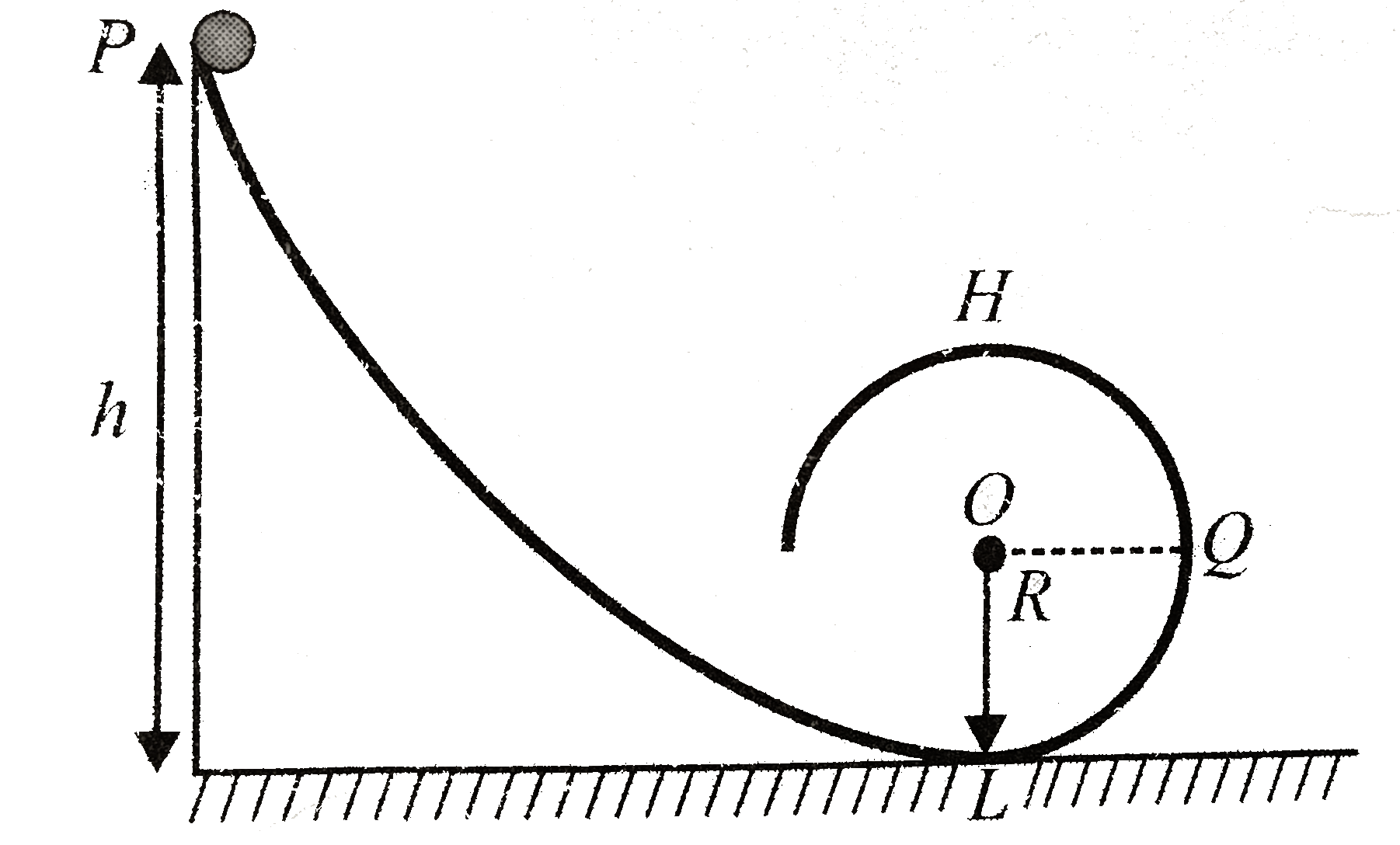
View SolutionA block of mass M with a semi - circular track of radius R rests on a smooth floor. A sphere of mass m and radius r is released from rest from A. Find the velocity of sphere and track , when the sphere reaches B.

View SolutionA small block of mass m slides along a smooth frictional track as shown in the figure. If it starts from rest at P, what is the resultant force acting on it at Q?

View SolutionA solid cylinder of mass M and radius R rolls down an inclined plane of height h without slipping. The speed of its centre when it reaches the bottom is.
View Solution
