A particle of mass m1 makes an elastic one dimensional collision with a stationary particle of mass m2.What fraction of the kinetic energy of m1 is carried away by m2?
m1m2
m2m1
2m1m2(m1+m2)2
4m1m2(m1+m2)2
The correct Answer is:D
To solve the problem of finding the fraction of kinetic energy of mass m1 that is carried away by mass m2 after an elastic collision, we can follow these steps:
Step 1: Understand the Initial Conditions
- Let the mass of the first particle be m1 and its initial velocity be v.
- The second particle has a mass m2 and is initially stationary, so its initial velocity u2=0.
Step 2: Calculate Initial Kinetic Energy of m1
- The initial kinetic energy KE1 of mass m1 is given by:
KE1=12m1v2
Step 3: Use the Formula for Final Velocities in Elastic Collisions
- For an elastic collision, the final velocities can be calculated using the following formulas:
- Final velocity of m1:
v′1=(m1−m2)(m1+m2)v
- Final velocity of m2:
v′2=2m1(m1+m2)v
Step 4: Calculate Final Kinetic Energy of m2
- The final kinetic energy KE2 of mass m2 after the collision is given by:
KE2=12m2(v′2)2
- Substitute v′2:
KE2=12m2(2m1(m1+m2)v)2
- Simplifying this gives:
KE2=12m2⋅4m21(m1+m2)2v2=2m21m2(m1+m2)2v2
Step 5: Calculate the Fraction of Kinetic Energy Transferred
- The fraction of kinetic energy transferred to m2 is given by:
Fraction=KE2KE1
- Substitute the expressions for KE2 and KE1:
Fraction=2m21m2(m1+m2)2v212m1v2
- Simplifying this gives:
Fraction=2m21m2(m1+m2)2⋅2m1=4m1m2(m1+m2)2
Final Answer
The fraction of the kinetic energy of m1 that is carried away by m2 is:
4m1m2(m1+m2)2
---
- Let the mass of the first particle be m1 and its initial velocity be v.
- The second particle has a mass m2 and is initially stationary, so its initial velocity u2=0.
- The initial kinetic energy KE1 of mass m1 is given by:
KE1=12m1v2
- For an elastic collision, the final velocities can be calculated using the following formulas:
- Final velocity of m1:
v′1=(m1−m2)(m1+m2)v
- Final velocity of m2:
v′2=2m1(m1+m2)v
- The final kinetic energy KE2 of mass m2 after the collision is given by:
KE2=12m2(v′2)2
- Substitute v′2:
KE2=12m2(2m1(m1+m2)v)2
- Simplifying this gives:
KE2=12m2⋅4m21(m1+m2)2v2=2m21m2(m1+m2)2v2
- The fraction of kinetic energy transferred to m2 is given by:
Fraction=KE2KE1
- Substitute the expressions for KE2 and KE1:
Fraction=2m21m2(m1+m2)2v212m1v2
- Simplifying this gives:
Fraction=2m21m2(m1+m2)2⋅2m1=4m1m2(m1+m2)2
The fraction of the kinetic energy of m1 that is carried away by m2 is:
4m1m2(m1+m2)2
Topper's Solved these Questions
- LINEAR MOMENTUM & ITS CONSERVATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - Advance McQ
20 Videos
- LINEAR MOMENTUM & ITS CONSERVATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - unsolved
110 Videos
- LINEAR MOMENTUM & ITS CONSERVATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - Conceptual MCQ s single option correct
30 Videos
- KINEMATICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - KINEMATICSEXERCISE - Unsolved Numerical Pro.
83 Videos
- NEWTONS LAWS OF MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Unsolved Numerical Problem
81 Videos
Similar Questions
A particle of mass m1 experienced a perfectly elastic collision with a stationary particle of mass m2. What fraction of the kinetic energy does the striking particle lose, if
(a) it recoils at right angles to its original motion direction,
(b) the collision is a head-on one?
View SolutionA particle of mass m experienced an elastic collision with a stationary particle of mass 2m . What fraction of the kinetic energy does the striking particle lose if recoils at the right angles to its original motion direction.
View Solution
Knowledge Check
A ball of mass m1 makes an elastic , one-dimensional collision with a stationary particle of mass m2. The fraction of the kinetic energy of m1 transferred to m2 is
A2m1m2(m1+m2)2B4m1m2(m1+m2)2Cm1m2(m1+m2)2D12m12m2(m1+m2)2A particle (A) of mass m1 elastically collides with another stationary particle (B) of mass m2. Then :
Am1m2=12 and the particles fly a part in the opposite direction with equal velocities.Bm1m2=13 and the particles fly apart in the opposite direction with equal velocities.Cm1m2=21 and the collision angle between the particles is 60∘ symmetrically.Dm1m2=21 and the particles fly apart symmetrically at an angle 90∘A particle of mass m1 makes a head - on elastic collision with another particle of mass m2 at rest. m1 rebounds straight back with 49 of its initial kinetic energy . Then m1m2 is :
A23B15C32D25
A particle of mass m1 experiences a perfectlly elastic collision with a stationary particle of mass m2. Match the entires in column I to all the entires in column II.
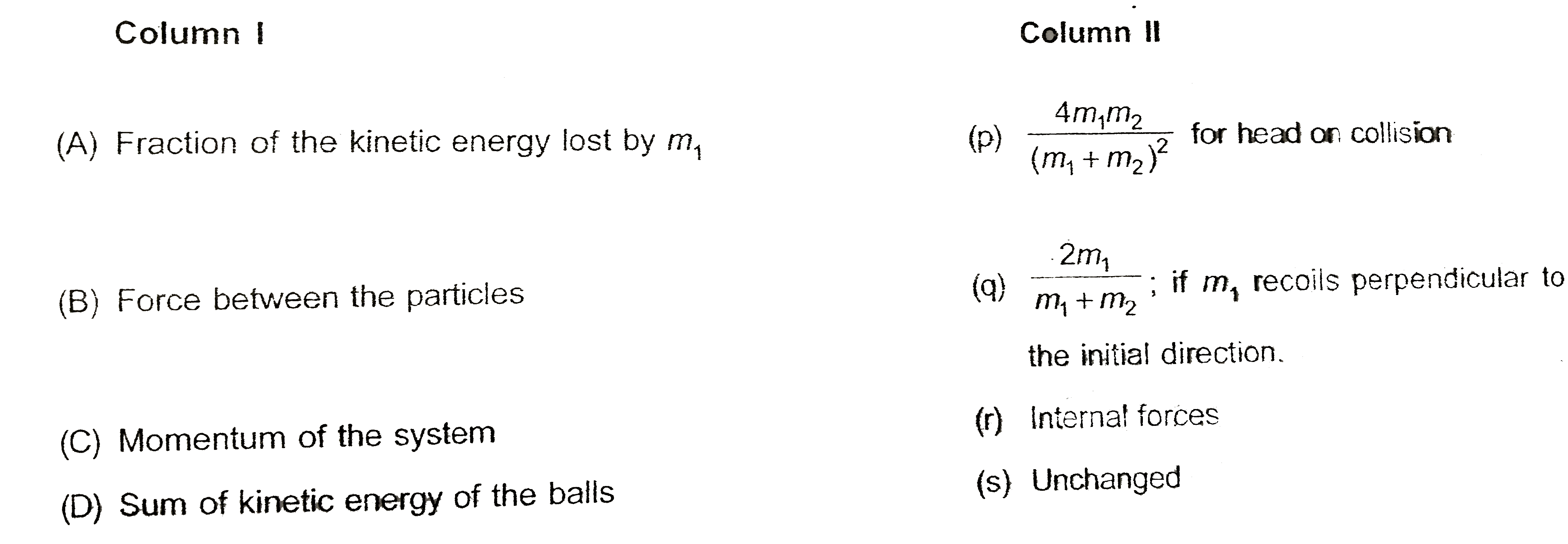
View SolutionA particle of mass m1 makes a head on elastic collision with another stationary body of mass m2, If m1 rebounds with half of its original speed then (m1m2) is
View SolutionA particle of mass m1, makes a head on elastic collision with another stationary body of mass m2 If m1, rebounds with half of its original speed then (m1m2) is
View SolutionA particle moving with kinetic energy =3J makes an elastic head-on collision with a stationary particle which has twice its mass. During the impact :-
View SolutionA particle moving with kinetic energy=3 J makes an elastic head-on collision with a stationary particle which has twice its mass. During the impact:
View Solution
