A particle moves in such a way that its position vector at any time t "is" hati+ Find a function of time (a) the velocity, (b) the speed, (c) the acceleration, (d) the magnitude of the acceleration, (e) the magnitude of the component of acceleration along velocity (called tangential' acceleration), (f) the magnitude of the component ofacceleration perpendicular to velocity (called normal acceleration).
Step by step video & image solution for A particle moves in such a way that its position vector at any time t "is" t "is" hati+1/2 t^(2) hatj + thatK Find a function of time (a) the velocity, (b) the speed, (c) the acceleration, (d) the magnitude of the acceleration, (e) the magnitude of the component of acceleration along velocity (called tangential' acceleration), (f) the magnitude of the component ofacceleration perpendicular to velocity (called normal acceleration). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- DESCRIPTION OF MOTIONBOOK - ANURAG MISHRACHAPTER - DESCRIPTION OF MOTIONEXERCISE - Level-1
86 Videos
- DESCRIPTION OF MOTIONBOOK - ANURAG MISHRACHAPTER - DESCRIPTION OF MOTIONEXERCISE - Level-2
25 Videos
- DESCRIPTION OF MOTIONBOOK - ANURAG MISHRACHAPTER - DESCRIPTION OF MOTIONEXERCISE - Level-3
34 Videos
- FORCE ANALYSISBOOK - ANURAG MISHRACHAPTER - FORCE ANALYSISEXERCISE - Matching type
13 Videos
Similar Questions
A particle moves in such a way that its position vector at any time t is →r=tˆi+12t2ˆj+tˆk.
Find as a function of time:
(i) The velocity (d→rdt) (ii) The speed (∣∣∣d→rdt∣∣∣) (iii) The acceleration (d→vdt)
(iv) The magnitude of the acceleration
(v) The magnitude of the component of acceleration along velocity (called tangential acceleration)
(v) The magnitude of the component of acceleration perpendicular to velocity (called normal acceleration).
View SolutionAn object moves in such a way that its position (in meters) as a function of time (in seconds) is →r=ˆi+3t2ˆj+tˆk. Give expressions for (a) the velocity of the object and (b) the acceleration of the object as functions of time.
View Solution
Knowledge Check
An object is moving in x−y plane its velocity and acceleration at t=0 are represented in figure.

The ratio of magnitude of velocity to magnitude of component of acceleration along velocity at t=0 :-A83B85C2D58For a body in circular motion with a constant angular velocity, the magnitude of the average acceleration over a period of half a revolution is … times the magnitude of its instantaneous acceleration.
A2πBπ2CπD2A particle has initial velocity (2ˆi+3ˆj) and acceleration (0.3ˆi+0.2ˆj). The magnitude of velocity after 10 second will be
A9unitsB9√2unitsC5√2unitsD5units
A particle moves in xy plane with its position vector changing with time (t) as →r=(sint)ˆi+(cost)ˆj ( in meter)
Find the tangential acceleration of the particle as a function of time. Describe the path of the particle.
View SolutionFigur shows the total acceleration and velocity of a particle moving clockwise in a circle of radius 2.5m at a given instant of time. At this instant, find:
(a) the radius acceleration,
(b) the speed of the acceleration,
(c) its tangential acceleration.
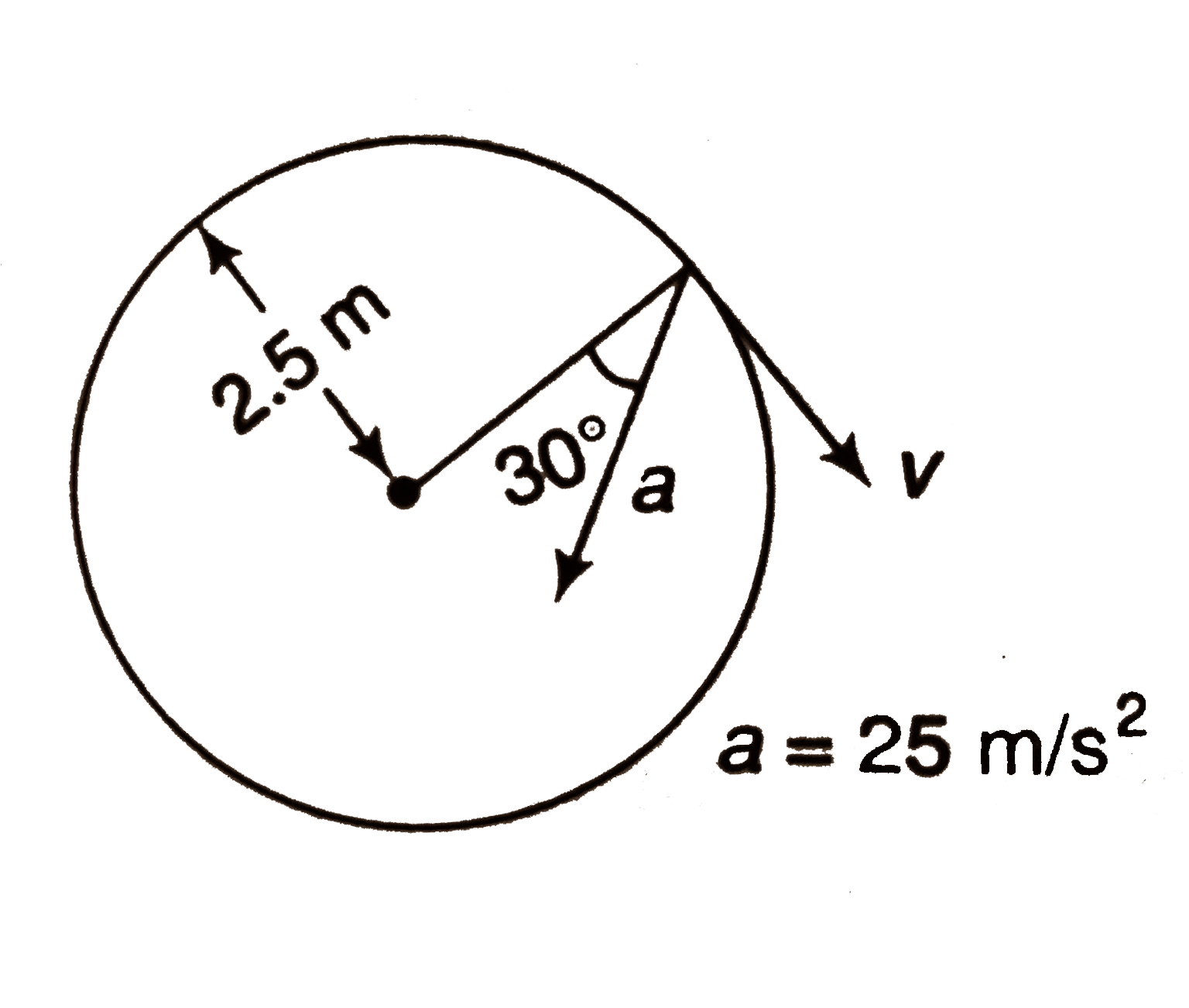
View SolutionA particle moves along the space curve →r=(t2+t)ˆi+(3t−2)ˆj+(2t3−4t2)ˆk. (t in sec, r in m ) Find at time t=2 the (a) velocity, (b) acceleration, (c) speed or magnitude of velocity and (d) magnitude of acceleration.
View SolutionA particle moves along a staight line such that its displacement at any time t is given by s=t3−6t2+3t+4m. Find the velocity when the acceleration is 0.
View SolutionThe position vector of an object at any time t is given by 3t2ˆi+6thayj+ˆk. Its velocity along y-axis has the magnitude
View Solution
