A thin metallic disc is rotating with constant angular velocity about a vertical axis that is perpendicular to its plane and passes through its centre. The rotation causes the free electrons in the disc to redistribute. Assume that there is no external electric or magnetic field. Then
a point on the rim of the disc is at a higher potential than the centre.
a point on the rim of the disc is at a lower potential than the centre.
a point on the rim of the disc is at the same potential as the centre
the potential in the material has an extremum between center and the rim
The correct Answer is:B
Step by step video, text & image solution for A thin metallic disc is rotating with constant angular velocity about a vertical axis that is perpendicular to its plane and passes through its centre. The rotation causes the free electrons in the disc to redistribute. Assume that there is no external electric or magnetic field. Then by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
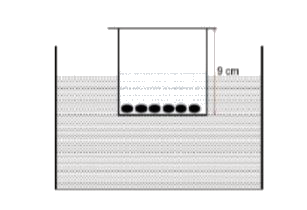
A wide bottom cylindrical massless plastic container of height 9 cm has 40 identical coins inside it and is floating on water with 3 cm inside the water. If we start putting more of such coins on its lid. It is observed that after N coins are put, its equilibrium changes from stable to unstable. Equilibrium in floating is stable if the geometric center of the submerged portion is above the center of mass of the object) The value of N is closed to
 04:00View Solution
04:00View SolutionA charge q is uniformly distributed on a non-conducting disc of radius R. It is rotated with an angular speed co about an axis passing through the centre of mass of the disc and perpendicular to its plane. Find the magnetic moment of the disc.
01:51View SolutionA circular disc is rotating in horizontal plane about vertical axis passing through its centre without friction with a person standing on the disc at its edge. If the person gently walks to centre of disc then its angular velocity
01:53View SolutionA metal disc of radius R rotates with an angular velcoity ω about an axis perpendicular to its plane passing through its centre in a magnetic field B acting perpendicular to the plane of the disc. Calculate the induced emf between the rim and the axis of the disc.
01:45View SolutionA thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity omega . Another disc of same dimensions but of mass (1)/(4) M is placed gently on the first disc co-axially. The angular velocity of the system is
02:15View SolutionA disc of mass 2 kg and diameter 40 cm is free to rotate about an axis passing through its centre and perpendicular to its plane. If a force of 50 N is applied to the disc tangentially Its angular acceleration will be
01:28View SolutionRadius of gyration of disc rotating about an axis perpendicular to its plane passing through through its centre is (If R is the radius of disc)
02:12View SolutionThe moment of inertia of a copper disc, rotating about an axis passing through its centre and perpendicular to its plane
View SolutionA thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity omega . Another disc of same dimensions but of mass M/4 is placed gently on the first disc coaxially. The angular velocity of the system now is
View SolutionA thin and circular disc of mass m and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity ω . If another disc of same dimension but mass ml4 is placed gently on the first disc co-axially, then the new angular velocity of the system is
02:14View SolutionA charge q is uniformly distributed on a non-conducting disc of radius R . It is rotated with an angular speed co about an axis passing through the centre of mass of the disc and perpendicular to its plane. Find the magnetic moment of the disc.
03:24View SolutionA thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity omega . Another disc of same dimensions but of mass (1)/(4) M is placed gently on the first disc co-axially. The angular velocity of the system is
03:46View SolutionThe moment of inertia of a copper disc, rotating about an axis passing through its centre and perpendicular to its plane
03:00View SolutionA metal disc of radius R rotates with an angular velcoity omega about an axis perpendiclar to its plane passing through its centre in a magnetic field B acting perpendicular to the plane of the disc. Calculate the induced emf between the rim and the axis of the disc.
06:16View SolutionIn a Faraday disc dynamo, a metal disc of radius R rotates with an angular velocity about an axis perpendicular to the plane of the disc and passing through its centre.The disc is placed in a magnetic field B acting perpendicular to the plane of the disc.Determine the induced emf between the rim and the axis of the disc.
03:08View Solution

