A monochromatic light source S of wavelength 440 nm is placed slightly above a plane mirror M as shown . Image of S in M can be used as a virtual source to produce interference fringes on the screen. The distance of source S from O is 20.0 cm, and the distance of screen from O is 100.0 cm (figure is not to scale). If the angle θ=0.50×10−3 radians, the width of the interference fringes observed on the screen is –

2.20 mm
2.64 mm
1.10 mm
0.55 mm
The correct Answer is:B
Step by step video, text & image solution for A monochromatic light source S of wavelength 440 nm is placed slightly above a plane mirror M as shown . Image of S in M can be used as a virtual source to produce interference fringes on the screen. The distance of source S from O is 20.0 cm, and the distance of screen from O is 100.0 cm (figure is not to scale). If the angle theta = 0.50 xx10^(-3) radians, the width of the interference fringes observed on the screen is – by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
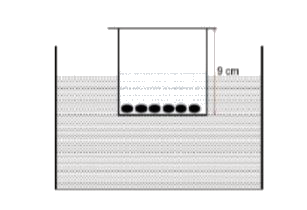
A wide bottom cylindrical massless plastic container of height 9 cm has 40 identical coins inside it and is floating on water with 3 cm inside the water. If we start putting more of such coins on its lid. It is observed that after N coins are put, its equilibrium changes from stable to unstable. Equilibrium in floating is stable if the geometric center of the submerged portion is above the center of mass of the object) The value of N is closed to
View SolutionInterference effects are produced at point P on a screen as a result of direct rays from a 500 nm source and reflected rays from a mirror, as shown in figure. If the sources is 100m to the left of the screen and 1.00 cm above the mirror, find the distance y (in milimeters) to the first dark band above the mirror.
View SolutionIn Lloyd's mirror experiment (Fig.) a light wave emitted directly be the source S (narrow slit) interferes with the wave reflected form a mirror M. As s result, an interference fringe pattern is

formed on the screen Sc. the source and the mirror are separated by a distance l=100cm.At a certain position of the source the fringe width on the screen was equal to Δx=0.25mm, and after source was moved away from the mirror plane by Δh=0.60mm, the fringe width decreased η=1.5 times. Find the wavelength of light.
View Solution_E01_104_Q01.png)
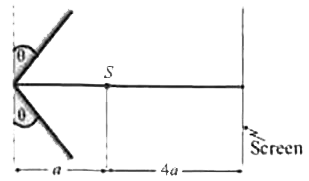
Two plane mirrors, a source S of light, wmitting monochromatic rays of wavelength λ and a screen are arranged as shown in figure. If angle θ is very small, calculate fringe width of the interference pattern formed by reflected rays.
View SolutionA monochromatic point source (S_(1)) is at a distance d from a screen. Another identical source (S_(2)) is at a large distance from the screen. The two sources are on a line which is perpendicular to the screen. Sources are coherent. What is the shape of interference fringes on the screen? (b) Three identical coherent sources are placed on a straight line with two neighbouring ones separated by a distance "d = 0.06 mm" . The sources produce monochromatic light of wavelength lambda" = 550.4 nm" . A line AB is located at a distance D = 2.50 m from the sources and is perpendicular to line joining the three sources. Intensity of light at P with any one of the sources switched on is I_(o) . Find the intensity when all three sources are switched on. Distance of point P from O is "y = 1.72 cm (see figure)"
View SolutionA point source of light 'S' at a distance d from the screen A produces light intensity I_(0) at the centre of the screen. If a completely reflecting mirror M is placed at a distance d behind the source as shown in the figure, find the intensity at the centre of the screen
View SolutionIn Lloyd's single mirror interference experiment, the source slit is at a distance of 2 mm from the plane mirror . The screen is kept at a distance of 1.5m from the source . If light of wavelength 5890 Å is used, calculate the fringe width.
View SolutionIn Lloyd's interference experiment, 10 fringes occupy a space of 1.5 mm. The distance between the source and the screen is 1.25 m. If light of wavelength 6000 Å is used, find the distance of the source from the plane minor.
View SolutionLight source of wavelength 400 nm used in a Lloyd's mirror, lies 1 mm above the plane mirror. Find the distance of first minima above the mirror. Distance of the screen from the plane of the sources is 2 m.
View SolutionThe distance between two coherent sources is 1 mm. The screen is placed at a distance of 1 m from the sources. If the distance of the third bright fringe is 1.2 mm from the central fringe, the wavelength of light used is
View SolutionIn Young's double slit experiment the coherent sources are 1.5 mm apart and the fringes are obtained on a screen at a distance 2.5 m from the plane of the sources. If the sources is illuminated with a light of 589.3 nm wavelength , find the number of fringes in the interference pattern thus formed on the screen. Total length of the fringes are 4.9×10−3 m.
View Solutionयंग के द्विस्लिट प्रयोग में दो स्त्रोतों के बीच दूरी 0.1 mm है। स्त्रोत से परदे की दूरी 20 cm है। प्रयुक्त किए गए प्रकाश का तरंगदैर्ध्य 5460Å है। तब पहले अदीप्त फ्रिन्ज की कोणीय स्थिति है
View SolutionThere are two plane mirrors. They are mutually inclined as shown in figure. S is a source of monochromatic light of wavelength λ. The reflected beam interfere and fringe pattern is obtained on the screen. If θ is small, the fringe width will be :
View SolutionInterference effects are produced at point P on a screen as a result of direct rays from a 500 nm source and reflected rays from a mirror, as shown in figure. If the sources is 100m to the left of the screen and 1.00 cm above the mirror, find the distance y (in milimeters) to the first dark band above the mirror.
View SolutionYou are standing at a distance 2 metre from a plane mirror.
What is the distance of your image from the mirror ?
View Solution