_E01_104_Q01.png)
Two plane mirrors, a source S of light, wmitting monochromatic rays of wavelength λ and a screen are arranged as shown in figure. If angle θ is very small, calculate fringe width of the interference pattern formed by reflected rays.
The correct Answer is:2(a+b)λ4aθ
_E01_104_S01.png)
Distance between two sources S1 and S2 d=2×2acosθsinθ=2asin2θ
Screen distance D=b+2acos2θ
β=λDd=λ(b+2acos2θ)2asin2θ=λ(b+2a)4aθ
(if θ is very much small)
Topper's Solved these Questions
- WAVE OPTICSBook:ALLENChapter:WAVE OPTICSExercise:Exercise 5 A (Previous Years Questions)
Explore 17 Videos
- WAVE OPTICSBook:ALLENChapter:WAVE OPTICSExercise:Exercise 5 B (Previous Years Questions)
Explore 19 Videos
- WAVE OPTICSBook:ALLENChapter:WAVE OPTICSExercise:Exercise 4 A (Conceptual subjective Exercise)
Explore 9 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR Book:ALLENChapter:UNIT & DIMENSIONS, BASIC MATHS AND VECTOR Exercise:Exercise (J-A)
Explore 7 Videos
Similar Questions
Two plane mirrors, a source S of light, emitting wavelengths ofλ1=4000∘A andλ2=5600∘Aand a screen are arranged as shown in figure. The angle θ shown is 0.05 radian and distance a and b are 1 cm and 38 cm respectively.
(a) Find the fringe width of interference pattern formed on screen by the blue light.
(b) Calculate the distance of first black line from central bright fringe.
(c) Find the distance between two black lines which are nearest to the central bright fringe.

View SolutionA prism having angle A∘ (which is very small) is placed in front of a point source S at a small distance d. A screen is placed at a large distance D as shown in the figure-6.113. Find the fringe width of interference pattern given that source is emitting light of wavelength λ and refractive index of prism is μ.
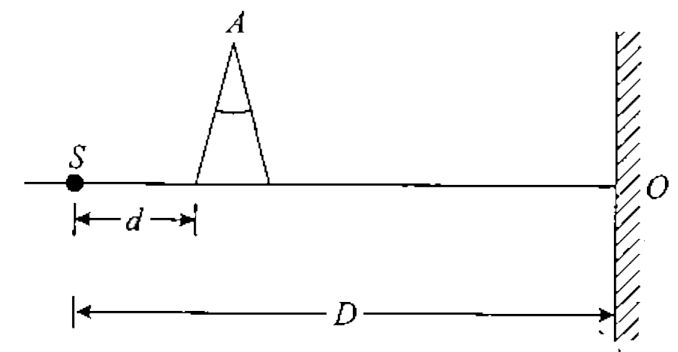
View Solution
Knowledge Check
Monochromatic green light of wavelength 500 nm illuminates a pair of slits 1 mm apart. Separation between the two consecutive bright fringes on the interference pattern formed on a screen 2 m away is
A0.25mmB0.1mmC1.0mmD0.01mmThere are two plane mirrors. They are mutually inclined as shown in figure. S is a source of monochromatic light of wavelength λ. The reflected beam interfere and fringe pattern is obtained on the screen. If θ is small, the fringe width will be :
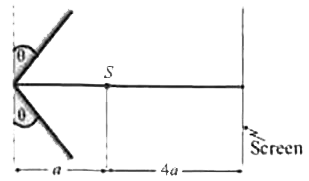 Aλ/θB3λ/2θC2λ/3θDnone of these
Aλ/θB3λ/2θC2λ/3θDnone of theseTwo plane mirrors are at an angle of 30∘. Find the deviation produced in the ray after 3 successive reflections as shown in figure
 A80∘B30∘C40∘D50∘
A80∘B30∘C40∘D50∘
Two plane mirror inclined at an angle of 60° as shown in the figure, then the angle between the incident ray and the first reflected ray is_____°
View SolutionA Lloyd's mirror or length 5 cm is illuminated with mon ochromatic light of wavelength λ(=6000Å) from a narrow 1 mm slit in its plane and 5 cm plane from its near edge. Find the fringe width on a screen 120 cm from the slit and width of interference pattern on the screen.

View SolutionLight incidence on a sphere of refractive index √3 placed in a air as shown in Figure. Find the angle (θ)in degree between emergent ray and reflected ray.
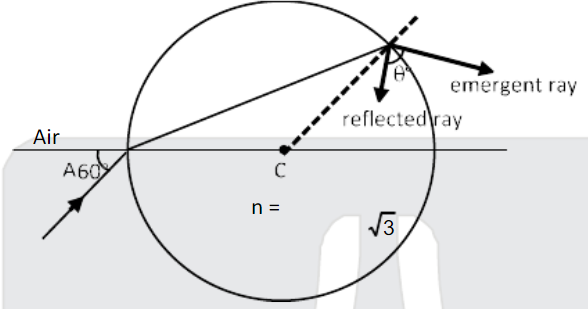
View SolutionA ray of light is reflected by two perpendicular plane mirrors as shown. The angle θ is equal to:

View SolutionTwo monochromatic sources of light A and B of wavelengths 5500 Å and 4400 Å respectively are used simultanesouly to produce interference bands on a screen. Which orders of the fringes in the two interference patterns will coincide?
View Solution
