Find bond enthalpy of C=O (in kJ/mol) using following information :
ΔHatomisation[C(s)]=700 kJ/mol
ΔHf[CO2(g)]=−400 kJ/mol
BEo=o=500 kJ/mol
Resonance energy of CO2=−150 kJ/mol
The correct Answer is:725
To find the bond enthalpy of the C=O bond using the provided information, we can follow these steps:
Step 1: Write the formation reaction of CO2
The formation of carbon dioxide (CO2) from its elements can be represented as:
C(s)+O2(g)→CO2(g)
Step 2: Use the given enthalpy of formation
The enthalpy of formation (ΔHf) of CO2 is given as:
ΔHf(CO2(g))=−400kJ/mol
Step 3: Write the expression for the reaction enthalpy
The reaction enthalpy (ΔHr) can be expressed as:
ΔHr=Bond enthalpies of reactants−Bond enthalpies of products
Step 4: Identify the bond enthalpies of reactants
For the reactants:
- The bond enthalpy of solid carbon (C(s)) is given as the atomization energy:
ΔHatomization(C(s))=700kJ/mol
- The bond enthalpy of O2 (as a double bond) is given as:
BEO=O=500kJ/mol
Thus, the total bond enthalpy of reactants is:
Bond enthalpy of reactants=700+500=1200kJ/mol
Step 5: Identify the bond enthalpies of products
For the products:
- CO2 has two C=O bonds, so we denote the bond enthalpy of C=O as x. Therefore, the bond enthalpy for two C=O bonds is 2x.
- The resonance energy of CO2 is given as:
Resonance energy=−150kJ/mol
Thus, the total bond enthalpy of products is:
Bond enthalpy of products=2x+(−150)=2x−150
Step 6: Set up the equation using the reaction enthalpy
Now we can set up the equation using the reaction enthalpy:
−400=1200−(2x−150)
Step 7: Solve for x
Rearranging the equation gives:
−400=1200−2x+150
−400=1350−2x
2x=1350+400
2x=1750
x=17502=875kJ/mol
Step 8: Conclusion
The bond enthalpy of C=O is:
Bond enthalpy of C=O=875kJ/mol
The formation of carbon dioxide (CO2) from its elements can be represented as:
C(s)+O2(g)→CO2(g)
The enthalpy of formation (ΔHf) of CO2 is given as:
ΔHf(CO2(g))=−400kJ/mol
The reaction enthalpy (ΔHr) can be expressed as:
ΔHr=Bond enthalpies of reactants−Bond enthalpies of products
For the reactants:
- The bond enthalpy of solid carbon (C(s)) is given as the atomization energy:
ΔHatomization(C(s))=700kJ/mol
- The bond enthalpy of O2 (as a double bond) is given as:
BEO=O=500kJ/mol
Bond enthalpy of reactants=700+500=1200kJ/mol
For the products:
- CO2 has two C=O bonds, so we denote the bond enthalpy of C=O as x. Therefore, the bond enthalpy for two C=O bonds is 2x.
- The resonance energy of CO2 is given as:
Resonance energy=−150kJ/mol
Bond enthalpy of products=2x+(−150)=2x−150
Now we can set up the equation using the reaction enthalpy:
−400=1200−(2x−150)
Rearranging the equation gives:
−400=1200−2x+150
−400=1350−2x
2x=1350+400
2x=1750
x=17502=875kJ/mol
The bond enthalpy of C=O is:
Bond enthalpy of C=O=875kJ/mol
Topper's Solved these Questions
Similar Questions
Calculate the ΔH∘f of C6H12O6(s) from the following data:
ΔHcomb[C6H12O6(s)]=−2816.kJ/mol
ΔH∘f of CO2(g)=−393.5kJ/mol
ΔH∘f of H2O(l)=−285.9kJ/mol
View SolutionWhat is the Bond energy (In kJ/ mol) of C−H in Methane from the following data?
ΔHf[CO2(g)]=−394kJ/molΔHf[H2O(l)]=−285kJ/molΔHsiblimation{Carbon (graphite)}=+716kJ/molΔHcombustion[CH4(g)]=−890kJ/molBond energy(H−H)=435kJ/mol
View Solution
Knowledge Check
Calculate resonance enthalpy of CO2(g) from following data:
ΔH∘combustion[Cgraphite]=−390Kj/mol
ΔH∘Sublimation[Cgraphite]=−715Kj/mol
ΔHB.E.[O=O]=500KJ/mol
ΔHB.E.[C=O]=875KJ/molA−40KJ/molB−145KJ/molC−72.5KJ/molD−290KJmolWhat will be the value of resonance energy of N2O if :
ΔHBDEN=N=400kJ/mol
ΔH∘fN2O=100kJmol−1
ΔHBDEN=N=950kJ/mol
ΔHBDEN=O=600kJmol−1
ΔHBDEO=O=500kJ/molA200kJ/molB150kJ/molC100kJ/molD50kJ/molThe bond energy of C=O if ΔH∘f(CO2)=390kJ, ΔH∘sublimation(Graphite)=720 kJ, O=O bond energy = 490 kJ and renonce energy (CO2)=36 kJ :
A782 kJB1564 kJC500 kJD626 kJ
Calculate the enthalpy of combustion of benzene (l) on the basis of the following data:
(i) Resonance energy of benzene (l) = -152 kJ/mol.
(ii) Enthalpy of hydrogenation of cyclohexene (l) = -119 kJ/mol.
(iii) ΔH∘l of H2O (l) = -285.8 kJ/mol.
(iv) ΔH∘l of H2O (l) = -285.8 kJ/mol<br>(v) ΔH∘l of CO2(g)=−393.5 kJ/mol.
View SolutionBorn- Hyber cycle below respesents the energy changes occurring as 298 K, jwhen MO(s) is formed from its elements, wherx x, y,z, a, b,c and d are enthalpy change elements, for corresponding pro0cesses respectively
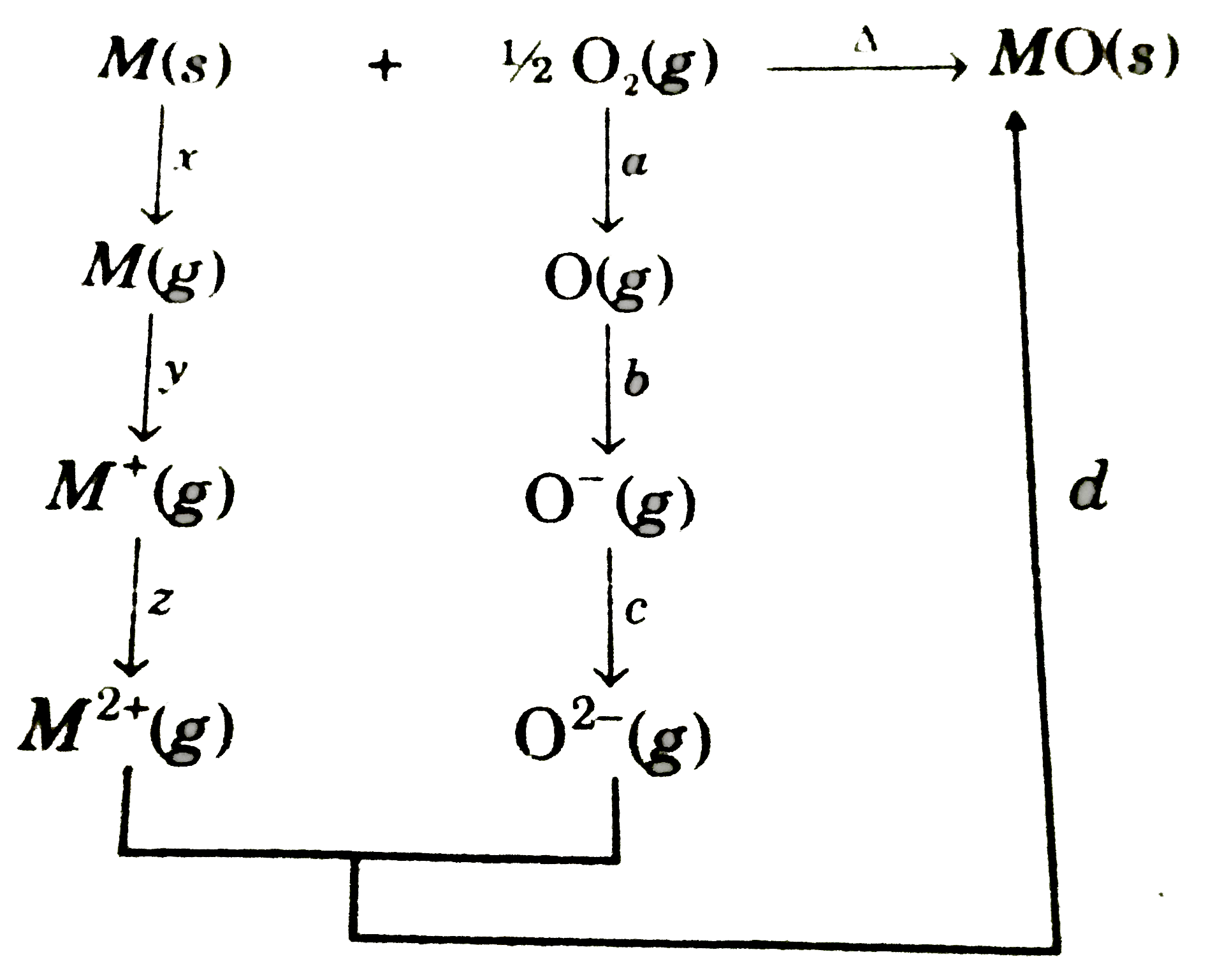
ΔHsubofM=180kJ/mol
I.E1(M)=218kJ/mol
I.E2(M)=384kJ/mol
ΔHatomisationofO2=640kJ/mol
ΔH(O→O−)=−142kJ/mol
ΔH(O→O−2)=−844kJ/mol
If ΔHfof MO(s) is −196kJ/mol then lattice energy of MO (s) will be :
View SolutionNitroglycerine (MW =227.1) denotes according to the following equation :
2C3H5(NO3)3I→3N2(g)+1/2O2(g)+6CO2(g)+5H2O(g)
The standard molar enthalpies of formation, ΔH∘f for the compounds are given bellow:
ΔH∘f[C3H5(NO3)3]=−364kJ/mol
ΔH∘f[CO2(g)]=−395.5kJ/mol
ΔH∘f[H2O(g)]=−241.8kJ/mol
ΔH∘f[N2(g)]=0kJ/mol
ΔH∘f[O2(g)]=0kJ/mol
The enthalpy change when 10g of nitroglycerine is detonated is
View Solutionयदि ΔH∘f(Et−S−Et)=−147 kJ/mol
ΔH∘f(Et−S−S−Et)=−202 kJ/mol और
ΔH∘fS(g)=+223 kJ/mol है तो S-S बंध ऊर्जा है
View SolutionThe Standard heat of formation of carbon atom gaseous state in KJ/mol is:
ΔH∘(atomisation of CO gas)=1072KJ/mol
ΔH∘(formation of 'O' gas)=247KJ/mol
ΔH∘(formation of CO gas)=−114KJ/mol
View Solution
