A particle moves in a plane along an elliptic path given by x2a2+y2b2=1 At point (0, b), the x-component of velocity is u. The y-component of acceleration at this point is-
−bu2/a2
−u2/b
−au2/b2
−u2/a
Step by step video & image solution for A particle moves in a plane along an elliptic path given by (x^(2))/(a^(2))+(y^(2))/(b^(2))=1 At point (0, b), the x-component of velocity is u. The y-component of acceleration at this point is- by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
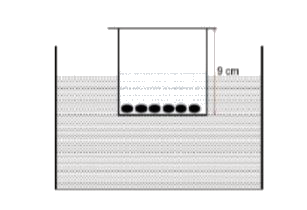
A wide bottom cylindrical massless plastic container of height 9 cm has 40 identical coins inside it and is floating on water with 3 cm inside the water. If we start putting more of such coins on its lid. It is observed that after N coins are put, its equilibrium changes from stable to unstable. Equilibrium in floating is stable if the geometric center of the submerged portion is above the center of mass of the object) The value of N is closed to
View SolutionA particle moves along the parabolic path x = y^2 + 2y + 2 in such a way that Y-component of velocity vector remains 5ms^(-1) during the motion. The magnitude of the acceleration of the particle is
View SolutionA particle whose velocity is given as vecv=hati+6thatjm//s is moving in x-y plane. At t-0, particle is at origin. Find the radius of curvature of path at point ((sqrt(2))/(3)m,(2)/(3)m)
View SolutionA particle starts from the origin of coordinates at time t=0 and moves in the xy plane with a constant acceleration α in the y-direction. Its equation of motion is y=βx2. Its velocity component in the x-directon is
View SolutionA particle moves along a parabolic path y=−9x2 in such a way that the x component of velocity remains constant and has a value 13m/s. Find the instantaneous acceleration of the projectile (in m/s2)
View SolutionA particle moves with a costant speed u along the curve y = sin x. The magnitude of its acceleration at the point corresponding to x= pi//2 is
View Solutionएक कण xy-तल में नियत त्वरण a से y -दिशा में गति करता है, जो समय t=0 पर मूल बिन्दु से गुजरता है। यदि इसकी गति का समीकरण y=bx2 (b एक नियतांक है) हो, तब x-दिशा में इसके वेग का घटक है
View SolutionVelocity of a particle v = 3hati M / s and fixed acceleration a = (- 1hati -5hatj) "मी/से"^(2) Free point, find the y component of the velocity when the particle reaches the maximum value of the x component of velocity
View SolutionA particle moves along the X-axis as x=u(t-2)+a(t-2)^(2) a) the initial velocity of the particle is u b) the acceleration of the particle is 2a c) at t=2s particle is at the origin
View SolutionA particle moves along the X-axis as x=u(t−2)+a(t−2)2
a) the initial velocity of the particle is u
b) the acceleration of the particle is 2a
c) at t=2s particle is at the origin
View SolutionA particle move along the parabolic path x=y^(2)+2y+2 in such a way that the y-component of velocity vector remain 5m//s during the motion. The magnitude the acceleration of the particle is k(25)//sec. where 'k' is
View SolutionA particle moves along a path y=ax2 (where a is constant) in such a way that x-component of its velocity (ux) remains constant. The acceleration of the particle is
View SolutionA particle moves along the parabolic path x=y2+2y+2 in such a way that Y-component of velocity vector remains 5ms−1 during the motion. The magnitude of the acceleration of the particle is
View SolutionA particle moves along a parabolic path y=-9x^(2) in such a way that the x component of velocity remains constant and has a value 1/3m//s . Find the instantaneous acceleration of the projectile (in m//s^(2) )
View SolutionA particle moves along a path y=ax2 (where a is constant) in such a way that x-component of its velocity (ux) remains constant. The acceleration of the particle is
View Solution