Find out the centre of mass of a composite object shown in figure. Object consists of a cone with its base joint with the base of a hemisphere. The dimensions of the object are shown in figure. Assume uniform density of the system.
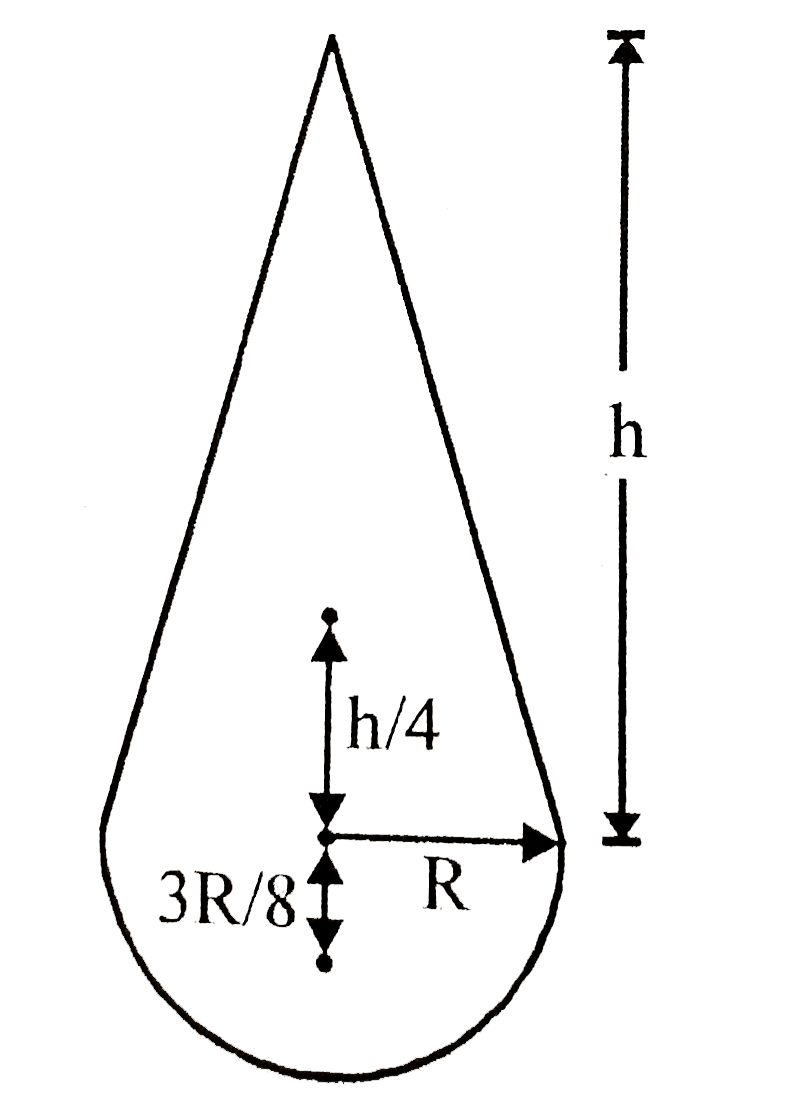
The shown object is made up of joining a solid cone and a hemisphere. We already know the location of the centre of mass of a cone and that of a hemisphere. The masses of the two are in proportion of their volume. The masses of cone and hemisphere are
Mass of cone is m1=ρ13πR2h
and that of hemisphere is m2=ρ23πR3
Now we apply the result of two body system to find the centre of mass of the composite body. Let l be the distance between the independent centre of mass of the bodies cone and hemisphere, then
l=3R8=h4
The position of centre of mass m2 is
x=m1lm1+m2=ρ13πR2h(3R8=h4)ρ13πR2h+ρ23πR3
x=h(3R+2h)8(h+2R)
Topper's Solved these Questions
- CENTRE OF MASS & MOMENTUM CONSERVATIONBook:BANSALChapter:CENTRE OF MASS & MOMENTUM CONSERVATIONExercise:Practice Exercise
Explore 20 Videos
- CENTRE OF MASS & MOMENTUM CONSERVATIONBook:BANSALChapter:CENTRE OF MASS & MOMENTUM CONSERVATIONExercise:EXERCISE-1 [SINGLE CORRECT CHOICE TYPE]
Explore 23 Videos
- CENTRE OF MASS & MOMENTUM CONSERVATIONBook:BANSALChapter:CENTRE OF MASS & MOMENTUM CONSERVATIONExercise:EXERCISE-4 (SECTION-B) (JEE-ADVANCED Previous Year Questions)
Explore 8 Videos
- GRAVITATIONBook:BANSALChapter:GRAVITATIONExercise:EXERCISE -4 Section - B
Explore 6 Videos
Similar Questions
Find out the centre of mass of a composite object shown in figure-4.28. Object consists of a cone with its base joint with the base of a hemisphere. The dimensions of the object are shown in figure. Assume uniform density of the system, find the centre of mass of this system.

View SolutionFindout the linear length of the image of the object shown in figure.
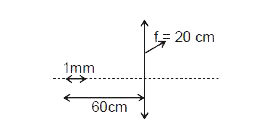
View SolutionFind the position of centre of mass of the section shown in figure

View SolutionFindout the linear length of the image of the object AB shown in figure.
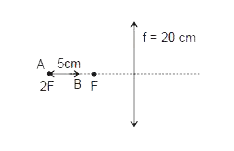
View SolutionFind out the position of centre of mass of the figure shown below.
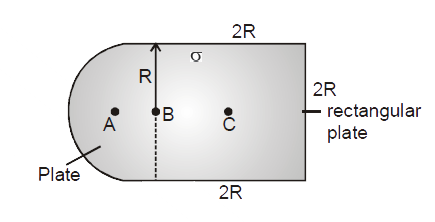
View SolutionFind the velocity of center of mass of the system shown in the figure.
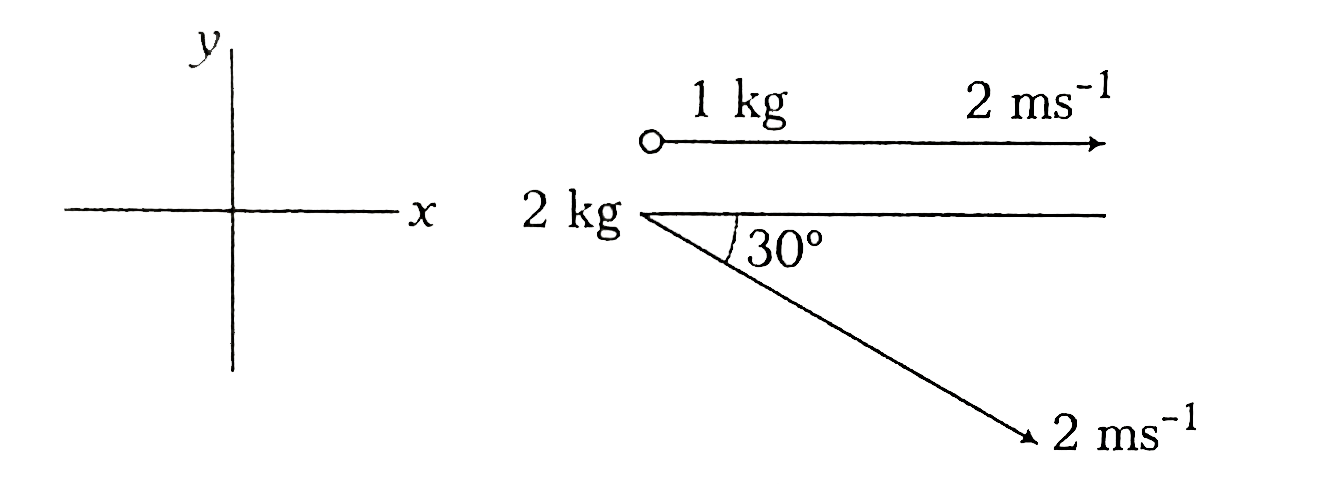
View SolutionFind the velocity of the center of mass of the system shown in the figure .
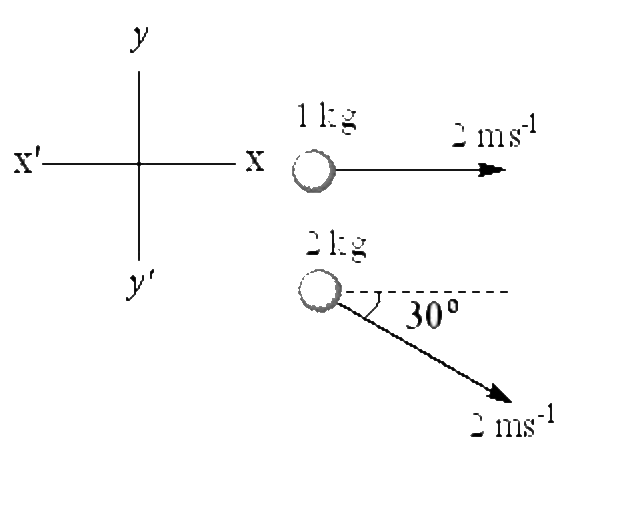
View SolutionFind out the position of centre of mass of the uniform lamina as shown in figure.
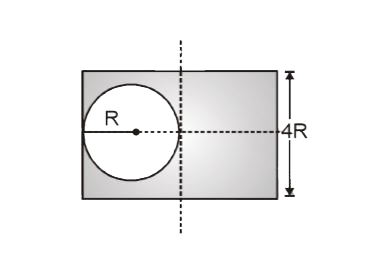
View SolutionFind the position of centre of mass of the uniform lamina shown in figure.

View SolutionThe coordinate of the centre of mass of a system as shown in figure : -
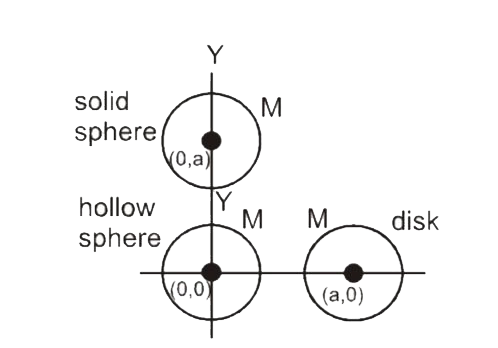
View Solution