A uniform rod of mass M and length L is held vertically upright on a horizontal surface as shown in figure. Assuming zero potential energy at the base of the rod, determine the potential energy of the rod.
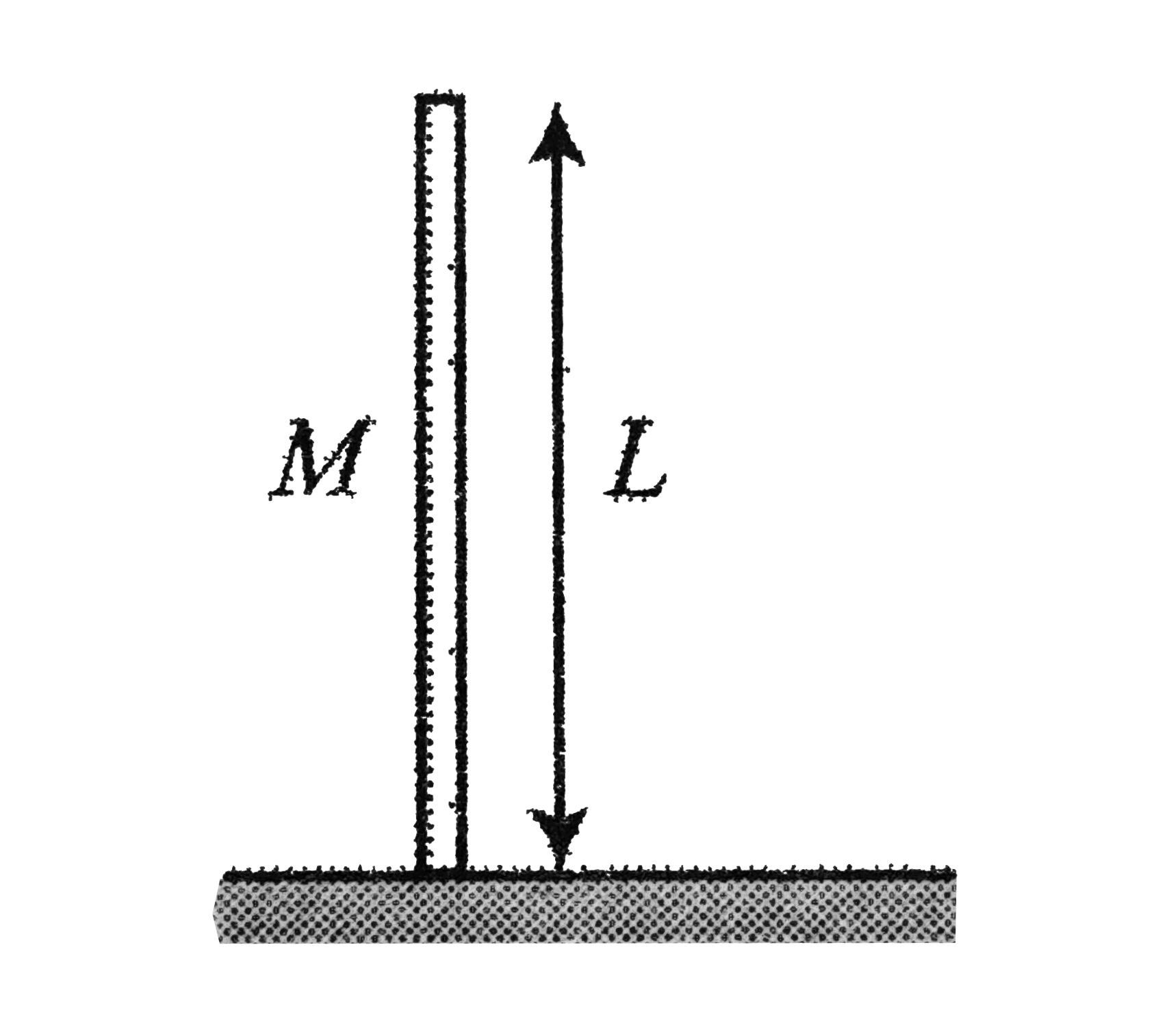
We can not write the potential energy of the rod directly. All the points of the rod are situated at different height from the ground. Therefore we divide it into many point masses with potential energy of a small mass being dU,
dU=(dm)gy
Total P.E. would be the summation of P.E. of all elements frem y=0 to y=l.
⇒ ∫l0dm g y
Mass of small element, of length dy is dm:
m is mass of length l,
∴ mass of unit length =ml
mass of length dy =mldy
dm=mldy
U=g∫l0yd.y
=mgl∫l0ydy =mgl×(l22)
=mg(12)
In this case, rather than solving this problem, we could have said that the mass of the rod is concentrated at some height Y from the ground. And we shall replace the rod with a point mass at that height.
To determine that height y, compare U with Mg Y
U=MgY=mg(1)2
we get Y=12
If we had known this position earlier, we could have solved the problem in no time without integration. This position Y is the center of mass of the rod. Such problem would have been really cumbersome for more complicated bodies like ring, disc, sphere, cone etc. but if their centre of mass is known, we could have solved them easily without calculations. Potential energy was an example, there are many more physical quantities of a system of particles which can be calculated by this concept of centre of centre of mass. Now we shall explore the concept of centre of mass in detail calculation of its position and application on physical quantities.
Topper's Solved these Questions
- CENTRE OF MASS & MOMENTUM CONSERVATIONBOOK - BANSALCHAPTER - CENTRE OF MASS & MOMENTUM CONSERVATIONEXERCISE - Solved Examples
15 Videos
- CENTRE OF MASS & MOMENTUM CONSERVATIONBOOK - BANSALCHAPTER - CENTRE OF MASS & MOMENTUM CONSERVATIONEXERCISE - Practice Exercise
20 Videos
- GRAVITATIONBOOK - BANSALCHAPTER - GRAVITATIONEXERCISE - EXERCISE -4 Section - B
6 Videos
Similar Questions
A uniform rod of mass M and length L is standing vertically along the y-axis on a smooth horizontal surface as shown. A small disturbance causes the lower end to slip on the smooth horizontal surface and rod starts falling. The path followed by centre of mass of the rod during the fall is
View SolutionA uniform rod AB of mass m and length l is at rest on a smooth horizontal surface. An impulse J is applied to the end B perpendicular to the rod in horizontal direction. Speed of the point A of the rod after giving impulse is:
View Solution
Knowledge Check
A uniform rod of mass M and length L, area of cross section A is placed on a smooth horizontal surface. Forces acting on the rod are shown in the digram

Total elastic potential energy stored in the rod is :A7F2L6AYB11F2L6AYC5F2L6AYD3F2L2AY
A uniform rod AB of mass m and length L rotates about a fixed vertical axis making a constant angle θ with it as shown in figure. The rod is rotated about this axis, so that point B the free end of the rod moves with a uniform speed V in the horizontal plane then the angular momentum of the rod about the axis is:A12mVLcosθB13mVLsinθCmVLsincosθDzeroA rod of mass M and length L is made to stand vertically. Potential energy of the rod in this position is
AMglBMgl2CMgl3DMgl4
A uniform rod of mass M and length L lies flat on a frictionless horizantal surface. Two forces F and 2F are applied along the length of the rod as shown. The tension in the rod at point P is

View SolutionA uniform rod of mass M and length L, area of cross section A is placed on a smooth horizontal surface. Forces acting on the rod are shown in the digram

Ratio of elastic potential energy stored in section PQ and section QR of the rod is
View SolutionA uniform rod of mass m, length l rests on a smooth horizontal surface. Rod is given a sharp horizontal impulse p perpendicular to the rod at a distance l/4 from the centre. The angular velocity of the rod will be
View SolutionA rod mass (M) hinged at (O) is kept in equilibrium with a spring of stiffness (k) as shown in figure. The potential energy stored in the spring is .

View SolutionA uniform rod of mass m and length l is at rest on smooth horizontal surface. An impulse P is applied to end B as shown.

Distance travelled by the centre of the rod, while rod rotates by 90∘
View Solution
