A road passes at some distance from a standing man. A truck is coming on the road with some acceleration. The truck driver blows a whistle of frequency 500Hz when the line joining the truck and the man makes an angle θ with the road. The man hears a note having a note having a frequency of 600Hz when the truck is closest to him. Also the speed of truck has git doubled during this time. Find the value of 'θ'.
The correct Answer is:θ=60∘
Let the truck velocity be vS
then, 600=(vv−vScosθ)500……(i)
As 2vS=vS+at
vS=at …(ii)
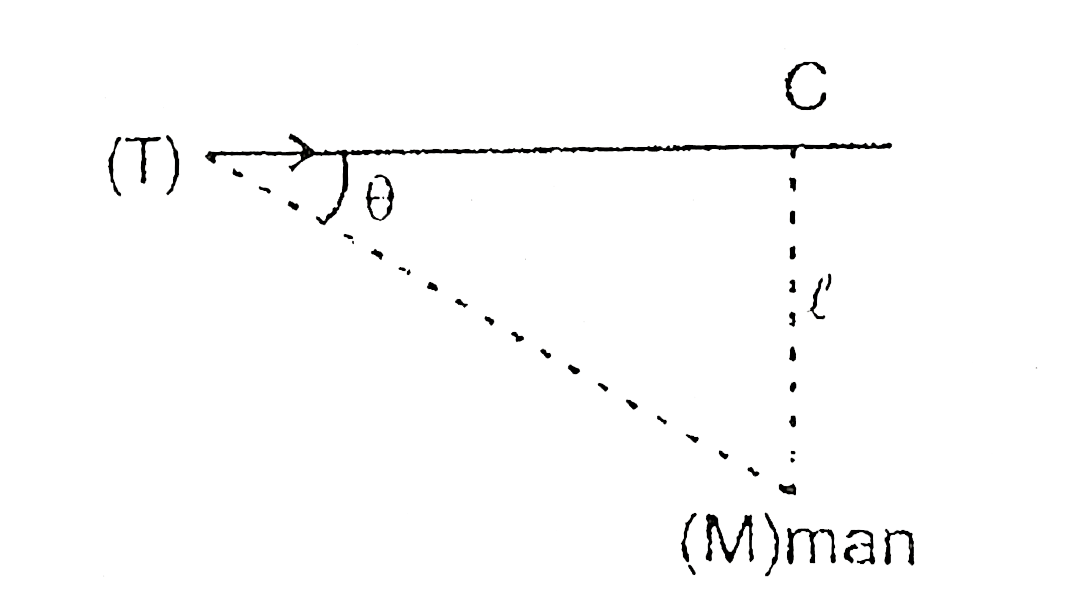
where 'a' is accelertion.
As vt=lsinθ
where v is velocity is sound.
Also, lcotθ=vSt+12at2
lcosθsinθ=32at2 (from eqn (ii))
lcosθsinθ=32a(lvsinθ)2
alv=(23sinθcosθ)v ......(iii)
from (i)
65=vv−atcosθ=vv−alvsinθcosθ
Put alv from (iii)
∴θ=60∘
Topper's Solved these Questions
- SOUND WAVESBOOK - RESONANCECHAPTER - SOUND WAVESEXERCISE - Exercise- 3 PART - II
1 Video
- SOUND WAVESBOOK - RESONANCECHAPTER - SOUND WAVESEXERCISE - Exercise- 2 PART - IV
8 Videos
- SIMPLE HARMONIC MOTIONBOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise
28 Videos
- STRING WAVESBOOK - RESONANCECHAPTER - STRING WAVESEXERCISE - Exercise
32 Videos
Similar Questions
Does a man standing at rest on a moving truck possess KE?
View SolutionA policemen blows a whistle of frequency 330 Hz as a car speeds and passed him with avelocity 18 km per hour. Find the change in frequency as heard by the driver of the car just as he passes the police man (Velocity of sound = 320 m//s)
View Solution
Knowledge Check
A truck accelerates from speed v to 2v. Work done during this is
Athree times as the work done in accelerating it form rest to vBsame as the work done in accelerating it form rest to vCfour times as the work done in acceleration it from rest to vDless than the work done in acclerating it from rest to vA truck accelerates from speed v to 2v. Work done during this is
Athree times as the work done in accelerating it from rest to v.Bsame as the work done in accelerating it from rest to v.Cfour times as the work done in accelerating it from rest to v.Dless than the work done in accelerating it from rest to vAn engine is moving on a circular track with a constant speed. It is blowing a whistle of frequency 500Hz. The frequency received by an observer standing stationary at the centre of the track is
A500HzBmore than 500hzCless than 500HzDmore or less than 500Hz depending on the actual speed of the engine
An engine standing at the platform blows a whistle of frequency 305 Hz. If the velocity of sound be 1220 km/h, the frequency of the whistle as heard by a man running towards the engine with a speed of 20 km/s is
View SolutionA truck crosses a man moving along the road at 6 km/hr. The man could see the truck upto 2 min find the speed of the truck, if at the time of disappearance the distance of truck to man is 1.2 km
View SolutionA truck covers a distance of 1200 km in 40 hours. What is the average speed of the truck?
View SolutionA truck covers a distance of 1800 km in 40 hours. What is the average speed of the truck?
View SolutionA truck accelerates from speed v to 2v. Work done in during this is
View Solution