A source is moving across a circle given by the equation x2+y2=R2 with constant speed vS=330π6√3m/s. In clockwise sense. A detector is stationary at the point (2R,0)w.r.t. the centre of the circle. The frequency emitted by the source is fS.
(a) What are the co-ordinates of the source when the detector records the maximum and minimum frequencies. Take speed of sound v=330m/s.
The correct Answer is:(√3R2,R2),(√3R2,−R2)<br>f'min<br>=(6sqrt(3))/(6sqrt(3)+pi)f_(S) , f'_(max) = (6sqrt(3))/(6sqrt(3)-pi)f_(S)

Wave emiited at A when reach at detector gives maximum apparent frequency during this time source reaches at E.
Time taken by sound to reach at C=R√3v
Distance treavelled by the source within this time =R√3v330π6√3=55πRv
Hence θ'=55πRvR=π6
∴ Angle from horizontal =30∘
Hence coordinates (R√32±R2)
Also , n'min=vv+vSf
n'max=vv−vSf
Topper's Solved these Questions
- SOUND WAVESBOOK - RESONANCECHAPTER - SOUND WAVESEXERCISE - Exercise- 3 PART - II
1 Video
- SOUND WAVESBOOK - RESONANCECHAPTER - SOUND WAVESEXERCISE - Exercise- 2 PART - IV
8 Videos
- SIMPLE HARMONIC MOTIONBOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise
28 Videos
- STRING WAVESBOOK - RESONANCECHAPTER - STRING WAVESEXERCISE - Exercise
32 Videos
Similar Questions
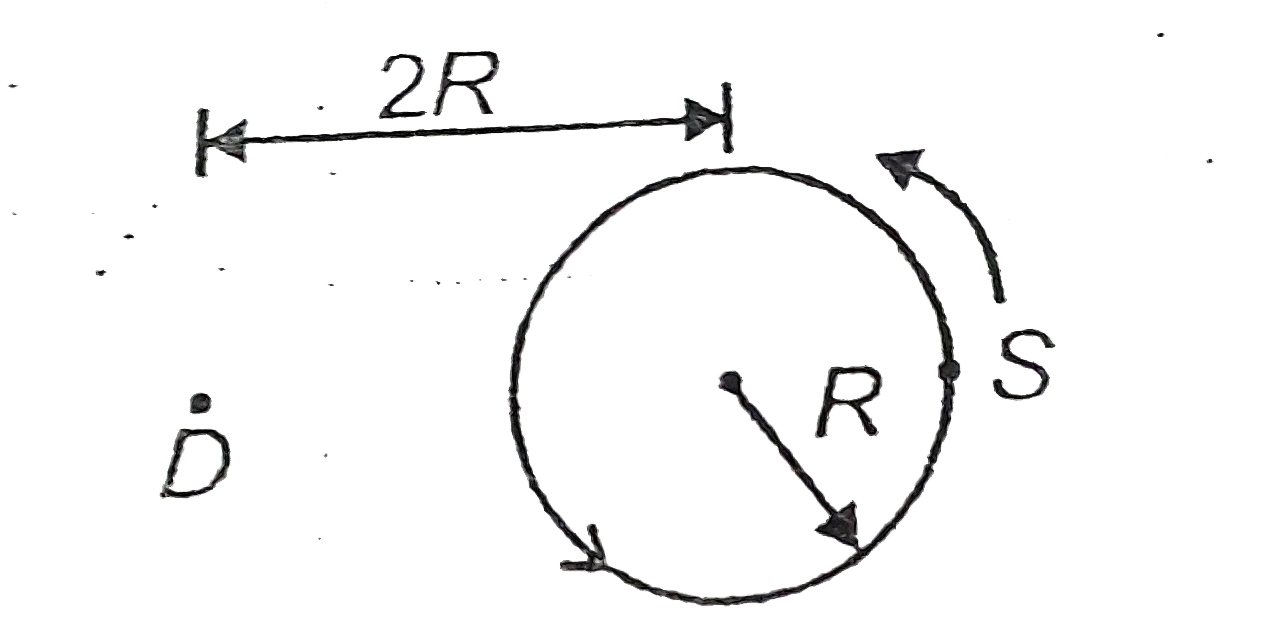
A whistle 'S' of frequency v revolves in a circle of radius R at a constant speed v. what is the ratio of the largest and smallest frequency detected by a detector D, at rest, at a distance 2 R from the centre of the circle as shown in the figure? (take speed of sound in air as c)
View SolutionA source of sound emitting a 1200 Hz note travels along a straight line at a speed of 170ms−1. A detector is placed at a distance of 200 m from the line of motion of the source. (a) Find the frequency of sound received by the detector at the instant when the source gets closest to it. (b) Find the distance between the source and the detector at the instant it detects the frequency 1200 Hz. Velocity of sound in air=340ms−1.
View Solution
Knowledge Check
When the observer moves towards the stationary source with velocity, v1, the apparent frequency of emitted note is f1. When the observer moves away from the source with velocity v1, the apparent frequency is f2. If v is the velocity of sound in air and f1f2 = 2,then vv1 = ?
A2B3C4D5A detector is released from rest over a source of sound of frequency f0=103Hz. The frequency observed by the detector at time t is plotted in the graph. The speed of sound in air is (g=10m/s2)
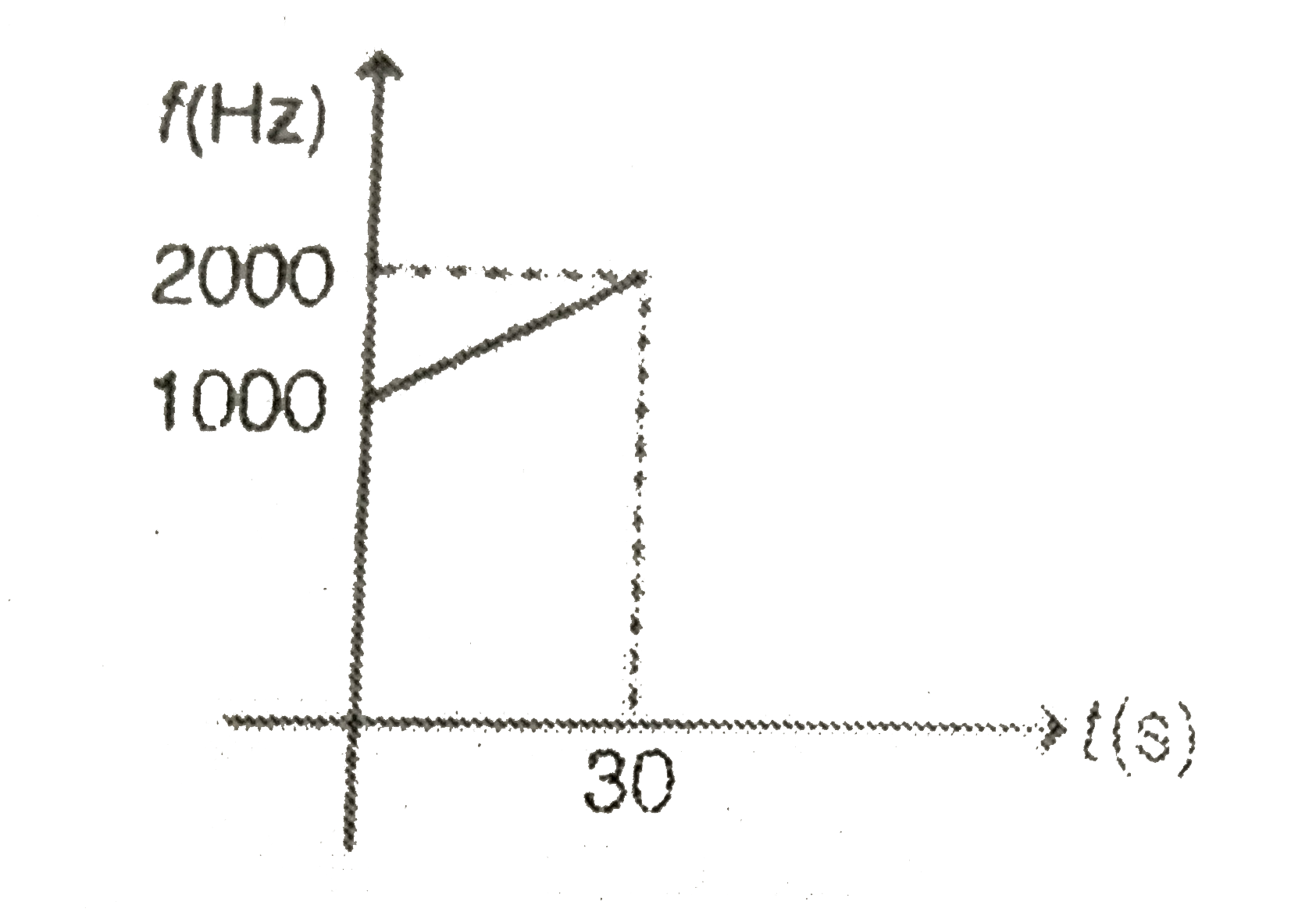 A330m/sB350 m/sC300 m/sD310 m/s
A330m/sB350 m/sC300 m/sD310 m/sA soure of sound of frequency 165 hz is placed in front of a wall at a distance 2 m from it. A dtector is also placed in front of the wall at the same distance from it. Find the minimum distance between the source and detector for which maximum sound is recorded int he detector . the speed of sound is 330 m/s
A4mB3 mC1 mD2m
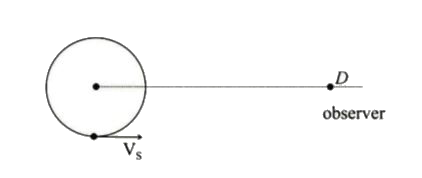
A source of sound is moving with a constant speed of 20 m s−1 in a circle. There is a stationary observer at some distance greater than the radius of the circle from the centre of circle as shown in the fiugre. Find the difference between the maximum and minimum frequency (in Hz) observed by the observer. (Assume speed of sound to be 340 m s−1 and frequency of the source is 360 Hz)
View SolutionA detector is released from rest over height h a source of sound of frequency f0=103Hz. The frequency observed by the detector at time t is plotted in the graph. The speed of sound in air is (g=10m/s2)
_E01_060_Q01.png)
View SolutionA source emitting sound of frequency 180Hz is placed in front of a wall at distance of 2m from it . A detector is also placed in front of the wall at the same distance from it. Find the minimum distance between the source and the detector for which the detector detects a maximum of sound. Speed of sound in air =360m/s.
View Solution
Two sources S1 and S2 of same frequency f emits sound. The sources are moving as shown with speed u each. A stationary observer hears that sound. The beat frequency is (v=velocity sound)
View SolutionAt t=0 , observer and source are at same place. Now the source is projected with velocity 60√2m/s at 45∘ . Natural frequency of source is 1000 Hz.find the frequency heard by the observer at t=2s. Take speed of sound = 340 m/s
View Solution
