A cylinder of 150mm radius rotates concentrically inside a fixed cylinder of 155mm radius. Both culinders are 300mm long. Determine the viscosity of the liquid which fills the space between the cylinders if a torque of 0.98n−m is required to maintain an angular velocity of 60r.p.m.

The correct Answer is:η=0.77N−sec/m2
Stress in wire B=mg3πr2B
Stress in wire B=4mg3πr2A
if mg3πr2B=4mg3πr2A either wire will break.
Topper's Solved these Questions
- ELASTICITY AND VISCOCITYBOOK - RESONANCECHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Exercise- 3 PART - IV
9 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 92 illustration
2 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise
43 Videos
Similar Questions
A cylinder of mass M, radius r1 and lenglth l is kept inside another cylinder of radius r2 and length l. The space between them is filled with a liquid of viscosity η. The inner cylinder starts rotating with angular velocity ω while the other cylinder is at rest. Find time when inner cylinder stops.
View SolutionA solid cylinder of mass 20kg rotates about its axis with angular velocity of 100 radian s−1. The radius of the cylinder is 0.25m. The magnitude of the angular momentum of the cylinder about its axis of rotation is
View Solution
Knowledge Check
A solid cylinder of radius 0.5 m and mass 50 kg is rotating at 300 rpm. Then the torque which will bring it to rest in 5 seconds is
A25πNmB25π2NmC50πNmD100πNmA small sphere of mass m and radius r is placed inside a horizontal hollow cylinder of radius R. Now cylinder rotating with some angular acceleration which slowly increases from zero to certain value α.
 AMaximum angle θ formed by line joining centre will be θ=sin−1(2αR5g)BAngular velocity of sphere will be ω′=ωrR where ω is angular velocity of cylinderCSphere will keep rotating at lowest positionDK.E. of sphere becomes constant when it reaches maximum height
AMaximum angle θ formed by line joining centre will be θ=sin−1(2αR5g)BAngular velocity of sphere will be ω′=ωrR where ω is angular velocity of cylinderCSphere will keep rotating at lowest positionDK.E. of sphere becomes constant when it reaches maximum heightThe axis of the uniform cylinder in figure is fixed. The cylinder is initially at rest. The block of mass M is initially moving to the friction and with speed v1. It passes over the cylinder to the dashed position. When it first makes contact with the cylinder, it slips on the cylinder, but the friciton is large enough so that slipping ceases before M loses contacts wtih the cylinder. the cylinder has a radius R and a rotaitonal intertia I
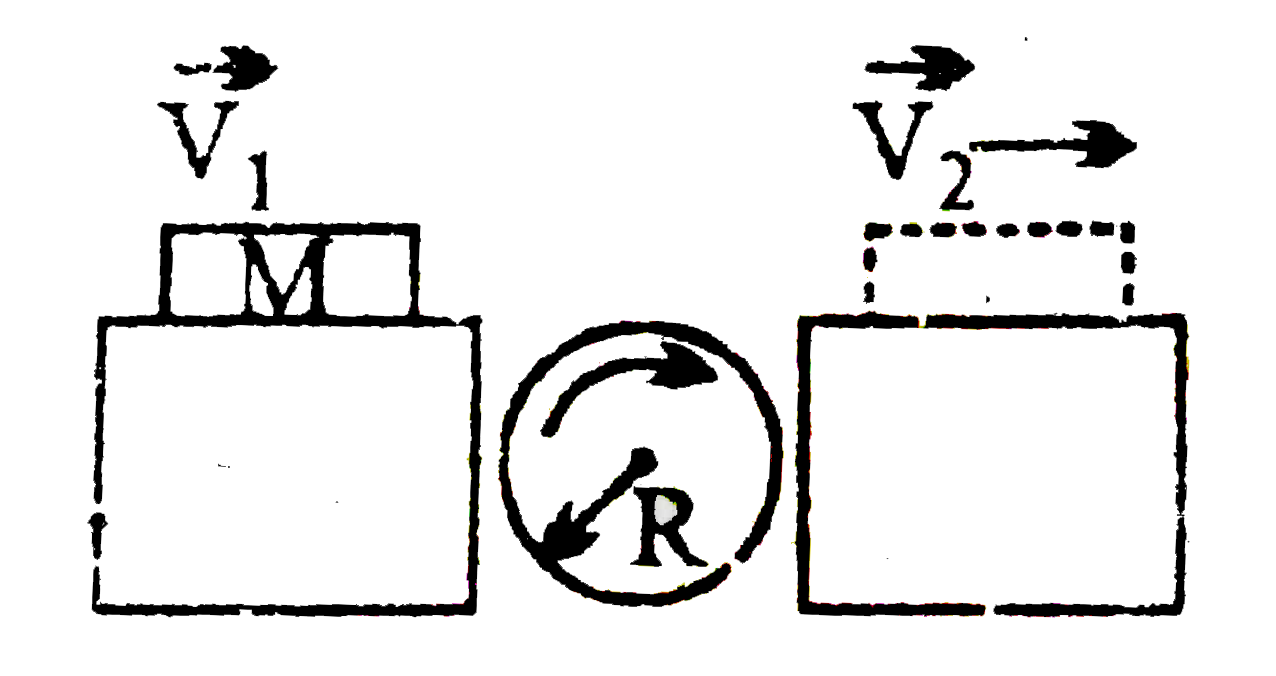
If ω is final angular velocity of the cylinder, thenAv1=ωRBv2=ωRCv1<ωRDnone
A long cylinder of radius R1 is displaced along its axis with a constant velocity v0 inside a stationary co-axial cylinder of radius R2. The space between the cylinders is filled with viscous liquid. Find the velocity of the liquid as a function of the distance r from the axis of the cylinders. The flow is laminar.
View SolutionA light thread with a body of mass m tied to its end is wound on a uniform solid cylinder of mass M and radius R. At a moment t=0 the system is set in motion. Assuming the friction in the axle of the cylinder to be negligible, find the time dependence of
(a) the angular velocity of the cylinder and
(b) the kinetic energy of the whole system ( M=2m).

View SolutionA solid cylinder of mass 'M' and radius 'R' is rotating along its axis with angular velocity ω without friction. A particle of mass 'm' moving with velocity v collides aganist the cylinder and sticks to its rim. After the impact calculate angular velocity of cylinder .
View SolutionA particle of mass m moves along the internal smooth surface of a vertical cylinder of the radius R. Find the force with which the particle acts on the cylinder wall if at the initial moment of time its velocity equals v0. And forms an angle α with the horizontal.
View Solution
Consider a disk of mass m, radius R lying on a liquid layer of thickness T and coefficient of viscosity η as shown in the fig.
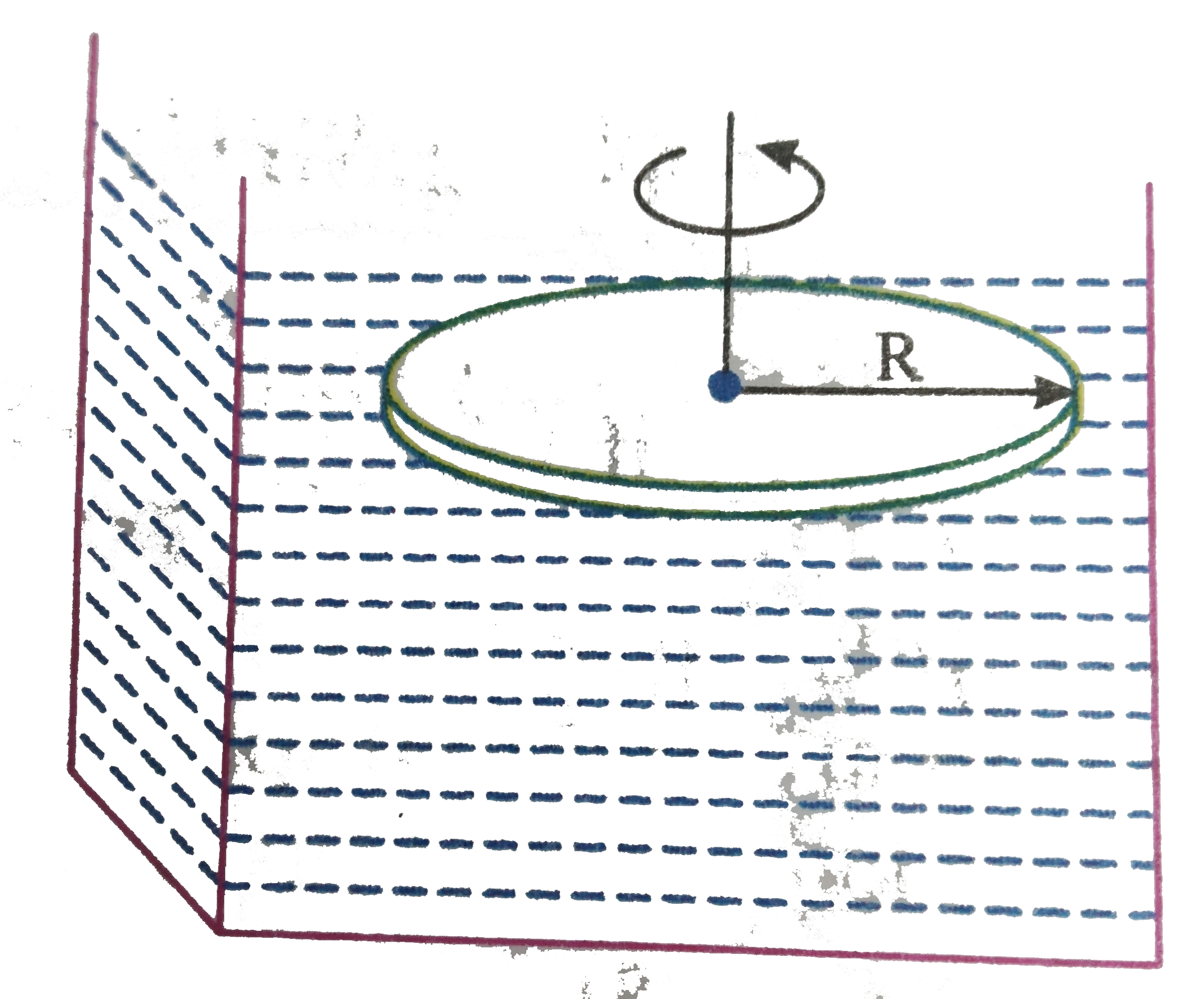
The torque required to rotate the disk at a constant angular velocity Ω given the viscosity is uniformly η.
View Solution
