A non conducting ring of radius R1 is charged such that the linear charge density is λ1cos2θ where θ is the polar angle. If the radius is increased to R2 keeping the charge constant, the linear charge density is changed to λ2cos2θ. The relation connecting R1, R2λ1 and λ2 will be
λ1/R1=λ2/R2
λ1/R2=λ2/R1
λ1λ2=R1R2
λ21R1=λ22R2
To solve the problem, we need to establish a relationship between the initial and final linear charge densities and the corresponding radii of the non-conducting ring.
1. Understanding the Charge Distribution:
The initial linear charge density on the ring is given by:
λ1=λ1cos2θ
where θ is the polar angle.
2. Calculating the Total Charge:
The total charge Q on the ring can be calculated by integrating the charge element dq around the ring. The charge element dq for a small segment of the ring at angle θ is given by:
dq=λ1⋅(R1dθ)=λ1cos2θ⋅(R1dθ)
3. Integrating Over the Full Ring:
To find the total charge Q, we integrate dq from 0 to 2π:
Q=∫2π0λ1R1cos2θdθ
We can factor out λ1R1:
Q=λ1R1∫2π0cos2θdθ
4. Evaluating the Integral:
The integral of cos2θ over one full cycle can be computed using the identity:
cos2θ=1+cos(2θ)2
Therefore:
∫2π0cos2θdθ=∫2π01+cos(2θ)2dθ=12(θ+sin(2θ)2)2π0=12(2π)=π
5. Substituting Back:
Now substituting back, we find:
Q=λ1R1⋅π
6. Changing the Radius:
When the radius is changed to R2 and the linear charge density changes to λ2, the total charge remains constant:
Q=λ2R2⋅π
7. Setting the Two Expressions for Charge Equal:
Since the total charge remains constant, we can set the two expressions for Q equal to each other:
λ1R1π=λ2R2π
8. Simplifying the Equation:
Dividing both sides by π:
λ1R1=λ2R2
9. Final Relation:
Rearranging gives us the final relation:
λ1λ2=R2R1
Final Answer:
The relation connecting R1, R2, λ1, and λ2 is:
λ1R1=λ2R2
The initial linear charge density on the ring is given by:
λ1=λ1cos2θ
where θ is the polar angle.
The total charge Q on the ring can be calculated by integrating the charge element dq around the ring. The charge element dq for a small segment of the ring at angle θ is given by:
dq=λ1⋅(R1dθ)=λ1cos2θ⋅(R1dθ)
To find the total charge Q, we integrate dq from 0 to 2π:
Q=∫2π0λ1R1cos2θdθ
We can factor out λ1R1:
Q=λ1R1∫2π0cos2θdθ
The integral of cos2θ over one full cycle can be computed using the identity:
cos2θ=1+cos(2θ)2
Therefore:
∫2π0cos2θdθ=∫2π01+cos(2θ)2dθ=12(θ+sin(2θ)2)2π0=12(2π)=π
Now substituting back, we find:
Q=λ1R1⋅π
When the radius is changed to R2 and the linear charge density changes to λ2, the total charge remains constant:
Q=λ2R2⋅π
Since the total charge remains constant, we can set the two expressions for Q equal to each other:
λ1R1π=λ2R2π
Dividing both sides by π:
λ1R1=λ2R2
Rearranging gives us the final relation:
λ1λ2=R2R1
The relation connecting R1, R2, λ1, and λ2 is:
λ1R1=λ2R2
Topper's Solved these Questions
- GMP ASSESMENTBook:FIITJEEChapter:GMP ASSESMENTExercise:Assertion & Reason
Explore 15 Videos
- GMP ASSESMENTBook:FIITJEEChapter:GMP ASSESMENTExercise:Multiple Question
Explore 58 Videos
- GMP ASSESMENTBook:FIITJEEChapter:GMP ASSESMENTExercise:Numerical Based
Explore 61 Videos
- GENERAL PHYSICSBook:FIITJEEChapter:GENERAL PHYSICSExercise:MATRIX MATCH TYPE
Explore 3 Videos
- GRAVITATIONBook:FIITJEEChapter:GRAVITATIONExercise:Numerical based Question
Explore 2 Videos
Similar Questions
Knowledge Check
A thin non-conducting ring of radius R has a linear charge density λ=λ0cosθ, where θ is measured as shown. The total electric dipole moment of the charge distribution is
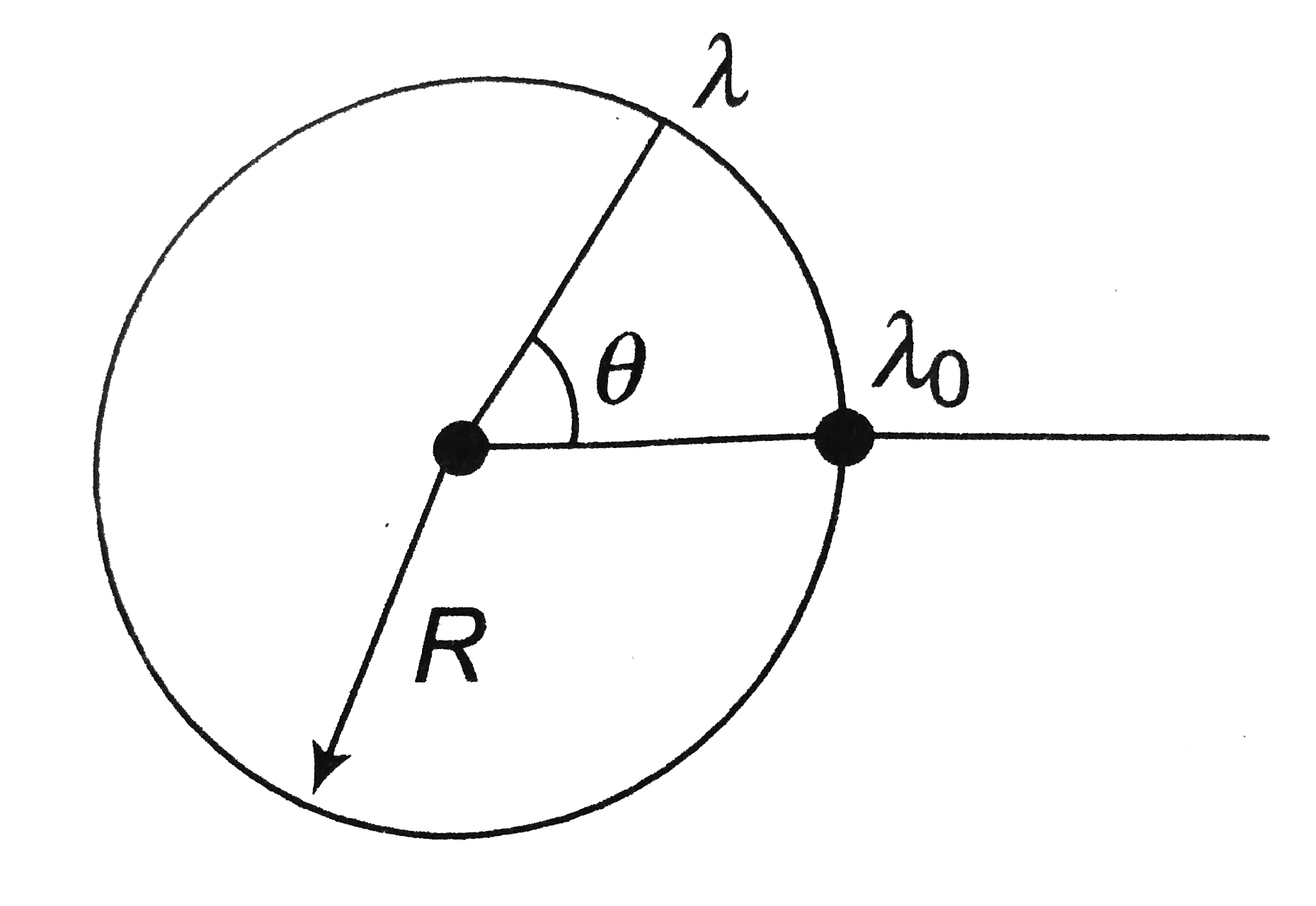 AR2λ0B2πR2λ0CπR2λ02DπR2λ0
AR2λ0B2πR2λ0CπR2λ02DπR2λ0A half ring of radius r has a linear charge density λ.The potential at the centre of the half ring is
Aλ4εoln0Bλ4π2ε0rCλ4πε0rDλ4πε0r2Charge over a nonconducting ring is distributed so that the linear charge density varies as λ=λ0sinθ. What is the direction of force on a charge q0 placed at the center?
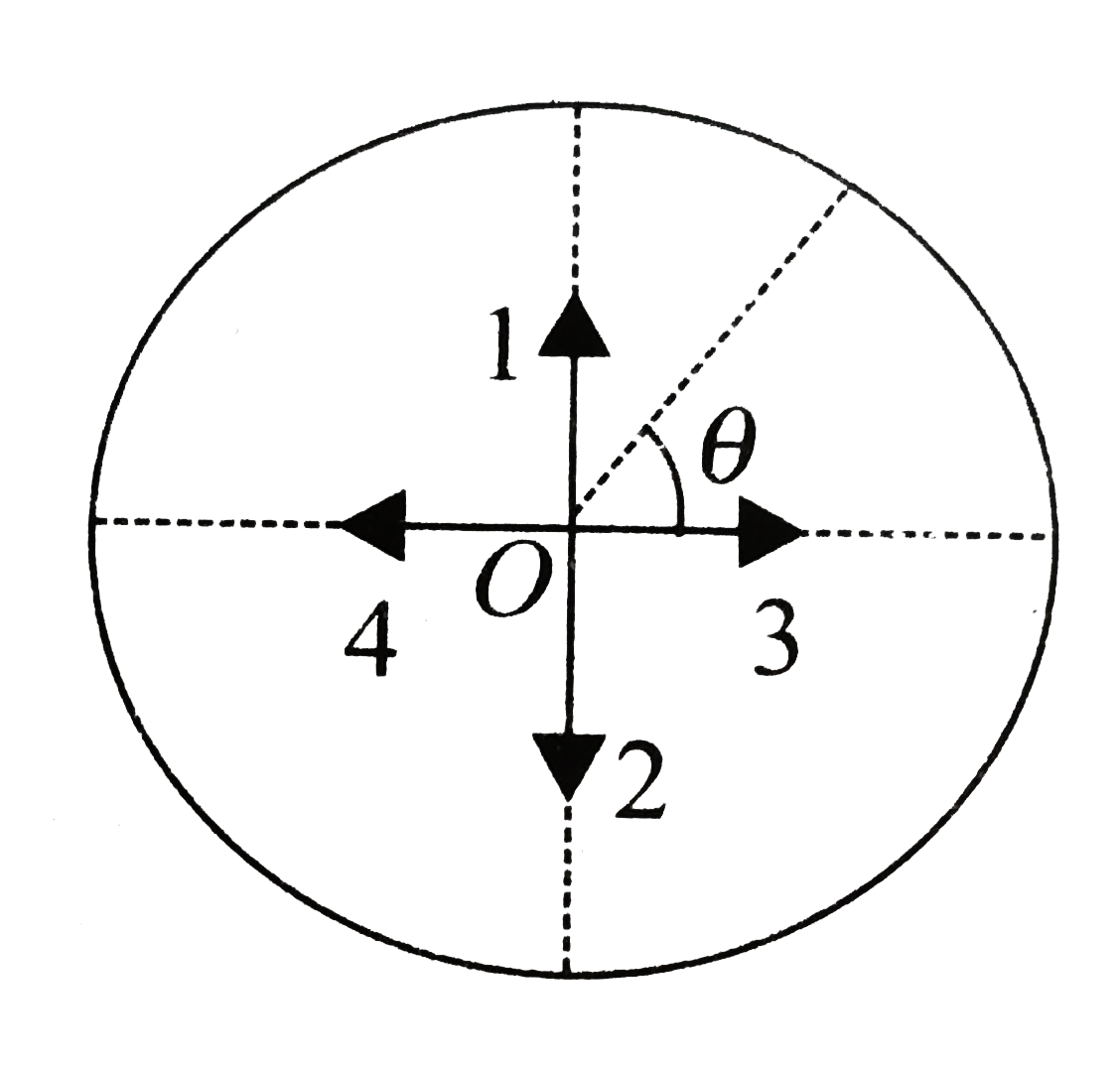 Aalong 1 if q0 is positive and 2 if q0 is negative.Balong 2 if q0 is positive and 1 if q0 is negative.Calong 3 if q0 is positive and 4 if q0 is negative.Dalong 4 if q0 is positive and 3 if q0 is negative.
Aalong 1 if q0 is positive and 2 if q0 is negative.Balong 2 if q0 is positive and 1 if q0 is negative.Calong 3 if q0 is positive and 4 if q0 is negative.Dalong 4 if q0 is positive and 3 if q0 is negative.
A thin nonconducting ring of radius r has a linear charge density q=q0cosθ, where q0 is a constant and θ is the angle at the centre from the diameter of maximum charge density in the anticlockwise direction. Find the electric field at the centre of the ring.
View SolutionTwo conducting spheres of radii r1 and r2 are charged to the same surface charge density . The ratio of electric field near their surface is
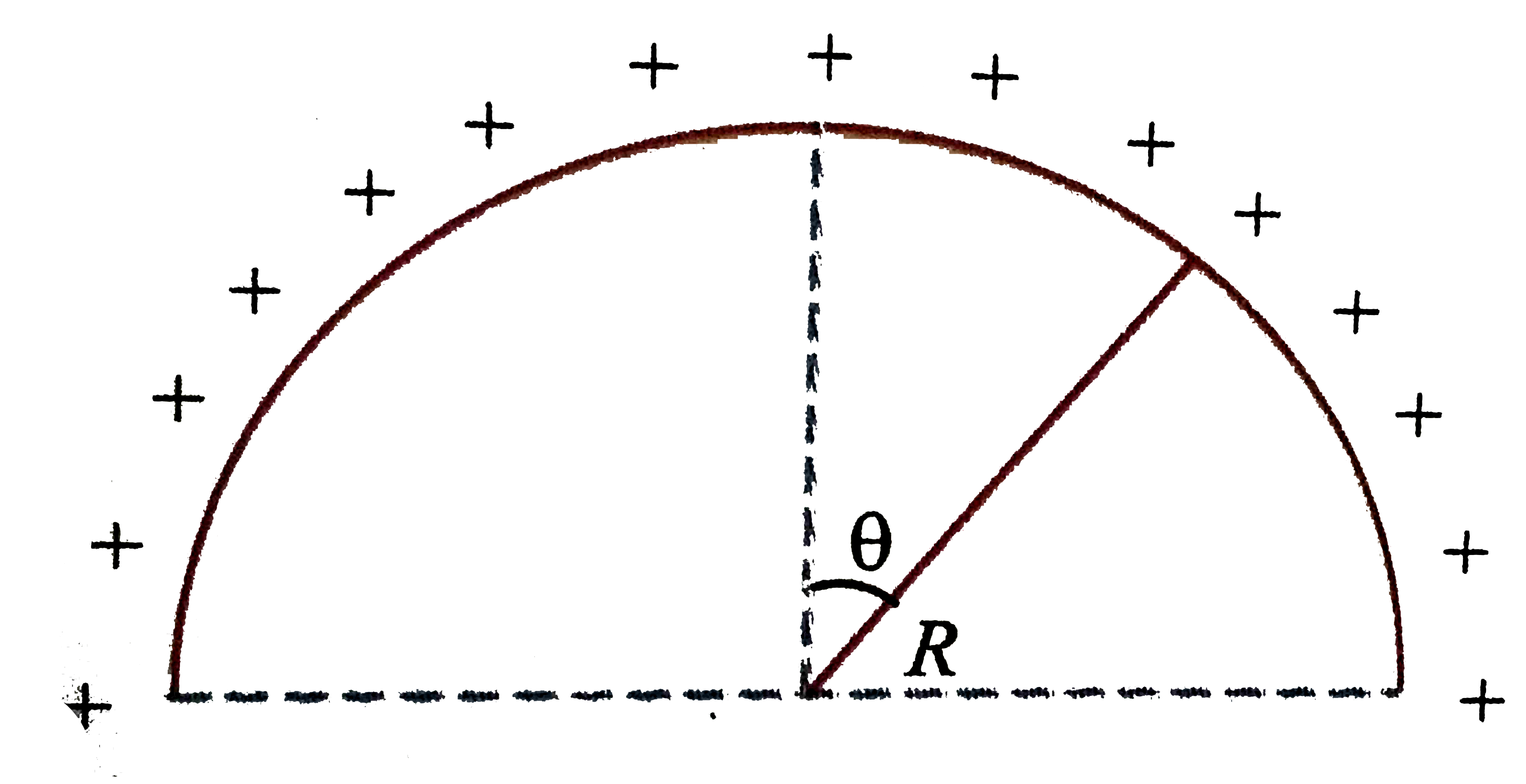
View SolutionThe linear charge density of a uniform semicircular wire varies with θ shown in the figure as λ=λ0cosθ if R is the radius of the loop ,of the charge on the wire is
View Solutionthe linear charge density of a thin metallic rod varies with the distance x from the end as λ=λ0x2(0≤x≤l) The total charge on the rod is
View SolutionAn arc of radius r carries charge. The linear density of charge is λ and the arc subtends an angle π3 at the centre.
View Solution