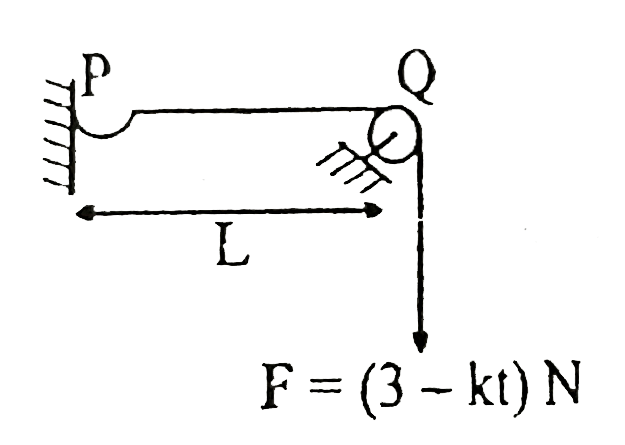
In the given figure a string of linear mass density kg//m and length m, is stretched by a froce , where k is a constant and t is time in sec. At the time , a pulse is generated at the end P of the string Find the value of k (in N//s) if the value of force becomes zero as the pulse reaches point Q.
The correct Answer is:
Time taken by pulse to reach from P to where
Now,
Topper's Solved these Questions
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Additional topic
70 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Multiple objective
131 Videos
- FLUID MECHANICSBOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE
10 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
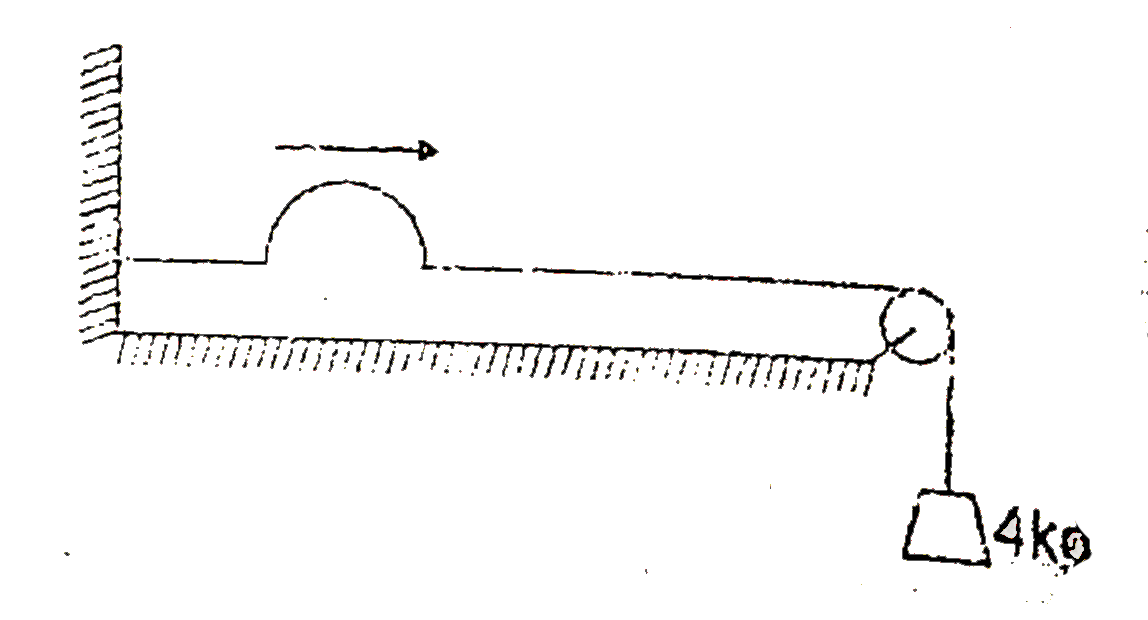
Figure shows a string of linear mass density 1.0g/cm on which a wave pulse is travelling. Find the time taken (in milli second) by the pulse in travelling through a distance of 60cm on the string. Take g=10m/s2.
View SolutionA way pulse is travelling on a string of linear mass density 6.4×10−3kgm−1 under a load of 80kgf. Calculate the time taken by the pulse to traverse the string, if its length is 0.7m.
View Solution
Knowledge Check
A tranverse wave propagating on a stretched string of linear density 3×10−4 kg per m is represented by the equation
y=0.2sin(1.5x+60t)
Where , x is in metre and t is in second. The tension in the string (in newton) isA0.24B0.48C1.2D1.8A 200Hz sinusoidal wave is travelling in the positive x-direction along a string with a linear mass density of 3.5×10−3kg/m and a tension of 35N. At time t=0, the point x=0 , has maximum displacement in the positive y-direction. Next when this point has zero displacement, the slope of the string is π/20. Which of the following expression represent (s) the displacement of string as a function of x(in metre) and t (in second)
Ay=0.025cos(200πt−2πx)By=0.5cos(200πt−2πx)Cy=0.025cos(100πt−10πx)Dy=0.5cos(100πt−10πx)A string of length 10.0 m and mass 1.25kg stretched with a tension of 50N. If a transverse pulse is created at one end of the string, how long does it take to reach the other end ?
A0.5sB1.0sC1.5sD2.0s
A non-uniform wire of length l and mass M has a variable linear mass density given by μ=kx, where x is distance from one end of wire and k is a constant. Find the time taken by a pulse starting at one end to reach the other end when the tension in the wire is T.
View SolutionA string of length 40 cm and weighing 10 g is attached to a spring at one end and to a fixed wall at the other end. The spring has a spring constant of 160Nm−1 and is stretched by 1-0 cm. If a wave pulse is produced on the string near the wall, how much time will it take to reach the spring ?
View SolutionA wire of density 7×103kgm3 ,length 1m and diameter 1mm is stretched by weight of 11kg .The frequency of the fundamental note is 10k .Find k
View SolutionTwo long strings A and B, each having linear mass density 1.2×10−2kgm−1, are stretched by different tensions 4.8 N and 7.5 N respectively and are kept parallel to each other with their left ends at x = 0. Wave pulses are produced on the strings at the left ends at t = 0 on string A and at t = 20 ms on string B. When and where will the pulse on B overtake that on A ?
View SolutionA string of length 0.4m and mass 10−2kg is clamped at one end . The tension in the string is 1.6N. The identical wave pulses are generated at the free end after regular interval of time , Δt . The minimum value of Δt , so that a constructive interference takes place between successive pulses is
View Solution
