A prism shaped styrofoam of density $ρ_{s t y r o f o a m} (< ρ_{w a t e r})$ is held completely submerged in water. It lies with its base horizontal. The base of foam is at depth $h_{0}$ below water surface and atmospheric pressure is $P_{0}$ Surface of water is open to atmosphere. Styrofoam prism is held in equilibrium by the string attached symmetrically as shown (Take $ρ_{s t y r o f o a m} = ρ_{f}, ρ_{w a t e r} = ρ_{w})$ .
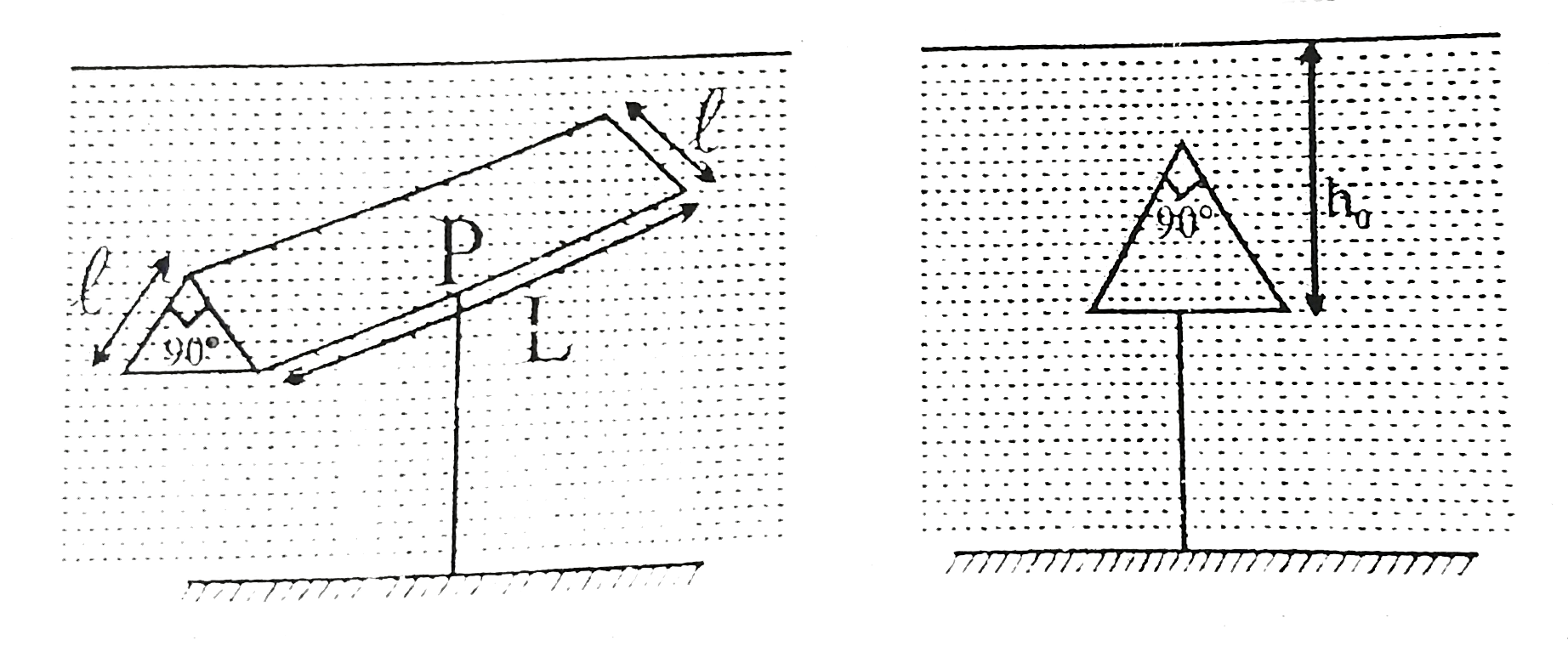Magnitude of force on any one of the slant face of styrofoam is

$(P_{0} + ρ_{w} g (h_{0} - \sqrt{2} l)) L l$

$(P_{0} + ρ_{w} g (h_{0} - \frac{l}{\sqrt{2}}) L l$

$(P_{0} - ρ_{w} g (h_{0} - \frac{l}{\sqrt{2}})) L l$

$(P_{0} + ρ_{w} g (h_{0} - \frac{l}{2 \sqrt{2}})) L l$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Average pressure on slant surface
$P_{a v g} = \frac{(P_{0} + ρ_{w} g h_{0}) + (P_{0} + ρ_{w} g h_{0} - \frac{1}{\sqrt{2}})}{2} = (P_{0} + ρ_{w} g h_{0} - \frac{l}{2 \sqrt{2}})$
Force on any one of the slant face
$= (P_{0} + ρ_{w} g (h_{0} - \frac{l}{2 \sqrt{2}}) L l$

Updated on:21/07/2023

Class 12 PHYSICS MASTER PRACTICE PROBLEM

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
A prism shaped styrofoam of density $ρ_{s t y r o f o a m} (< ρ_{w a t e r})$ is held completely submerged in water. It lies with its base horizontal. The base of foam is at depth $h_{0}$ below water surface and atmospheric pressure is $P_{0}$ Surface of water is open to atmosphere. Styrofoam prism is held in equilibrium by the string attached symmetrically as shown (Take $ρ_{s t y r o f o a m} = ρ_{f}, ρ_{w a t e r} = ρ_{w})$ .
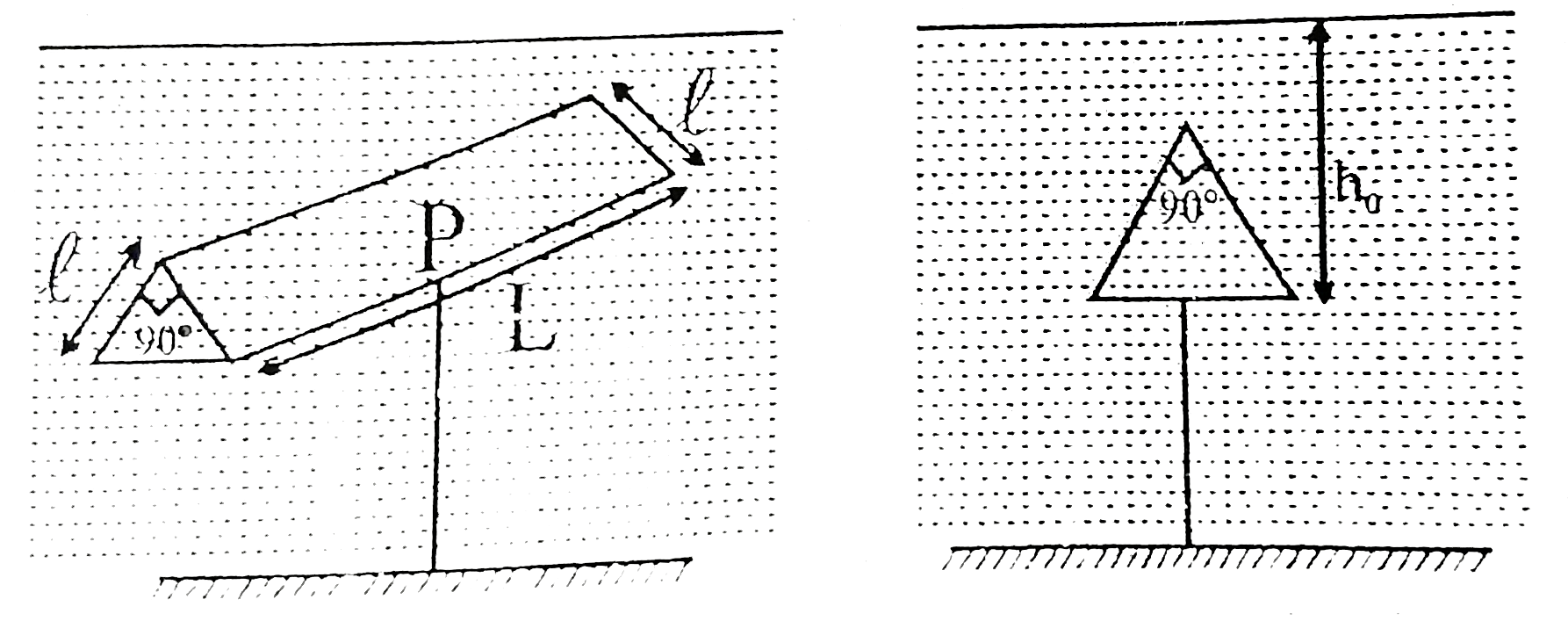Net force exerted by liquid on the styrofoam is
A $\sqrt{2} ρ_{w} g l^{2} L$
B $2 ρ_{w} g l^{2} L$
C $ρ_{w} \frac{g (l^{2} L)}{2}$
D $ρ_{w} g l^{2} L$
Question 2 - Select One or More
A prism shaped styrofoam of density $ρ_{s t y r o f o a m} (< ρ_{w a t e r})$ is held completely submerged in water. It lies with its base horizontal. The base of foam is at depth $h_{0}$ below water surface and atmospheric pressure is $P_{0}$ Surface of water is open to atmosphere. Styrofoam prism is held in equilibrium by the string attached symmetrically as shown (Take $ρ_{s t y r o f o a m} = ρ_{f}, ρ_{w a t e r} = ρ_{w})$ .
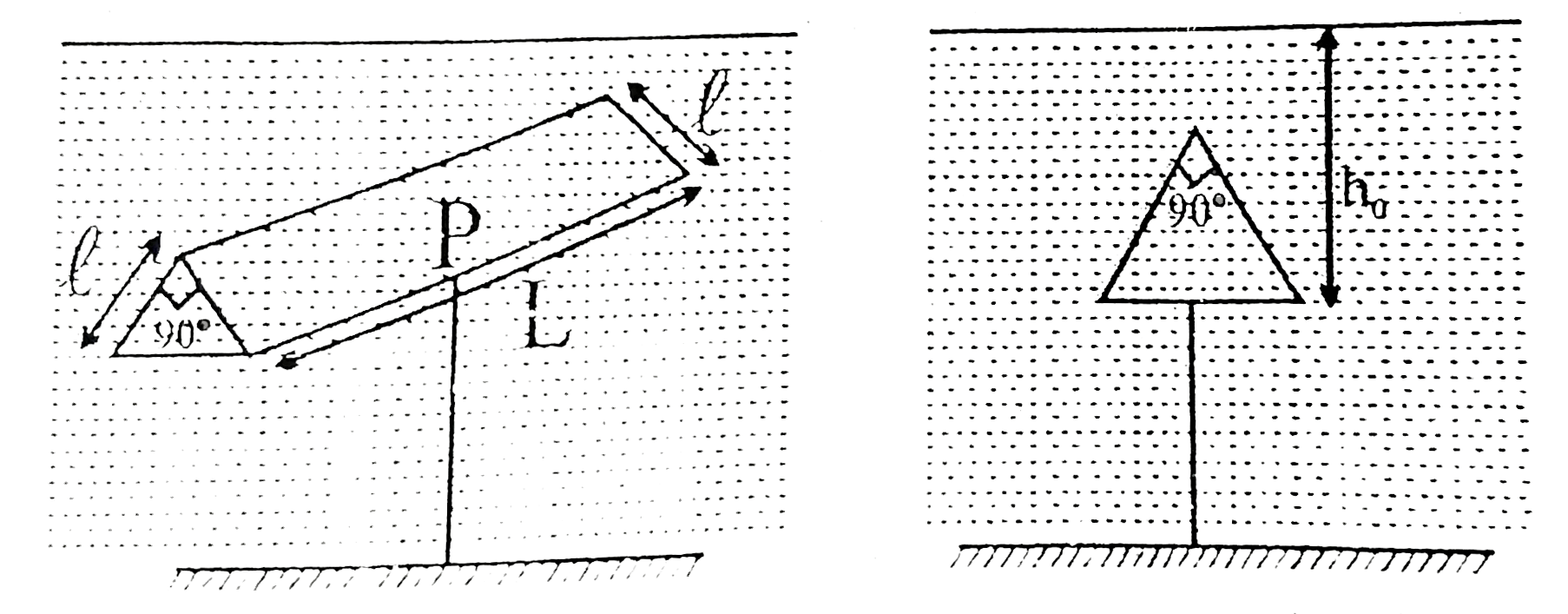Now string is cut and styrofoam is allowed to come to surface. A point mass is to be placed symmetrically on the upper surface of styrofoam such that it is in equilibrium with its base in horizontal plane. In equilibrium position styrofoam has half of its slant length submerged. Surface tension of water is T. contact angle is 0^(@). Determine mass m to achieve equilibrium.

A $\frac{ρ_{f} g L l^{2}}{2} + \frac{3}{4} ρ_{w} g L l^{2} - 2 T [L + l]$
B $\frac{- ρ_{f} g L l^{2}}{2} + \frac{3}{8} ρ_{w} g L l^{2} - \sqrt{2} T [L + l]$
C $\frac{- ρ_{f} g L l^{2}}{2} + \frac{3}{8} ρ_{w} g L l^{2} - \frac{T [L + l)}{\sqrt{2}}$
DNone of these
Question 3 - Select One
The pressure at depth h below the surface of a liquid of density $ρ$ open to the atmosphere is
Agreater than the atmospheric pressure by $ρ g h$
Bless than the atmospheric pressure by $ρ g h$
Cequal to the atmospheric pressure
Dincreases exponentially with depth

BANSAL-MASTER PRACTICE PROBLEM-Comphrehension

Home
Ask Doubt
Profile

The correct Answer is:D

Average pressure on slant surface Pavg=(P0+ρwgh0)+(P0+ρwgh0−1√2)2=(P0+ρwgh0−l2√2) Force on any one of the slant face =(P0+ρwg(h0−l2√2)Ll

Topper's Solved these Questions

Similar Questions

Knowledge Check

The pressure at depth h below the surface of a liquid of density ρ open to the atmosphere is

BANSAL-MASTER PRACTICE PROBLEM-Comphrehension

Average pressure on slant surface
$P_{a v g} = \frac{(P_{0} + ρ_{w} g h_{0}) + (P_{0} + ρ_{w} g h_{0} - \frac{1}{\sqrt{2}})}{2} = (P_{0} + ρ_{w} g h_{0} - \frac{l}{2 \sqrt{2}})$
Force on any one of the slant face
$= (P_{0} + ρ_{w} g (h_{0} - \frac{l}{2 \sqrt{2}}) L l$

The pressure at depth h below the surface of a liquid of density $ρ$ open to the atmosphere is