2 loudspeakers are emitting sound waves of wavelength lambda with an initial phase difference of π2 at what minimum distance from O on line AB will one hear a maximum

25λ
100λ√15
25λ3
50λ
The correct Answer is:C
Δϕ=2nπ
⇒π2+2πλdsinθ=2nπ
2πλdsinθ=(2n−12)π
sinθ=(2n−12)λ2d=12×λ2×3λ=112
⇒y√(100λ)2=112
144y2=(100λ)2
y≈100λ12=25λ3
Topper's Solved these Questions
- MASTER PRACTICE PROBLEMBook:BANSALChapter:MASTER PRACTICE PROBLEMExercise:Comphrehension
Explore 134 Videos
- MASTER PRACTICE PROBLEMBook:BANSALChapter:MASTER PRACTICE PROBLEMExercise:Reasoning
Explore 75 Videos
- FLUID MECHANICSBook:BANSALChapter:FLUID MECHANICSExercise:PYQS AIEEE
Explore 10 Videos
- SEMICONDUCTORSBook:BANSALChapter:SEMICONDUCTORSExercise:CBSE Question
Explore 32 Videos
Similar Questions
What is the minimum distance between two points in a wave having a phase difference 2π ?
View SolutionTwo point sources of sound are kept at a separation of 10 cm. They vibrate in phase to produce waves of wavelength 5.0 cm. What would be the phase difference between the two waves arriving at a point 20 cm from one source (a) on the line joining the sources and (b) on the perpendicular bisector of the line joining the sources ?
View Solution
Knowledge Check
A sine wave of wavelength λ is travelling in a medium. The minimum distance between the two particles always having same speed is.
AλBλ2Cλ3Dλ4Two wavefronts separated by a distance of λ/2 have a phase difference of -
A90∘B180∘C270∘D360∘Two sound sources emitting sound each of wavelength λ are fixed at a given distance apart. A listener moves with a velocity u along the line joining the two suorces. The number of beats heard by him per second is
A2u/λBu/λCu3λD2λu
There is a destructive interference between the two waves of wavelength λ coming from two different paths at a point. To get maximum sound or constructive interference at that point, the path of one wave is to be increased by
View SolutionTwo coherent narrow slits emitting sound of wavelength λ in the same phase are placed parallet to each other at a small separation of 2λ . The sound is delected by maving a delector on the screen at a distance D(>>λ) from the slit S1 as shows in figure. Find the distance y such that the intensity at P is equal to intensity at O .
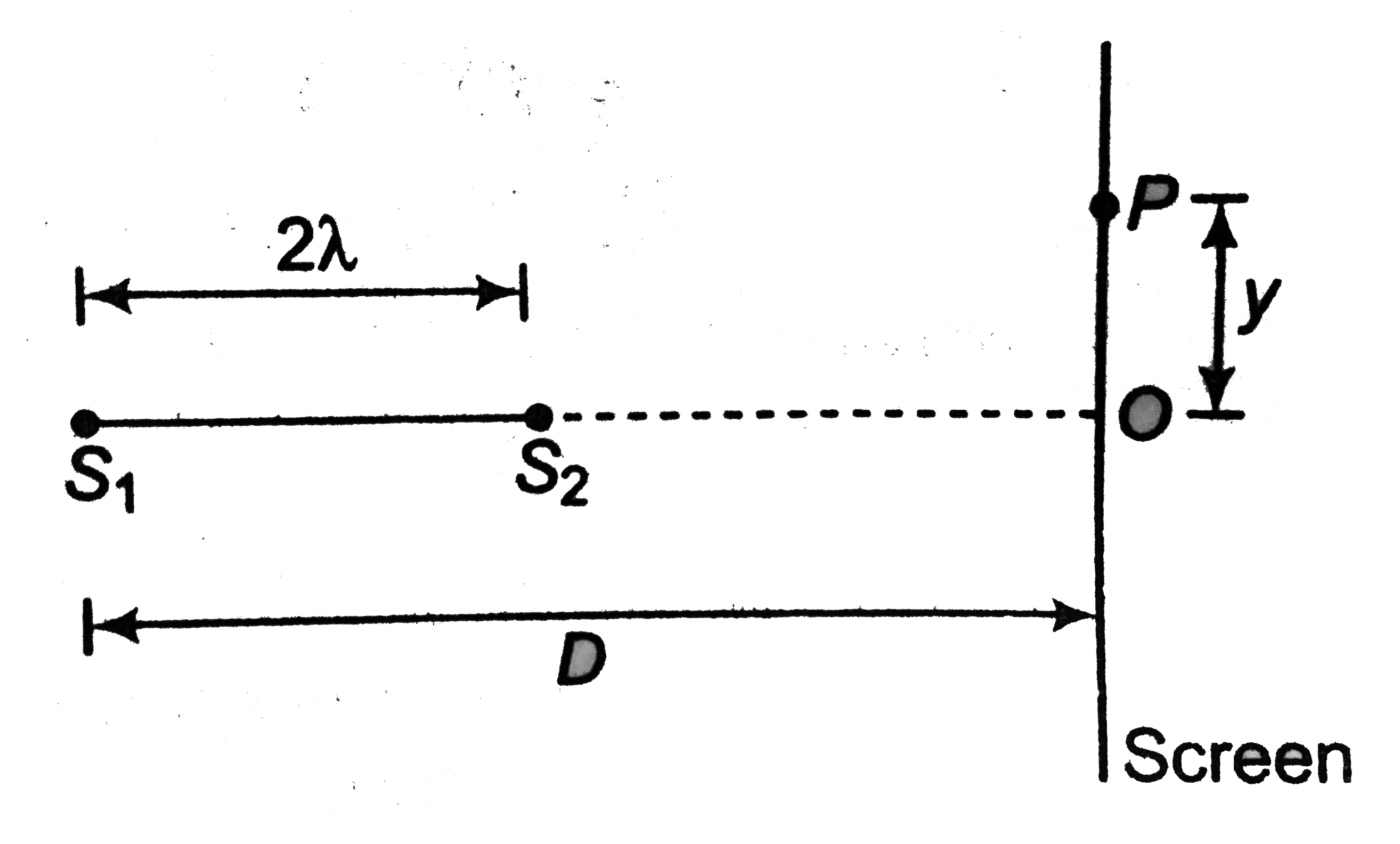
View SolutionTwo sound sources emitting sound each of wavelength λ are fixed at a given distance apart. A listener moves with a velocity u along the line joining the two suorces. The number of beats heard by him per second is
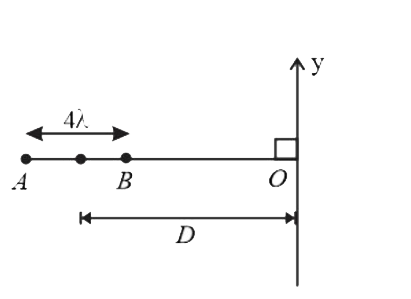
View SolutionTwo coherent sound sources A and B produce a sound of wavelength λ. If at t=0 a detector starts moving with constant velocity v0 from point O along y - direction, then the minimum time, after which it detects the sound of maximum intensity, is (D>>λ)
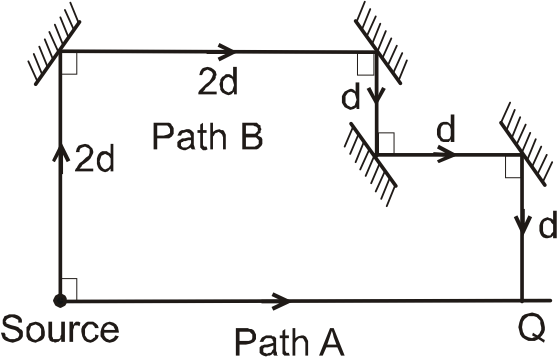
View SolutionA sound source emits two sinusoidal sound waves, both of wavelength λ, along paths A and B as shown in figure.The sound travelling along path B is reflected from five surfaces as shown and then merges at point Q, producing minimum intensity at that point.The minimum value of d in terms of λ is :
View Solution