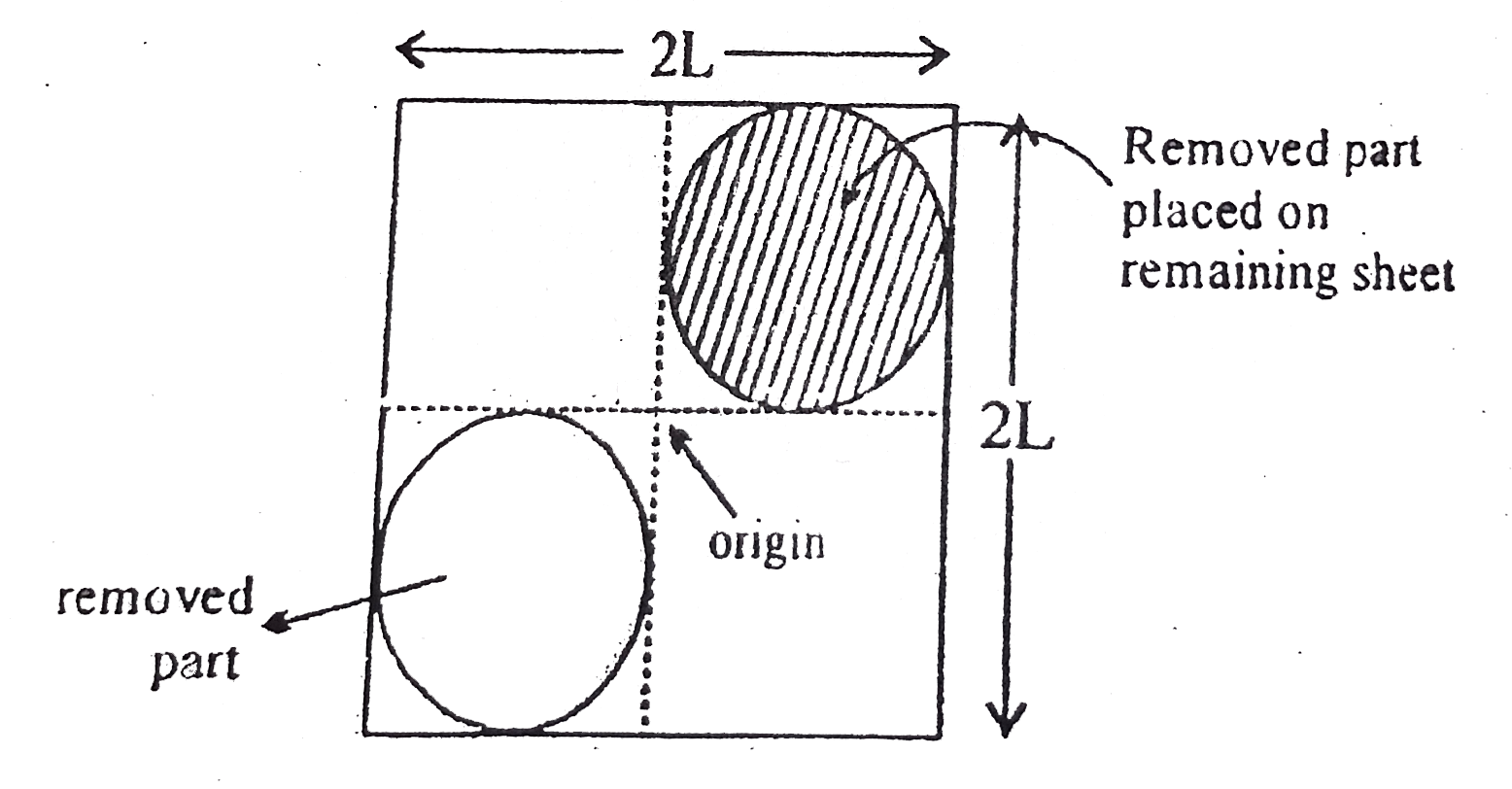
Figure shows a square lamina with a disc of radius L2 removed from it which is now placed symmetrically over upper right quarter what is location of centre of mass of system relative to origin shown in figure
πL12(ˆi+ˆj)
πL8(ˆi+ˆj)
πL4(ˆi+ˆj)
πL16(ˆi+ˆj)
The correct Answer is:D

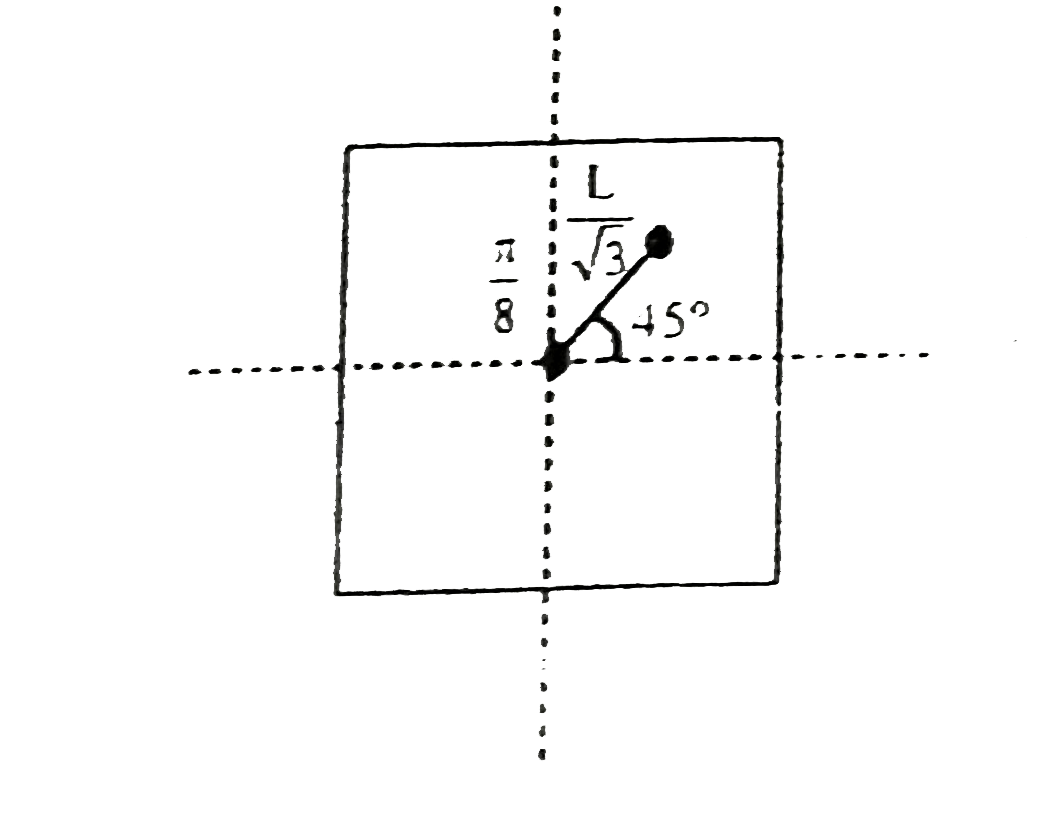
XCr=(πm16)L√2−πm16(−L√2)+mm
⇒π8(L√2)omorig∈
→r=π8(L√2)(cos45ˆi+son45ˆj)=πL8(ˆi+ˆj)
=+mlm=+σπL24σL2L=π4L
Topper's Solved these Questions
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Comphrehension
134 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Reasoning
75 Videos
- FLUID MECHANICSBOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE
10 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
The given figure shows a square lamina with a disc of radius L2 removed from it which is now placed symmetrically over upper right quarter. The location of the centre of mass of the system relative to origin shown in the figure is πLy(ˆi+ˆj). Find y.
'(##CEN_KSR_PHY_JEE_C08_E01_018_Q11##)'
View SolutionA disc of radius a2 is cut out from a uniform disc of radius a as shown in figure . Find the X Coordinate of centre of mass of remaining portion
View Solution
Knowledge Check
Figure shows a square plate of uniform thickness and side length √2m. One fourth of the plate is removed as indicated. The distance of centre of mass of the remaining portion from the centre of the original square plate is
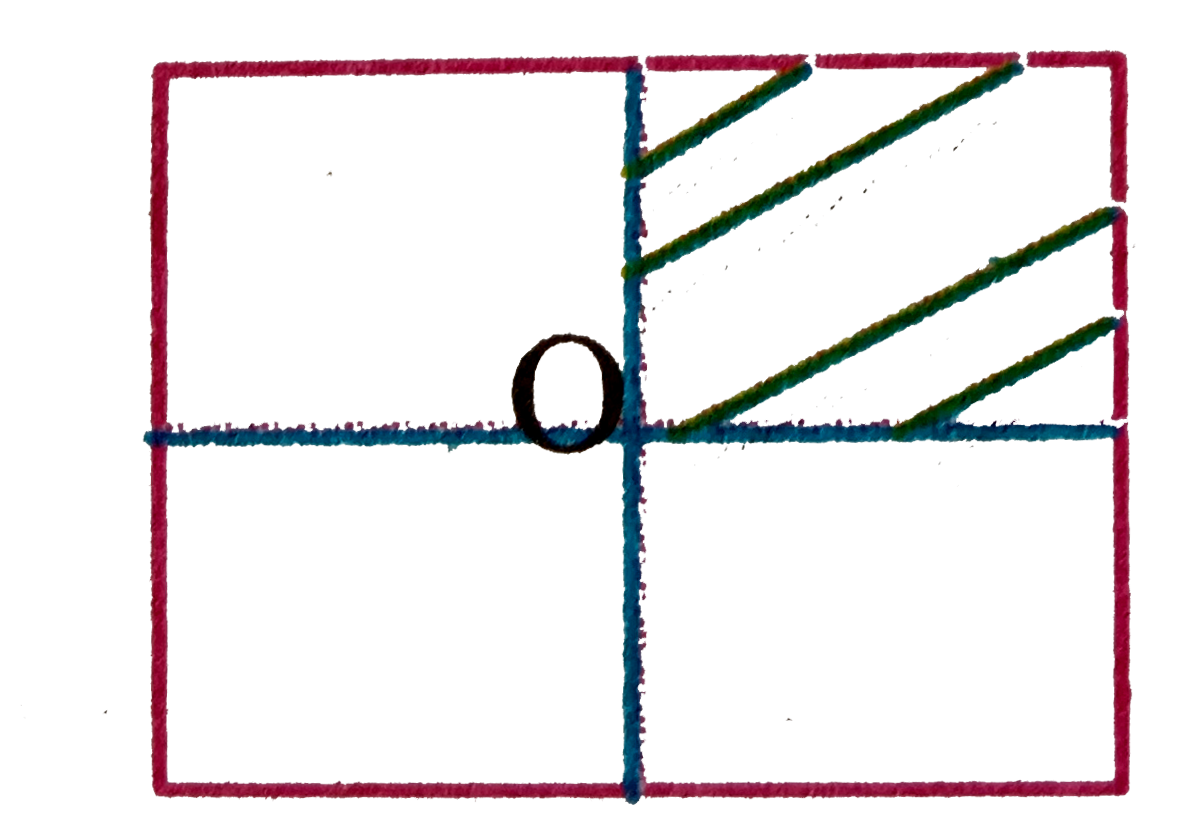 A1/3mB1/2mC1/6mD1/8m
A1/3mB1/2mC1/6mD1/8mThere is a thin uniform disc of radius R and mass per unit area σ in which a hole of radius R/2 has been cut out as shown in the figure. Inside the hole, a square plate of same mass per unit area σ is inserted so that its corners touch the periphery of the hole. The distance of the centre of mass of the system from the origin is
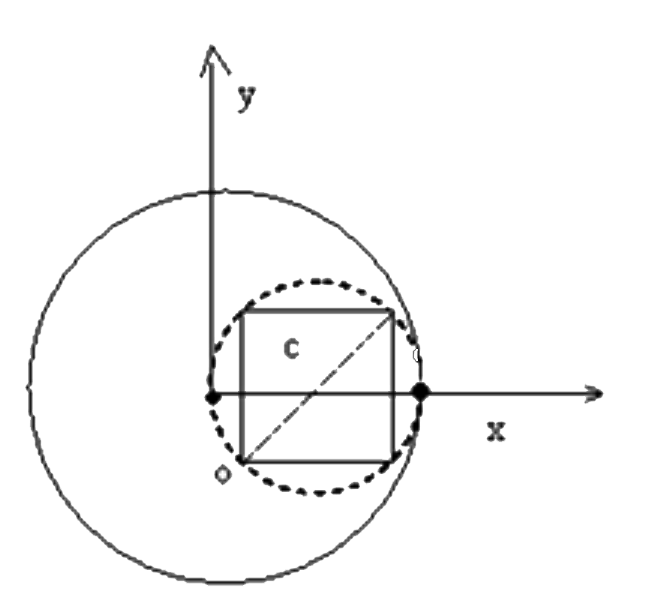 AR(2−π)2(3π+2)BR(1−π)2(2π+1)C2Rπ2(3π+2)D3Rπ2(2π+1)
AR(2−π)2(3π+2)BR(1−π)2(2π+1)C2Rπ2(3π+2)D3Rπ2(2π+1)
The given figure shows a disc of radius R=20cm with a portion of it removed symmetrically. The removed part is a disc, of radius R2. The removed part is now placed in contact with the larger disc as shown in the figure. The disc has uniform mass distribution. With respect to origin O at centre of larger disc, find x -coordinate (in cm ) of the centre of mass of system.
'(##CEN_KSR_PHY_JEE_C08_E01_011_Q07##)'
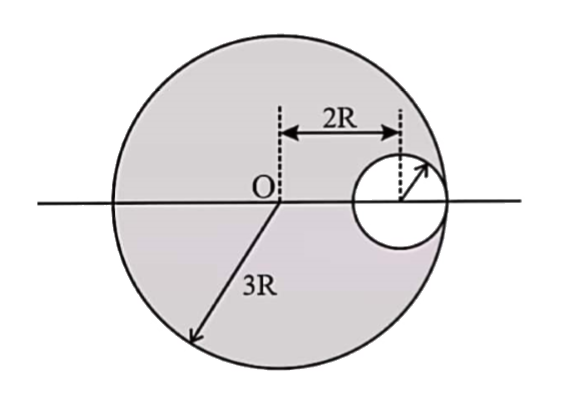
View SolutionThe figure shows a disc of radius 3R from which a circular hole of radius R is cut as shown in the figure. The distance of the centre of mass of the remaining object from the point O is
View SolutionThe figure shows a square metal plate of side l from which a square plate of side a has been cut as shown in the figure. Find the ratio (a/l) so that the centre of mass of the remaining L-shaped plate coincides with the point A
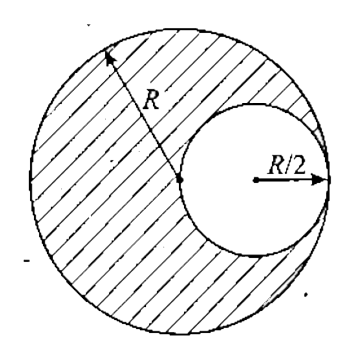
View SolutionFigure-4.11 shows a circular a disc of radius R from which a small disc is cut such that the periphery of the small disc touch the large disc and whose radius is R/2. Find the centre of mass of the remaining part of the disc.
View SolutionFigure shows a disc of radius R=20cm with a portion of it removed symmetrically. The removed part is a disc of radius R/2. The removed part is now placed in contact with the larger disc. Centres of all the three discs lie on x-axis. Disc has uniform mass distribution. With respect to origin at centre of larger disc find x-coordinate of center of mass of system (in cm).
View SolutionFigure shows a uniform disc of radius R, from which a hole of radius R2 has been cut out from left of the centre and is placed on the right of the centre of the disc. Find the CM of the resulting disc.

View Solution