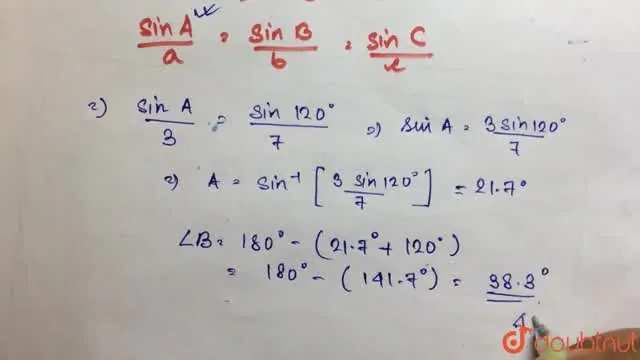Sovle ΔABC if a=3,b=5,c=7.
Video Solution
Text Solution

By the law of cosines m∠A:32=52+72−2(5)(7)cosA, so cosA=32−52−72−2(5)(7)≈0.9285
and m∠A≈21.79
by the law of sines, sinB5=21.793, so m∠≈38.21∘.
Finally, m∠C≈180∘−21.79∘−38.21=120∘.
|
Updated on:21/07/2023
Related Playlists
- BOOK - ENGLISH SATCHAPTER - TRIANGLE TRIGONOMETRY EXERCISE - EXERCISES13videos
TRIANGLE TRIGONOMETRY
- BOOK - ENGLISH SATCHAPTER - TRANSFORMATION AND SYMMETRYEXERCISE - EXERCISES4videos
TRANSFORMATION AND SYMMETRY
- BOOK - ENGLISH SATCHAPTER - TRIGONOMETRIC FUNCTIONSEXERCISE - MCQs (Exercise)30videos
TRIGONOMETRIC FUNCTIONS
Similar Questions
Solve ΔABC if a=7,b=12,m∠C=62∘.
View SolutionIn a ΔABC, if a = 4, b = 5 , c = 6 then angle C is equal to
View SolutionSovle ΔABC if m∠A=90∘,a=7,b=5.
View SolutionSovle ΔABC if m∠A=125∘,a=10,b=8.
View SolutionIn a ΔABC, if a = 3, b = 5 and c = 7, find cos c
View SolutionIf in ΔABC,a=3,b=5,c=7 find the greatest angle.
View SolutionIn ΔABC,
if a = 2, b = 3, c = 4, prove that cosA=78.View SolutionSolve ΔABC if m∠A=50∘,a=7,b=5.
View SolutionSolve ΔABC if m∠A=50∘,a=3,b=5.
View SolutionIn ΔABC,a=3,b=5,c=6, find the value of : sin(A2)
View Solution