Given the following data which, can form two triangles?
I. ∠C=30∘,c=8,b=12
II. ∠B=45∘,a=12√2,b=12√2
III. ∠C=60∘,b=12,c=5√3
only I
only II
only III
only I and II
The correct Answer is:A
To determine which sets of data can form two triangles, we will follow the conditions for triangle formation based on the given angle and sides. Let's analyze each case step by step.
Given Data:
1. I. ∠C=30∘,c=8,b=12
2. II. ∠B=45∘,a=12√2,b=12√2
3. III. ∠C=60∘,b=12,c=5√3
Step 1: Analyze the first set of data (I)
- Given: ∠C=30∘,c=8,b=12
- Identify the largest side: b=12
- Calculate the altitude from angle C:
Altitude=b⋅sin(∠C)=12⋅sin(30∘)=12⋅12=6
- Compare the smallest side c=8 with the altitude 6 and the largest side 12:
- Since 6<8<12, two triangles can be formed.
Step 2: Analyze the second set of data (II)
- Given: ∠B=45∘,a=12√2,b=12√2
- Identify the largest side: a=b=12√2
- Calculate the altitude from angle B:
Altitude=a⋅sin(∠B)=12√2⋅sin(45∘)=12√2⋅1√2=12
- Compare the smallest side (which is equal to the other side) with the altitude:
- Since 12=12, only one triangle can be formed.
Step 3: Analyze the third set of data (III)
- Given: ∠C=60∘,b=12,c=5√3
- Identify the largest side: b=12
- Calculate the altitude from angle C:
Altitude=b⋅sin(∠C)=12⋅sin(60∘)=12⋅√32=6√3
- Compare the smallest side c=5√3 with the altitude 6√3 and the largest side 12:
- Since 5√3<6√3<12, no triangle can be formed.
Conclusion:
- Only the first set of data can form two triangles.
- The second set can form one triangle.
- The third set cannot form any triangles.
Final Answer:
Only the data from I can form two triangles.
1. I. ∠C=30∘,c=8,b=12
2. II. ∠B=45∘,a=12√2,b=12√2
3. III. ∠C=60∘,b=12,c=5√3
- Given: ∠C=30∘,c=8,b=12
- Identify the largest side: b=12
- Calculate the altitude from angle C:
Altitude=b⋅sin(∠C)=12⋅sin(30∘)=12⋅12=6
- Compare the smallest side c=8 with the altitude 6 and the largest side 12:
- Since 6<8<12, two triangles can be formed.
- Given: ∠B=45∘,a=12√2,b=12√2
- Identify the largest side: a=b=12√2
- Calculate the altitude from angle B:
Altitude=a⋅sin(∠B)=12√2⋅sin(45∘)=12√2⋅1√2=12
- Compare the smallest side (which is equal to the other side) with the altitude:
- Since 12=12, only one triangle can be formed.
- Given: ∠C=60∘,b=12,c=5√3
- Identify the largest side: b=12
- Calculate the altitude from angle C:
Altitude=b⋅sin(∠C)=12⋅sin(60∘)=12⋅√32=6√3
- Compare the smallest side c=5√3 with the altitude 6√3 and the largest side 12:
- Since 5√3<6√3<12, no triangle can be formed.
- Only the first set of data can form two triangles.
- The second set can form one triangle.
- The third set cannot form any triangles.
Only the data from I can form two triangles.
Topper's Solved these Questions
- BOOK - ENGLISH SATCHAPTER - TRIANGLE TRIGONOMETRY EXERCISE - EXERCISES13videos
TRIANGLE TRIGONOMETRY
- BOOK - ENGLISH SATCHAPTER - TRANSFORMATION AND SYMMETRYEXERCISE - EXERCISES4videos
TRANSFORMATION AND SYMMETRY
- BOOK - ENGLISH SATCHAPTER - TRIGONOMETRIC FUNCTIONSEXERCISE - MCQs (Exercise)30videos
TRIGONOMETRIC FUNCTIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
10√12÷2√3
A5B10C40D20 - Question 2 - Select One
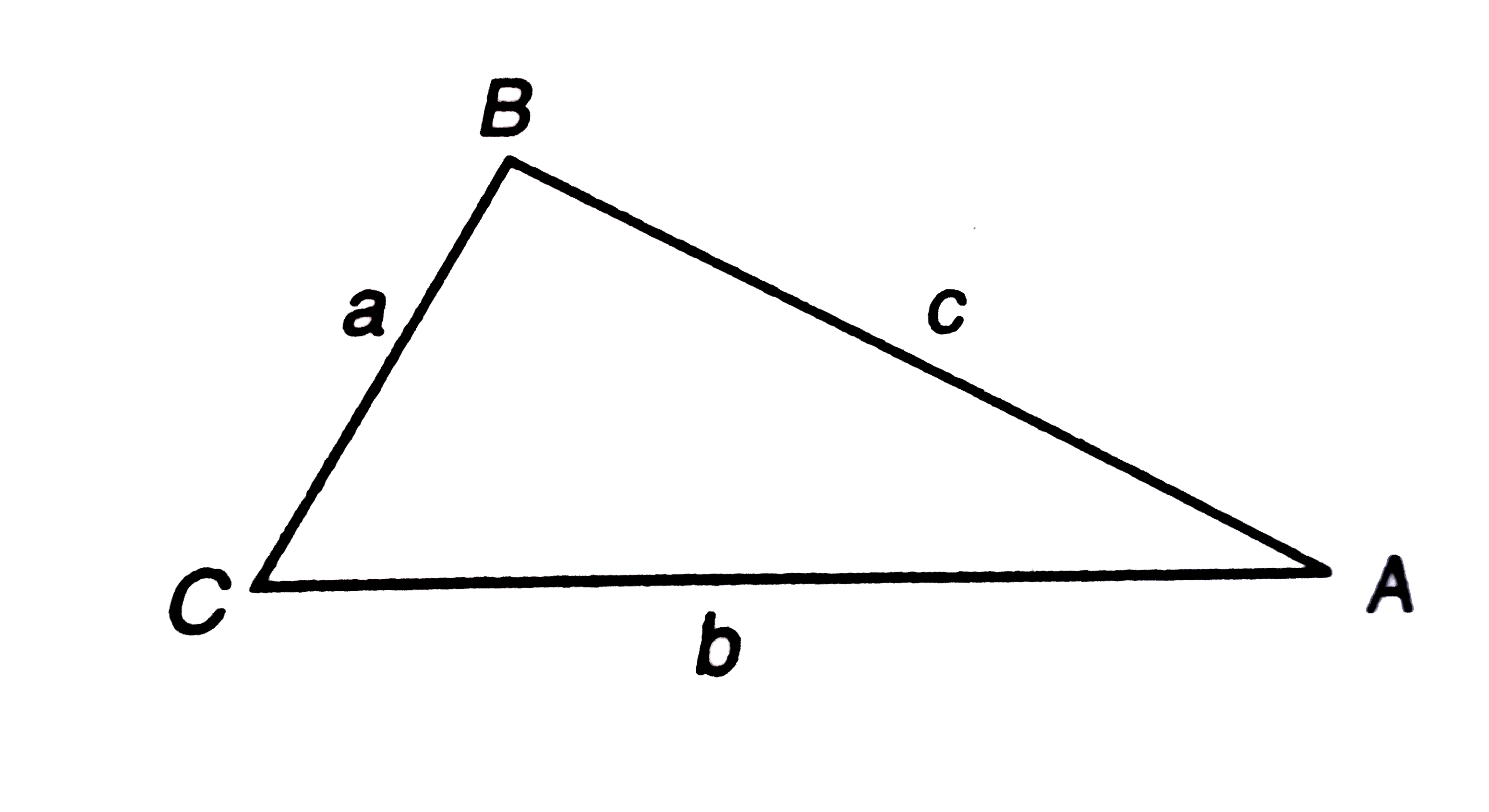
In △ABC above, a=2x, b=3x+2, c=√12, and ∠C=60∘. Find x.A0.50B0.64C0.77D1.64
If in △ABC,a=2√3,b=3√2 and ∠B=60∘, then find the measure of ∠C.
View SolutionA ΔABC can be constructed in which ∠B=60∘,∠C=45∘andAB+BC+CA=12cm.
View SolutionIf a=1+√3,b=2 and c=√2, then the measure of ∠B is
View SolutionIn a triangle ABC,ab=2+√3 and ∠C=60∘ then angle A and B is
View SolutionIf a and b are two real number lying between 0 and 1 such that z1=a+i,z2=1+biandz3=0 form an equilateral triangle , then (A) a=2+√3 (B) b=4−√3 (C) a=b=2−√3 (D) a=2,b=√3
View SolutionIn any ΔABC, prove that
(i) tanB−C2=b−cb+ccotA2.
(ii) If ∠B=90∘, prove that tan(A2)=√(b−cb+c).
(iii) If ∠C=90∘, prove that tan(A−B2)tan(A+B2)=a−ba+b.View Solution

