If angular velocity of a disc depends an angle rotated θ as ω=θ2+2θ, then its angular acceleration α at θ=1 rad is :
8rad/s2
10rad/s2
12rad/s2
None of these
The correct Answer is:C
To find the angular acceleration α at θ=1 rad, we start with the given angular velocity equation:
ω=θ2+2θ
Step 1: Find dωdθ
To find the angular acceleration α, we first need to calculate the derivative of ω with respect to θ:
dωdθ=ddθ(θ2+2θ)
Using basic differentiation rules:
dωdθ=2θ+2
Step 2: Substitute θ=1
Now we substitute θ=1 rad into the derivative we just calculated:
dωdθ∣∣∣θ=1=2(1)+2=2+2=4
Step 3: Calculate ω at θ=1
Next, we calculate ω at θ=1:
ω∣∣∣θ=1=12+2(1)=1+2=3
Step 4: Calculate Angular Acceleration α
Now we can find the angular acceleration α using the formula:
α=ω⋅dωdθ
Substituting the values we found:
α=3⋅4=12rad/s2
Final Answer
Thus, the angular acceleration α at θ=1 rad is:
α=12rad/s2
---
Topper's Solved these Questions
- KINEMATICSBook:ALLENChapter:KINEMATICSExercise:EXERCISE-02
Explore 57 Videos
- KINEMATICSBook:ALLENChapter:KINEMATICSExercise:EXERCISE-03
Explore 6 Videos
- KINEMATICSBook:ALLENChapter:KINEMATICSExercise:Integer Type Question
Explore 3 Videos
- ERROR AND MEASUREMENTBook:ALLENChapter:ERROR AND MEASUREMENTExercise:Part-2(Exercise-2)(B)
Explore 22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Book:ALLENChapter:KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Exercise:BEGINNER S BOX-7
Explore 8 Videos
Similar Questions
A uniiform disc of radius r spins with angular velocity ω and angular acceleration α. If the centre of mass of the disc has linear acceleration a, find the magnitude and direction of aceeleration of the point 1,2, and 3.
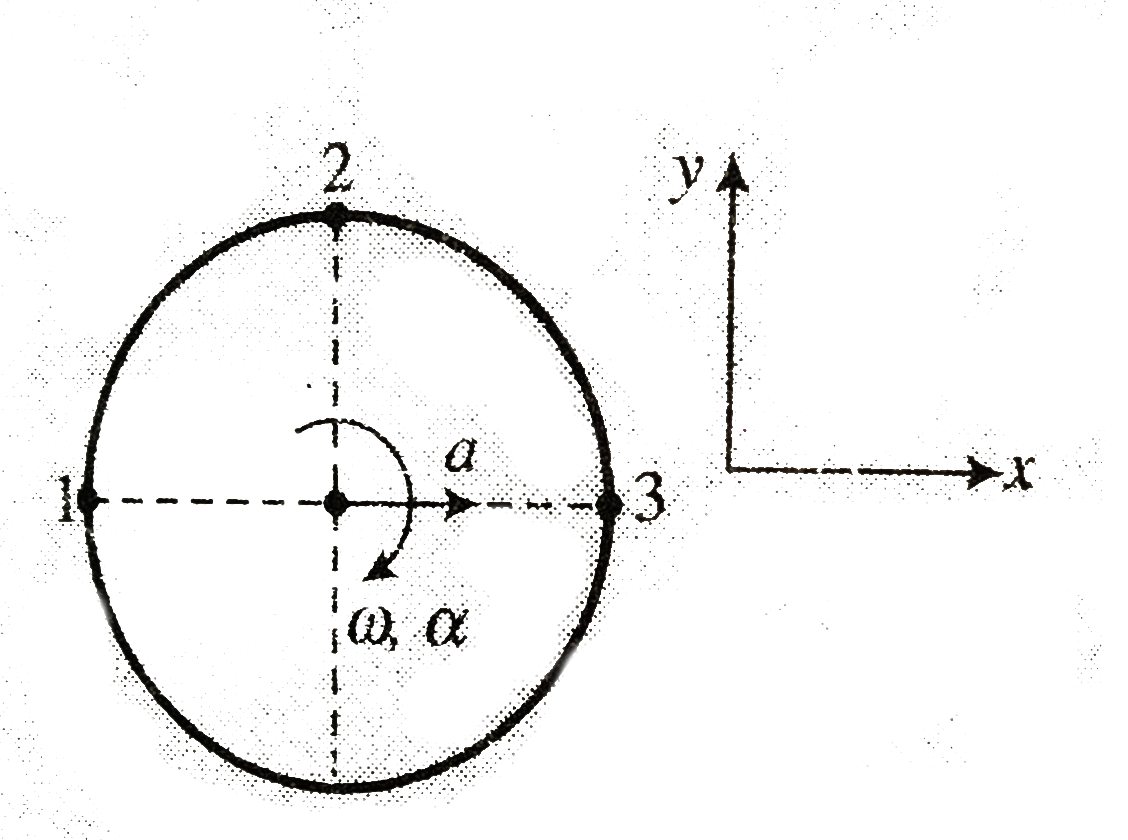
View SolutionThe angular position of a point on a rotating wheel is given by θ=2.0+4.0t2+2.0t3, where θ is in radians and t is in seconds. At t = 0, what are (a) the point's angular position and (b) its angular velocity? ( c) What is its angular velocity at t = 3.0 s? (d) Calculate its angular acceleration at t = 4.0 s. (e) Is its angular acceleration constant?
View Solution
Knowledge Check
The angle turned by a body undergoing circular motion depends on time as θ=θ0+θ1t+θ2t2. Then the angular acceleration of the body is
Aθ1Bθ2C2θ1D2θ2The angle turned by a body undergoing circular motion depends on time as θ=θ0+θ1t+θ2r2. Then the angular acceleration of the body is
Aθ1Bθ2C2θ1D2θ2A stationary horizontal disc is free to rotate about its axis. When a torque is applied on it, its kinetic energy as a function of θ, where θ is the angle by which it has rotated, is given as kθ2 If its moment of inertia is I then the angular acceleration of the disc is
Ak2IθBkIθCk4IθD2kIθ
The angular displacement of a particle is given by θ=t3+t2+t+1 then,the angular acceleration of the particle at t=2 sec is …….rad s^(-2)
View SolutionThe angular displacement of a particle is given by θ=t3+2t+1, where t is time in seconds. Its angular acceleration at t=2s is
View SolutionThe angular displacement of a flywheel varies with time as θ=at+bt2−ct3. Then the angular acceleration is given by
View SolutionAngular displacement (θ) of a flywheel varies with time as θ=at+bt2+ct3 then angular acceleration is given by
View SolutionAngular displacement (θ) of a flywheel varies with time as θ=2t+3t2 radian. The angular acceleration at t=2s is given by
View Solution