The equation of one dimensional motion of the particle is described in column I. At t=0, particle is at origin and at rest. Match the column I with the statement in Column II.
Column IColumn II(A)x=(3t2+2)m(p)Velocity of particle at t=1s is 8m/s(B)v=8tm/s(q)Particle moves with uniform acceleration(C)a=16t(r)Particle moves with variable acceleration(D)v=6t−3t2(s)Particle will change its direction some time
The correct Answer is:(A)-q, (B)-p, q (C)-p, r, (D)-r, s
[A]X=3t2+2⇒V=dxdt=6t⇒a=d2xdt2
[B]V=8t⇒a=dvdt=8
[D] For changing the direction 6t−3t2=0
⇒t=0,2 sec
Topper's Solved these Questions
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Assertion-Reason
20 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Comprehension#1
3 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - EXERCISE-02
57 Videos
- ERROR AND MEASUREMENTBOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLENCHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
Similar Questions
A sine wave y=sin (2πx−2πt+π/3) is propagating in the medium. Match the description of the motion of particles of the medium with entries in columnI
Column-IColumn-II(A)x=13m, t=13sec(P)Velocity is in positive y direction(B)x=13m, t=1sec(Q)Velocity is in negative y direction(C)x=1m, t=13sec(R)Particle is stationary(D)x=1m, t=1sec(S)Particle has positive displacement(T)Particle has negative displacement
View SolutionA particle is given an initial velocity of →u=(3ˆi+4ˆj)m/s. Acceleration of the particle is →a=(3t2+2tˆj)m/s2. Find the velocity of particle at t=2s.
View Solution
Knowledge Check
The motion of a particle is described as s=2−3t+4t3. What is the acceleration of the particle at the point where its velocity is zero?
AA)0BB)4CC)8 unitDD)12 unitStarting from rest, acceleration of a particle is a=2t(t−1). The velocity of the particle at t=5s is
AAt t=2s, the position of the particle is at x=9mBParticle acceleration at t=2s is 1m/s2CParticle acceleration is 1/2m/s2 through out the motionDParticle will never go in negative direction from it's starting positionThe acceleration of a moving particle whose space time equation is given by s=3t2+2t−5, is
A6B5C0D1
A particle starts from rest at t=0 and moves along a straight line with a variable acceleration given by a (t)=(3t+1)ms−2 ,where t],[ is in seconds.The velocity of the particle at t=4s is
View SolutionThe acceleration a of a particle in ms2 is give by a=18t2+36t+120. If the particle starts from rest, then velocity at end of 1 s is
View SolutionA particle is moving along a straight line. Its displacement-time graph is shown
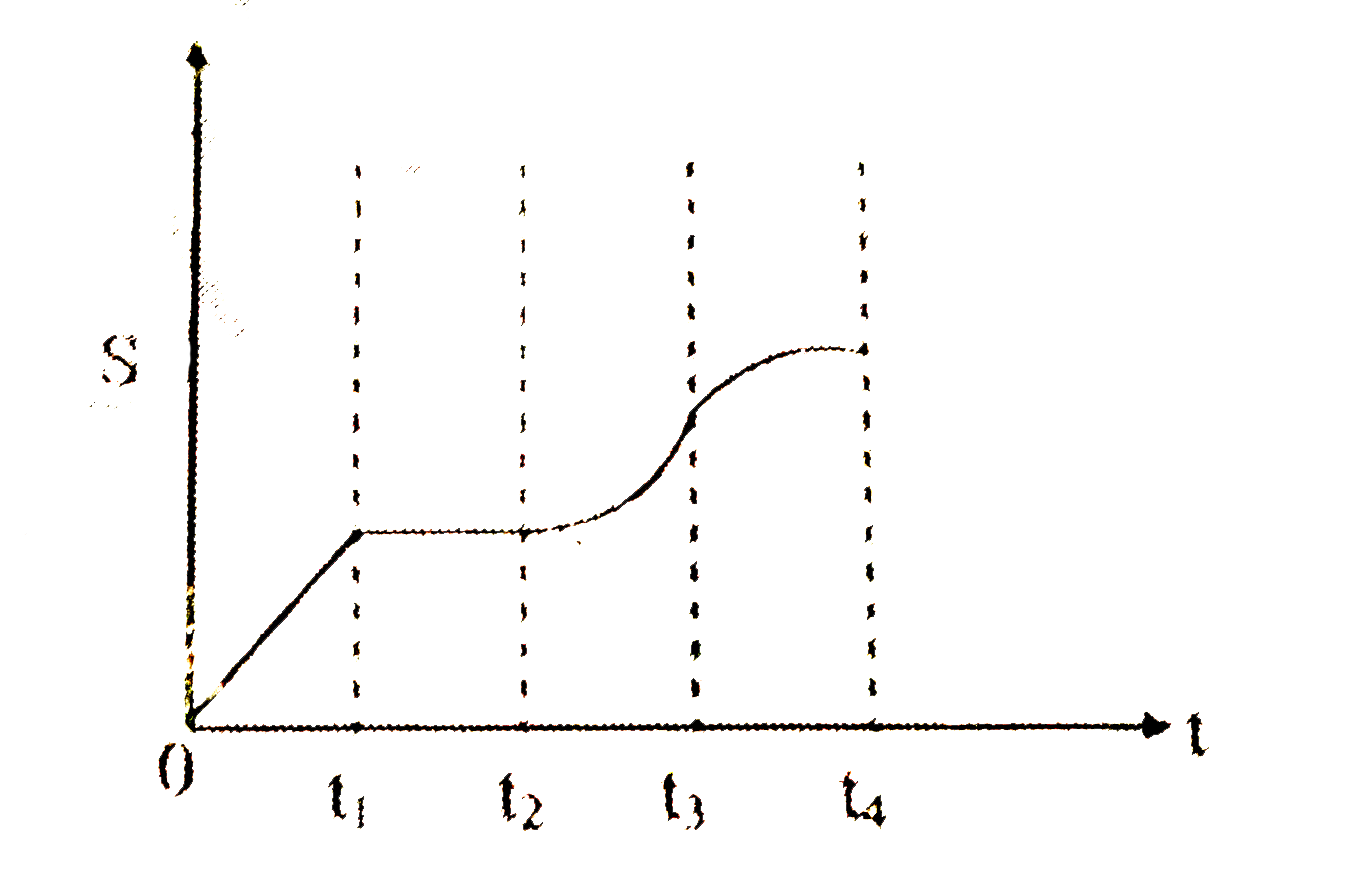
Column-IColumn-II(A)Work done on particle from 0 to t1(P)Positive(B)Work done on particle from t1 to t2(Q)Negative(C)Work done on particle from t2 to t3(R)zero(D)Work done on particle from t3 to t4(S)Unpredectible
View SolutionIf velocity of a particle is given by v=2t2−2 then find the acceleration of particle at t = 2 s.
View SolutionThe motion of a particle along a straight line is described by the function x=(2t−3)2, where x is in metres and t is in seconds. Find
(a) the position, velocity and acceleration at t=2s.
(b) the velocity of the particle at the origin .
View Solution